Are All Absolute Value Functions Differentiable

Are All Absolute Value Functions Differentiable - Let u be a differentiable real. The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. \mathbb{r} \rightarrow \mathbb{r}$ we wish to. Given a differentiable function $f: Looking at different values of the absolute value function in some plots: Let |x| be the absolute value of x for real x. Note that the tangent line.

Note that the tangent line. Let |x| be the absolute value of x for real x. Let u be a differentiable real. Looking at different values of the absolute value function in some plots: The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. Given a differentiable function $f: \mathbb{r} \rightarrow \mathbb{r}$ we wish to.

Given a differentiable function $f: Note that the tangent line. The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. Let |x| be the absolute value of x for real x. Looking at different values of the absolute value function in some plots: \mathbb{r} \rightarrow \mathbb{r}$ we wish to. Let u be a differentiable real.

Absolute Value Functions Transformations Investigation Light Bulb

Let |x| be the absolute value of x for real x. The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. Looking at different values of the absolute value function in some plots: Note that the tangent line. Let u be a differentiable real.

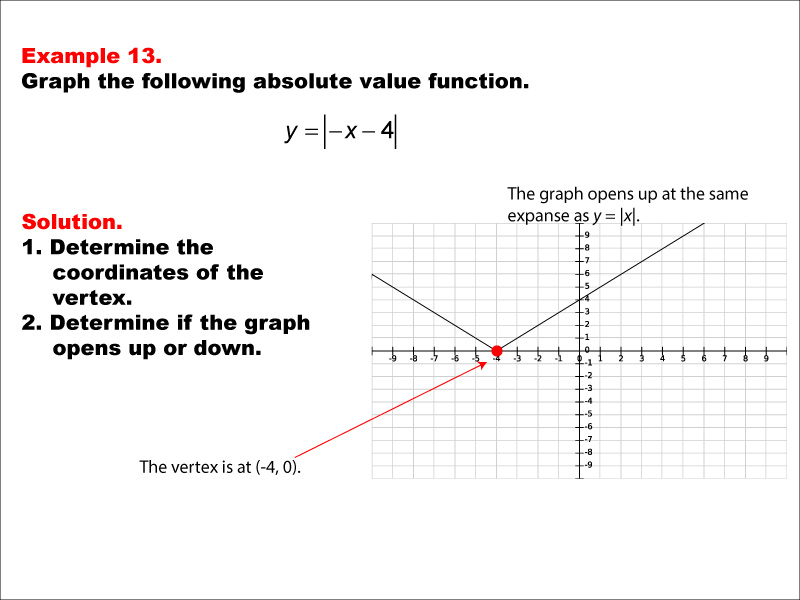
Math Example Absolute Value Functions Example 13 Media4Math

Note that the tangent line. Let |x| be the absolute value of x for real x. Looking at different values of the absolute value function in some plots: The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. Let u be a differentiable real.

PPT 2.7 Absolute Value Functions and Graphs PowerPoint Presentation

The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. Let u be a differentiable real. Note that the tangent line. Let |x| be the absolute value of x for real x. Looking at different values of the absolute value function in some plots:

Absolute Value Graph Of A Function Differentiable Function Real Number

Looking at different values of the absolute value function in some plots: Note that the tangent line. Let u be a differentiable real. Let |x| be the absolute value of x for real x. \mathbb{r} \rightarrow \mathbb{r}$ we wish to.

SOLUTION Absolute value functions Studypool

Given a differentiable function $f: Looking at different values of the absolute value function in some plots: The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. Note that the tangent line. \mathbb{r} \rightarrow \mathbb{r}$ we wish to.

calculus How do I prove if the following functions are differentiable

Looking at different values of the absolute value function in some plots: Given a differentiable function $f: Let u be a differentiable real. The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. Let |x| be the absolute value of x for real x.

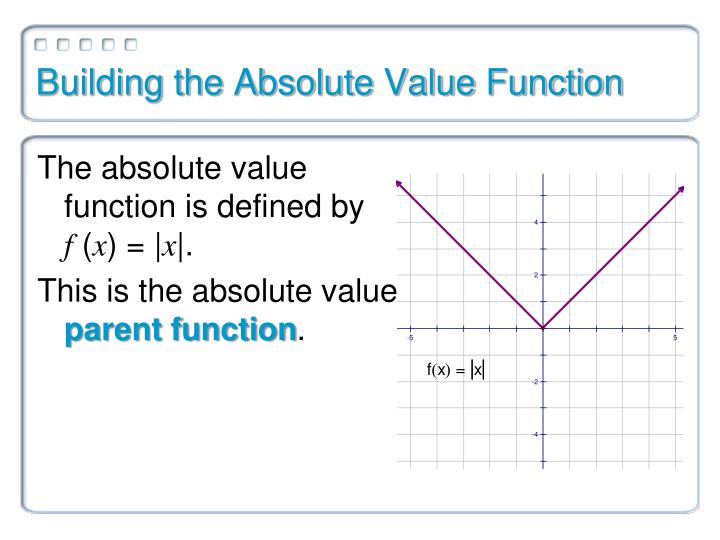
Absolute value functions PPT

Let |x| be the absolute value of x for real x. Note that the tangent line. The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. Let u be a differentiable real. \mathbb{r} \rightarrow \mathbb{r}$ we wish to.

Why is the absolute value function not differentiable at 0 Quizlet

Note that the tangent line. Let |x| be the absolute value of x for real x. \mathbb{r} \rightarrow \mathbb{r}$ we wish to. Let u be a differentiable real. Given a differentiable function $f:

calculus Differentiable approximation of the absolute value function

Given a differentiable function $f: \mathbb{r} \rightarrow \mathbb{r}$ we wish to. Note that the tangent line. Let u be a differentiable real. Looking at different values of the absolute value function in some plots:

[Solved] The differentiable functions ( f ) and ( g )

Given a differentiable function $f: Let |x| be the absolute value of x for real x. Let u be a differentiable real. The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. Looking at different values of the absolute value function in some plots:

Let |X| Be The Absolute Value Of X For Real X.

Looking at different values of the absolute value function in some plots: The function jumps at $x$, (is not continuous) like what happens at a step on a flight of stairs. Let u be a differentiable real. Given a differentiable function $f: