Differential Equations Initial Conditions - For a second order differential equation we have three possible types of boundary conditions: Solve a differential equation analytically by using the dsolve function, with or without initial conditions. In this unit our differential equations will always have initial conditions at t = 0. With these two initial conditions and the general solution to the differential equation, we can find. Pde’s are usually specified through a set of boundary or initial conditions.
Pde’s are usually specified through a set of boundary or initial conditions. Solve a differential equation analytically by using the dsolve function, with or without initial conditions. In this unit our differential equations will always have initial conditions at t = 0. With these two initial conditions and the general solution to the differential equation, we can find. For a second order differential equation we have three possible types of boundary conditions:
In this unit our differential equations will always have initial conditions at t = 0. Solve a differential equation analytically by using the dsolve function, with or without initial conditions. With these two initial conditions and the general solution to the differential equation, we can find. For a second order differential equation we have three possible types of boundary conditions: Pde’s are usually specified through a set of boundary or initial conditions.
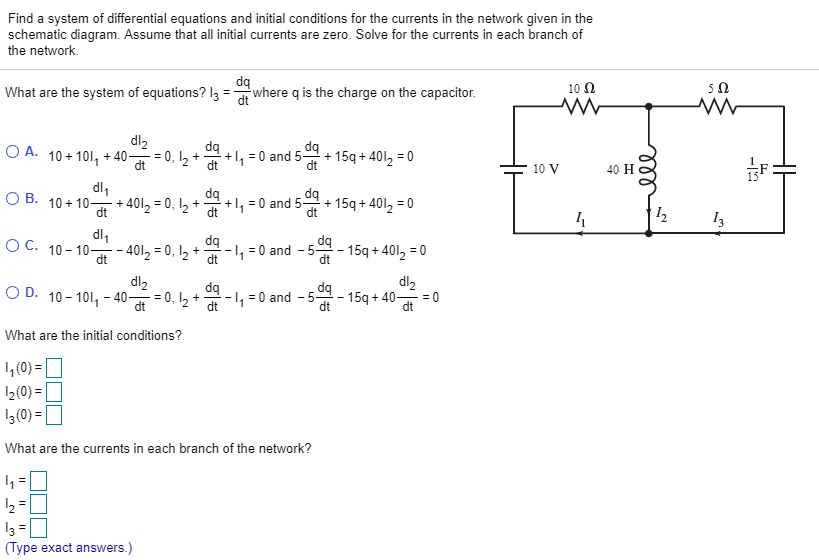
Solved Find a system of differential equations and initial
For a second order differential equation we have three possible types of boundary conditions: With these two initial conditions and the general solution to the differential equation, we can find. Pde’s are usually specified through a set of boundary or initial conditions. In this unit our differential equations will always have initial conditions at t = 0. Solve a differential.
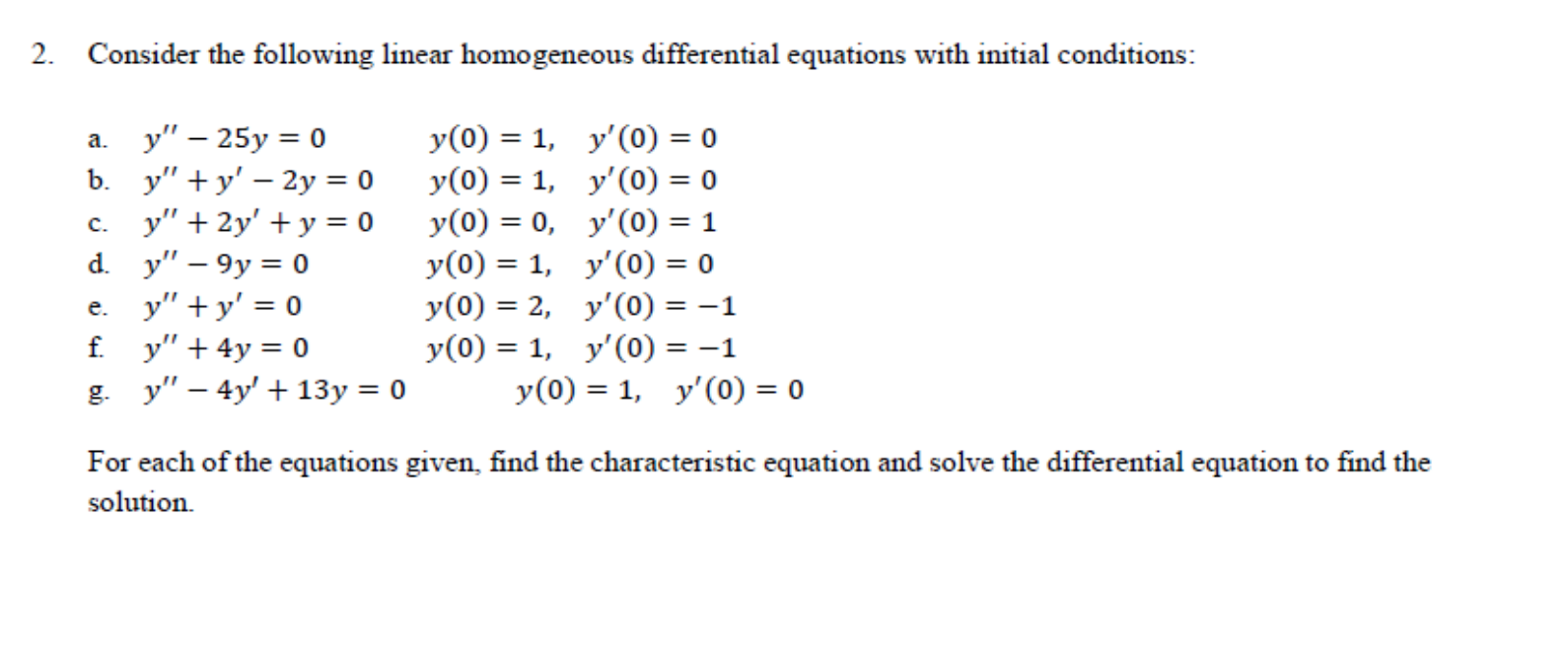
Solved Consider the following linear homogeneous
Pde’s are usually specified through a set of boundary or initial conditions. With these two initial conditions and the general solution to the differential equation, we can find. For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0. Solve a differential.
[Solved] differential equation (1 point) A 10 kilogram object suspended
For a second order differential equation we have three possible types of boundary conditions: With these two initial conditions and the general solution to the differential equation, we can find. Pde’s are usually specified through a set of boundary or initial conditions. In this unit our differential equations will always have initial conditions at t = 0. Solve a differential.
Differential Equations Solver
For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0. Pde’s are usually specified through a set of boundary or initial conditions. Solve a differential equation analytically by using the dsolve function, with or without initial conditions. With these two initial.
Differential Equations
In this unit our differential equations will always have initial conditions at t = 0. Pde’s are usually specified through a set of boundary or initial conditions. For a second order differential equation we have three possible types of boundary conditions: With these two initial conditions and the general solution to the differential equation, we can find. Solve a differential.
Solved Consider the system of differential equations with
Pde’s are usually specified through a set of boundary or initial conditions. Solve a differential equation analytically by using the dsolve function, with or without initial conditions. In this unit our differential equations will always have initial conditions at t = 0. For a second order differential equation we have three possible types of boundary conditions: With these two initial.
(PDF) Numerical Methods for Ordinary Differential Equations Initial
With these two initial conditions and the general solution to the differential equation, we can find. Solve a differential equation analytically by using the dsolve function, with or without initial conditions. For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0..
Solved Solve each one of the following differential
With these two initial conditions and the general solution to the differential equation, we can find. For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0. Solve a differential equation analytically by using the dsolve function, with or without initial conditions..
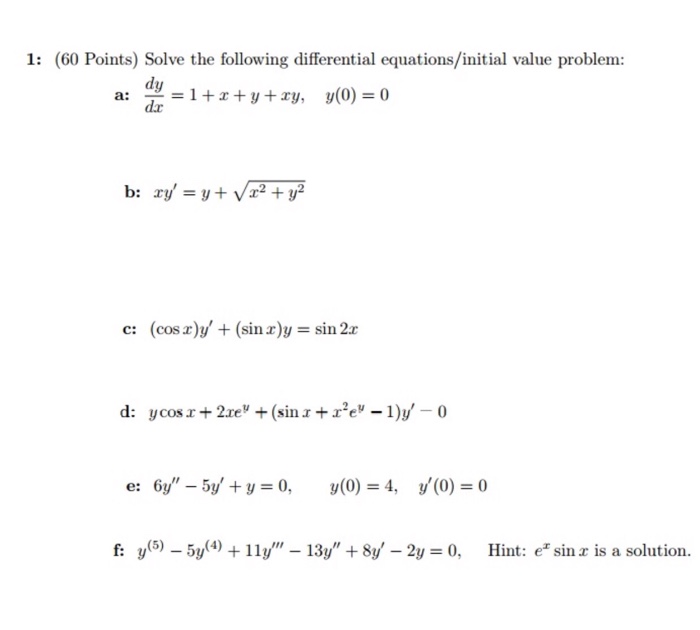
Solved Solve the following differential equations/initial
Solve a differential equation analytically by using the dsolve function, with or without initial conditions. With these two initial conditions and the general solution to the differential equation, we can find. For a second order differential equation we have three possible types of boundary conditions: In this unit our differential equations will always have initial conditions at t = 0..
Exact Differential Equations
In this unit our differential equations will always have initial conditions at t = 0. Pde’s are usually specified through a set of boundary or initial conditions. With these two initial conditions and the general solution to the differential equation, we can find. Solve a differential equation analytically by using the dsolve function, with or without initial conditions. For a.
For A Second Order Differential Equation We Have Three Possible Types Of Boundary Conditions:
Solve a differential equation analytically by using the dsolve function, with or without initial conditions. Pde’s are usually specified through a set of boundary or initial conditions. With these two initial conditions and the general solution to the differential equation, we can find. In this unit our differential equations will always have initial conditions at t = 0.