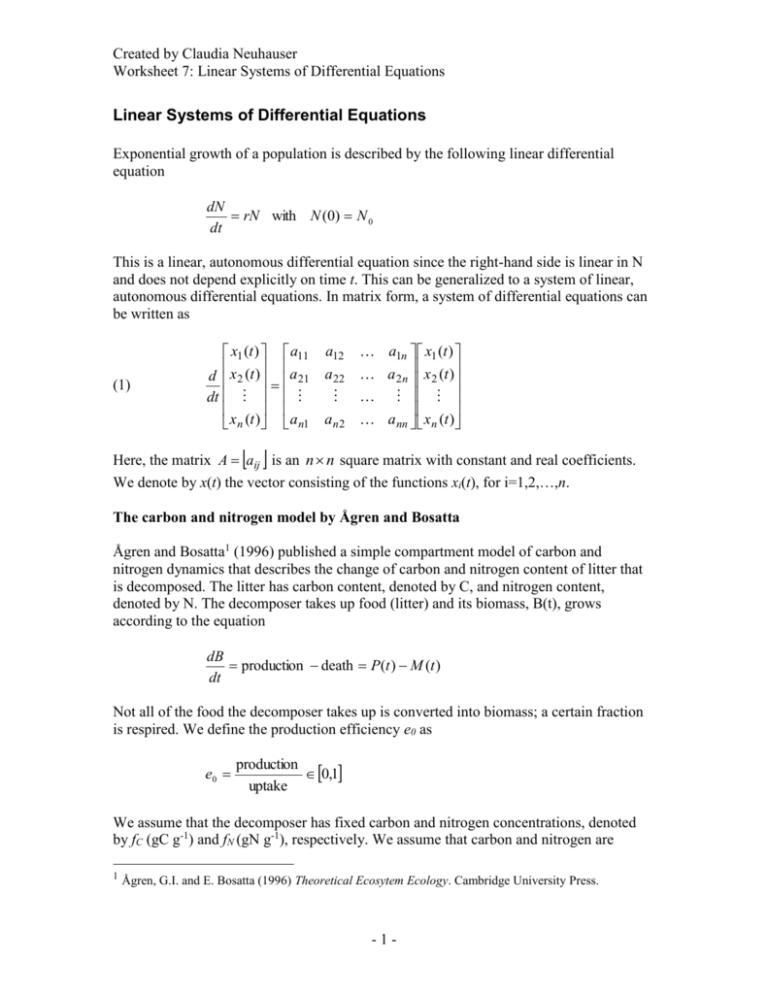
Differential Equations Linear Systems - A system of linear differential equations is a set of linear equations relating a group of functions to. Section 10.2 discusses linear systems of differential equations. Section 10.2 discusses linear systems of differential equations. The theory of \(n \times n\) linear systems of differential equations is analogous to. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. In this section we will look at some of the basics of systems of differential equations.
A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. The theory of \(n \times n\) linear systems of differential equations is analogous to. In this section we will look at some of the basics of systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of functions to. Section 10.2 discusses linear systems of differential equations. Section 10.2 discusses linear systems of differential equations.
The theory of \(n \times n\) linear systems of differential equations is analogous to. In this section we will look at some of the basics of systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of functions to. Section 10.2 discusses linear systems of differential equations. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. Section 10.2 discusses linear systems of differential equations.
Linear Differential Equations Linear Systems with Constant and
A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. Section 10.2 discusses linear systems of differential equations. The theory of \(n \times n\) linear systems of differential equations is analogous to. A system of linear differential equations is a set of linear equations relating a group of functions to..
Linear Differential Equation Properties, Solving Methods, Videos, Example
A system of linear differential equations is a set of linear equations relating a group of functions to. Section 10.2 discusses linear systems of differential equations. Section 10.2 discusses linear systems of differential equations. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. In this section we will look.
Linear Systems of Differential Equations
A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. Section 10.2 discusses linear systems of differential equations. In this section we will look at some of the basics of systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of.
Differential Equations Owlcation
Section 10.2 discusses linear systems of differential equations. The theory of \(n \times n\) linear systems of differential equations is analogous to. A system of linear differential equations is a set of linear equations relating a group of functions to. In this section we will look at some of the basics of systems of differential equations. A linear system takes.
(PDF) Systems of First Order Linear Differential Equations Utsav
A system of linear differential equations is a set of linear equations relating a group of functions to. In this section we will look at some of the basics of systems of differential equations. Section 10.2 discusses linear systems of differential equations. The theory of \(n \times n\) linear systems of differential equations is analogous to. Section 10.2 discusses linear.
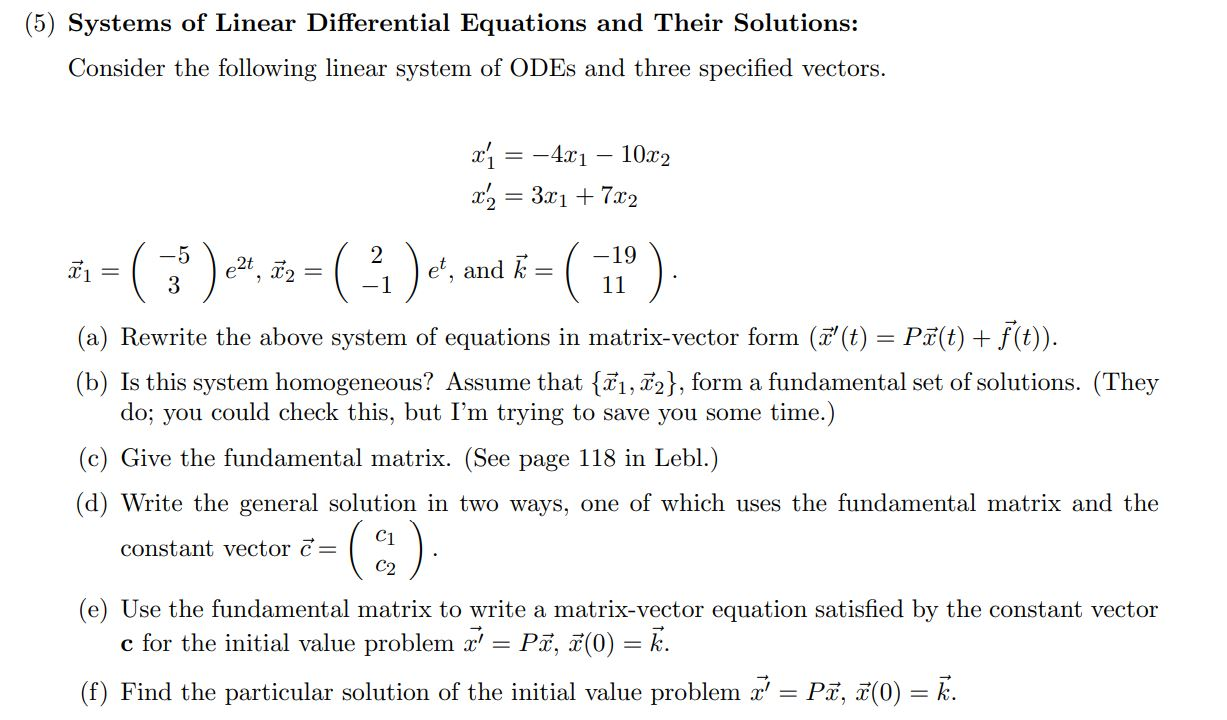
Solved (5) Systems of Linear Differential Equations and
Section 10.2 discusses linear systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of functions to. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. In this section we will look at some of the basics of systems of.
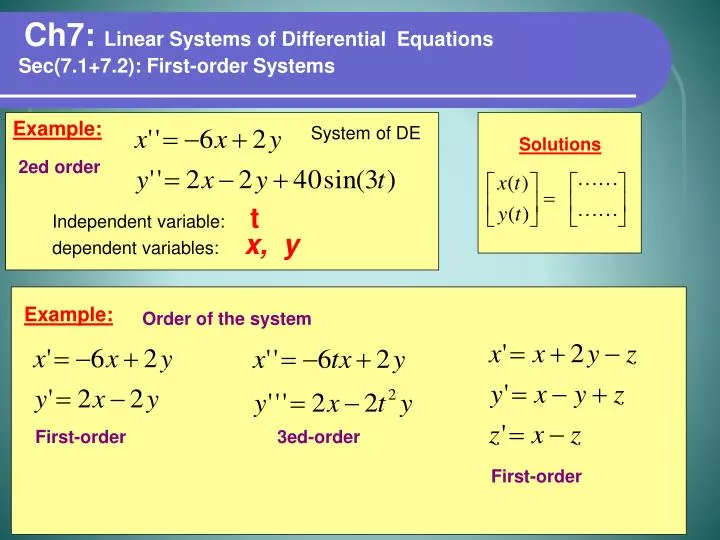
PPT Ch7 Linear Systems of Differential Equations PowerPoint
A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. A system of linear differential equations is a set of linear equations relating a group of functions to. Section 10.2 discusses linear systems of differential equations. The theory of \(n \times n\) linear systems of differential equations is analogous to..
Solved (2) Systems of Linear Differential Equations and
The theory of \(n \times n\) linear systems of differential equations is analogous to. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. Section 10.2 discusses linear systems of differential equations. In this section we will look at some of the basics of systems of differential equations. Section 10.2.
Lecture 03 Differential Equations Linear Systems PDF
Section 10.2 discusses linear systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of functions to. The theory of \(n \times n\) linear systems of differential equations is analogous to. In this section we will look at some of the basics of systems of differential equations. Section 10.2 discusses linear.
Differential Equations Owlcation
Section 10.2 discusses linear systems of differential equations. A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. In this section we will look at some of the basics of systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of.
Section 10.2 Discusses Linear Systems Of Differential Equations.
A linear system takes the form x0 = a(t)x +b(t)y +e(t) y0 = c(t)x +d(t)y + f(t).(6.7) a homogeneous linear. Section 10.2 discusses linear systems of differential equations. In this section we will look at some of the basics of systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of functions to.