Differential Equations With Matrices - In this section we will give a brief review of matrices and vectors. Let me start with five useful properties of determinants, for all square matrices. To get matrices instead of derivatives, we have three basic choices—forward or backward or. U(t) = c1eλ1tx1 + c2eλ2tx2. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. Is eλ1tx 1 really a solution to d dt u =.
U(t) = c1eλ1tx1 + c2eλ2tx2. To get matrices instead of derivatives, we have three basic choices—forward or backward or. Is eλ1tx 1 really a solution to d dt u =. In this section we will give a brief review of matrices and vectors. Let me start with five useful properties of determinants, for all square matrices. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving.
In this section we will give a brief review of matrices and vectors. To get matrices instead of derivatives, we have three basic choices—forward or backward or. U(t) = c1eλ1tx1 + c2eλ2tx2. Let me start with five useful properties of determinants, for all square matrices. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. Is eλ1tx 1 really a solution to d dt u =.
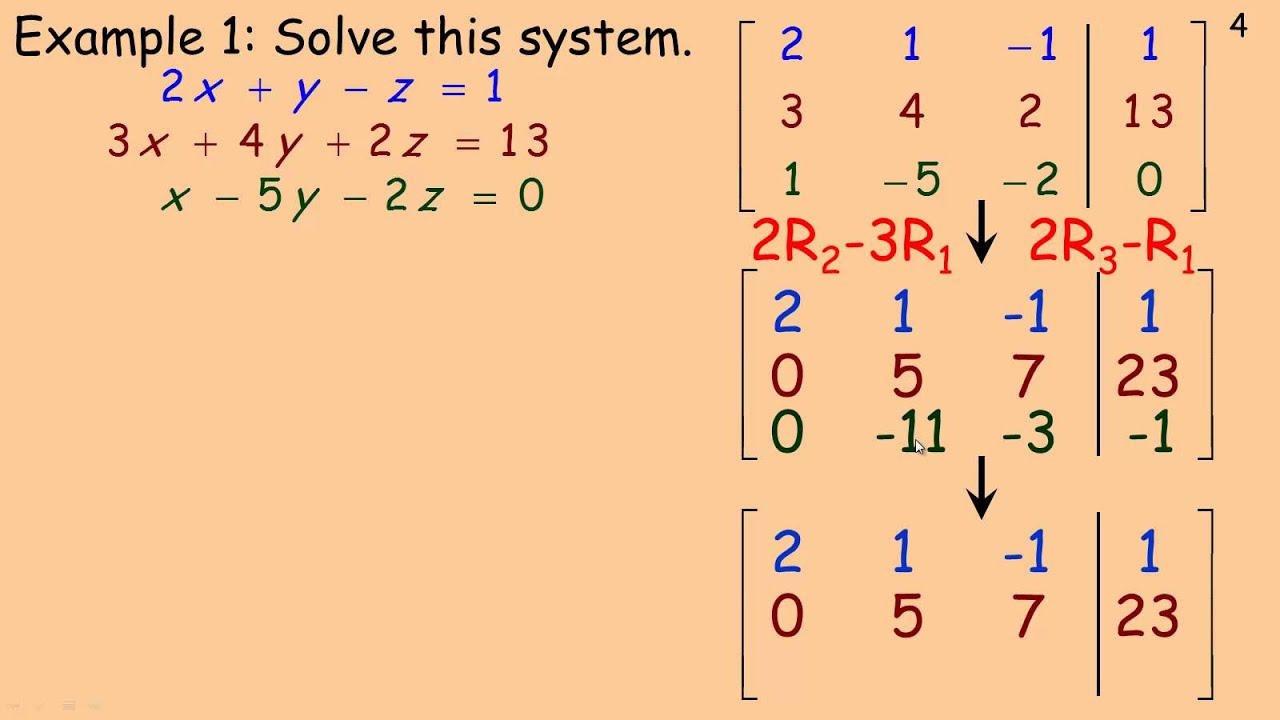
MATRICES Using matrices to solve Systems of Equations
Is eλ1tx 1 really a solution to d dt u =. U(t) = c1eλ1tx1 + c2eλ2tx2. In this section we will give a brief review of matrices and vectors. Let me start with five useful properties of determinants, for all square matrices. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving.
MATRICES Using matrices to solve Systems of Equations
Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. In this section we will give a brief review of matrices and vectors. To get matrices instead of derivatives, we have three basic choices—forward or backward or. Let me start with five useful properties of determinants, for all square matrices. Is eλ1tx 1 really a solution to.
Textbooks Differential Equations Freeup
Is eλ1tx 1 really a solution to d dt u =. To get matrices instead of derivatives, we have three basic choices—forward or backward or. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. Let me start with five useful properties of determinants, for all square matrices. In this section we will give a brief review.
Solving 3 Systems Of Equations Using Matrices Tessshebaylo
Is eλ1tx 1 really a solution to d dt u =. U(t) = c1eλ1tx1 + c2eλ2tx2. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. Let me start with five useful properties of determinants, for all square matrices. To get matrices instead of derivatives, we have three basic choices—forward or backward or.
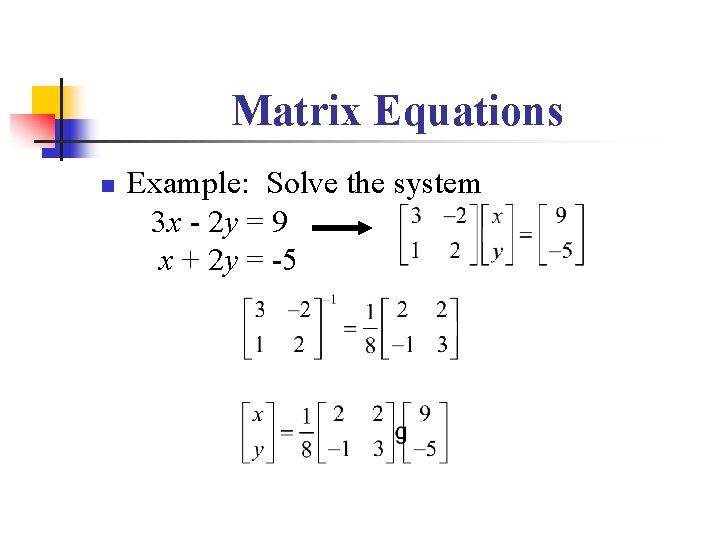
Solve 2 Variable Equations Using Matrices Tessshebaylo
In this section we will give a brief review of matrices and vectors. Is eλ1tx 1 really a solution to d dt u =. U(t) = c1eλ1tx1 + c2eλ2tx2. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. To get matrices instead of derivatives, we have three basic choices—forward or backward or.
Matrices differential equations
In this section we will give a brief review of matrices and vectors. Is eλ1tx 1 really a solution to d dt u =. To get matrices instead of derivatives, we have three basic choices—forward or backward or. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. Let me start with five useful properties of determinants,.
Modelling Motion with Differential Equations
To get matrices instead of derivatives, we have three basic choices—forward or backward or. Let me start with five useful properties of determinants, for all square matrices. U(t) = c1eλ1tx1 + c2eλ2tx2. In this section we will give a brief review of matrices and vectors. Is eλ1tx 1 really a solution to d dt u =.
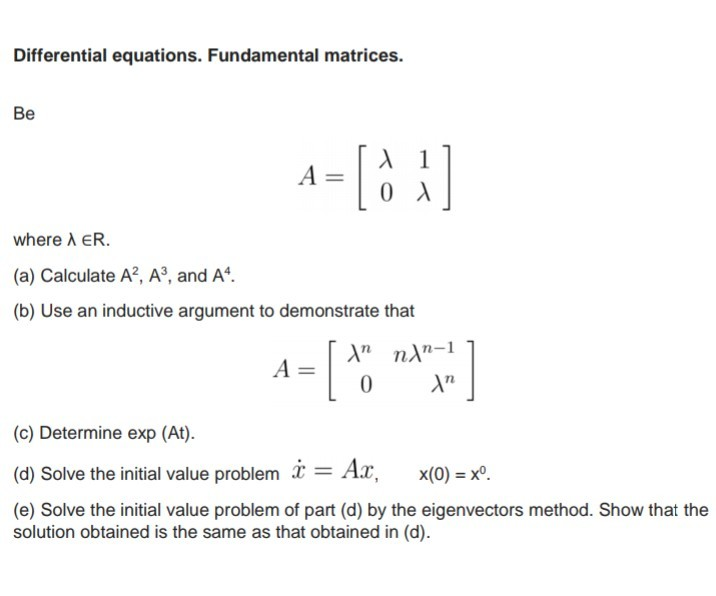
Solved Differential equations. Fundamental matrices. Be
In this section we will give a brief review of matrices and vectors. To get matrices instead of derivatives, we have three basic choices—forward or backward or. Let me start with five useful properties of determinants, for all square matrices. U(t) = c1eλ1tx1 + c2eλ2tx2. Is eλ1tx 1 really a solution to d dt u =.
Matrices And Linear System Of Equations Pdf Tessshebaylo
Is eλ1tx 1 really a solution to d dt u =. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. U(t) = c1eλ1tx1 + c2eλ2tx2. In this section we will give a brief review of matrices and vectors. To get matrices instead of derivatives, we have three basic choices—forward or backward or.
(PDF) Compound matrices and ordinary differential equations
In this section we will give a brief review of matrices and vectors. Is eλ1tx 1 really a solution to d dt u =. U(t) = c1eλ1tx1 + c2eλ2tx2. To get matrices instead of derivatives, we have three basic choices—forward or backward or. Linear algebra, particularly the study of matrices, is fundamental in understanding and solving.
Let Me Start With Five Useful Properties Of Determinants, For All Square Matrices.
Linear algebra, particularly the study of matrices, is fundamental in understanding and solving. To get matrices instead of derivatives, we have three basic choices—forward or backward or. U(t) = c1eλ1tx1 + c2eλ2tx2. In this section we will give a brief review of matrices and vectors.

/aae14611c6e6e45b3bd99783f827f01c.jpg)