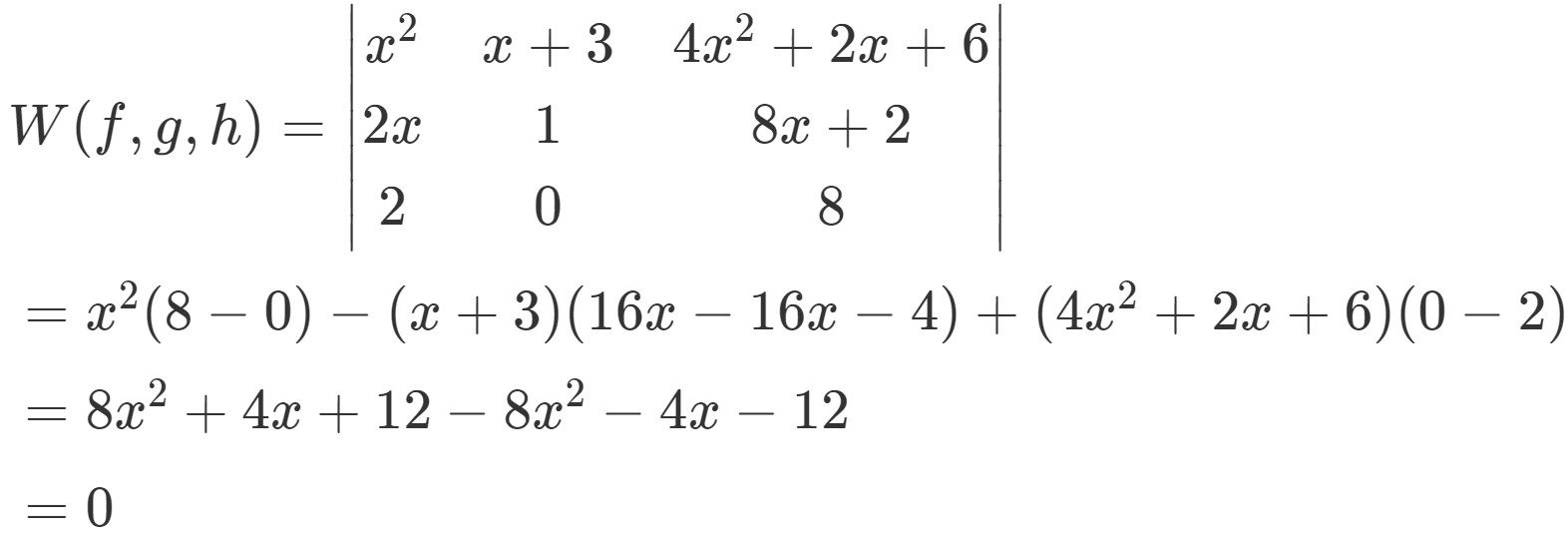Differential Equations Wronskian - If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}. In this section we will examine how the wronskian, introduced in the previous section,.
If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. In this section we will examine how the wronskian, introduced in the previous section,. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,.
In this section we will examine how the wronskian, introduced in the previous section,. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,. If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and.
Wronskian StudyPug
The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}. In this section we will examine how the wronskian, introduced in the previous section,. If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,.
The Wronskian Edge in Differential Equations Simplification and Solutions
The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}. If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,. In this section we will examine how the wronskian, introduced in the previous section,.
Ordinary Differential Equations Wronskian of X 3 and X 2 X
In this section we will examine how the wronskian, introduced in the previous section,. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,. If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}.
Wronskian, differential, determinant
If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. In this section we will examine how the wronskian, introduced in the previous section,. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}.
SOLUTION Differential equations wronskian determinant higher order
If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}. In this section we will examine how the wronskian, introduced in the previous section,. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,.
SOLUTION Differential equations wronskian determinant higher order
The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}. In this section we will examine how the wronskian, introduced in the previous section,. If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and.
Wronskian Analysis Example Worksheet 5 Differential Equations CN
The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}. If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. In this section we will examine how the wronskian, introduced in the previous section,. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,.
The Wronskian Edge in Differential Equations Simplification and Solutions
In this section we will examine how the wronskian, introduced in the previous section,. If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}.
Ordinary Differential Equations Wronskian Friday, September 30
In this section we will examine how the wronskian, introduced in the previous section,. If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}.
[Solved] Match the second order linear equations with the Wronskian of
In this section we will examine how the wronskian, introduced in the previous section,. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}. If the wronskian of [latex]f[/latex] and [latex]g[/latex] is [latex]e^{t}\text{cos}(t)+\text{sin}(t)[/latex], and. The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,.
If The Wronskian Of [Latex]F[/Latex] And [Latex]G[/Latex] Is [Latex]E^{T}\Text{Cos}(T)+\Text{Sin}(T)[/Latex], And.
The wronskian of these n solutions is defined as, w(t) := det h x(1)(t),x(1)(t),.,x(1)(t) i,. The determinant is called the wronskian and is defined by \[w=x_{1} \dot{x}_{2}. In this section we will examine how the wronskian, introduced in the previous section,.