Differentiality

Differentiality - Some places define it as: If the left hand derivative and the right hand derivative at a point are equal. Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions: The theorems assure us that essentially all functions that we see in the course.

The theorems assure us that essentially all functions that we see in the course. If the left hand derivative and the right hand derivative at a point are equal. Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions: Some places define it as:

If the left hand derivative and the right hand derivative at a point are equal. Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions: The theorems assure us that essentially all functions that we see in the course. Some places define it as:

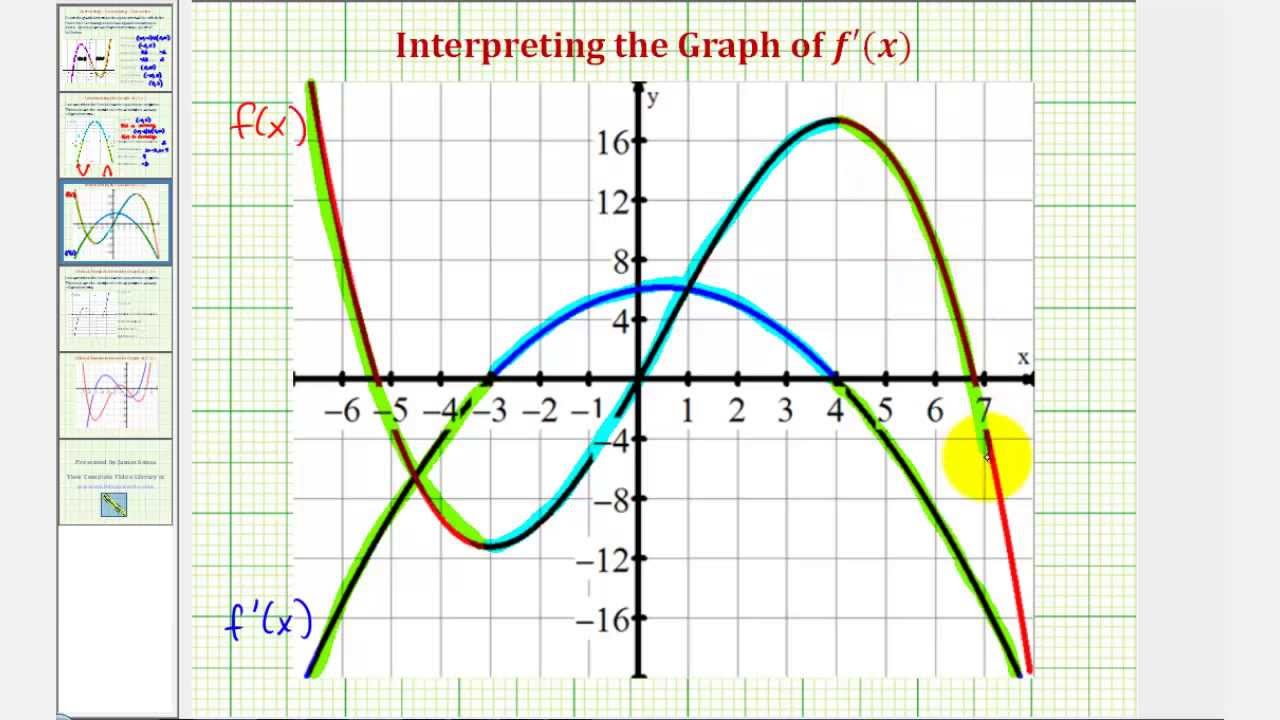
Ex 1 Interpret the Graph of the First Derivative Function Degree 2

Pin on School Stuff

Chapter 1 Differential YouTube

Some places define it as: Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions: The theorems assure us that essentially all functions that we see in the course. If the left hand derivative and the right hand derivative at a point are equal.

Differentials YouTube

Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions: If the left hand derivative and the right hand derivative at a point are equal. The theorems assure us that essentially all functions that we see in the course. Some places define it as:

Ex 1 Determine Differential y (dy) YouTube

Some places define it as: If the left hand derivative and the right hand derivative at a point are equal. The theorems assure us that essentially all functions that we see in the course. Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions:

Continuity and Differentiability YouTube

13 4 Calculate the Total Differential YouTube

The theorems assure us that essentially all functions that we see in the course. Some places define it as: If the left hand derivative and the right hand derivative at a point are equal. Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions:

Using Differentials YouTube

The theorems assure us that essentially all functions that we see in the course. Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions: If the left hand derivative and the right hand derivative at a point are equal. Some places define it as:

Total Differential Problems & Solutions Part 2 YouTube

Some places define it as: If the left hand derivative and the right hand derivative at a point are equal. Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions: The theorems assure us that essentially all functions that we see in the course.

Testing Multivariate Normality using R [Bengali] YouTube

If the left hand derivative and the right hand derivative at a point are equal. The theorems assure us that essentially all functions that we see in the course. Some places define it as: Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions:

Some Places Define It As:

Lim⁡ x→a f(x) exists lim⁡ x→a f(x) = f(a) characteristics of continuous functions: The theorems assure us that essentially all functions that we see in the course. If the left hand derivative and the right hand derivative at a point are equal.