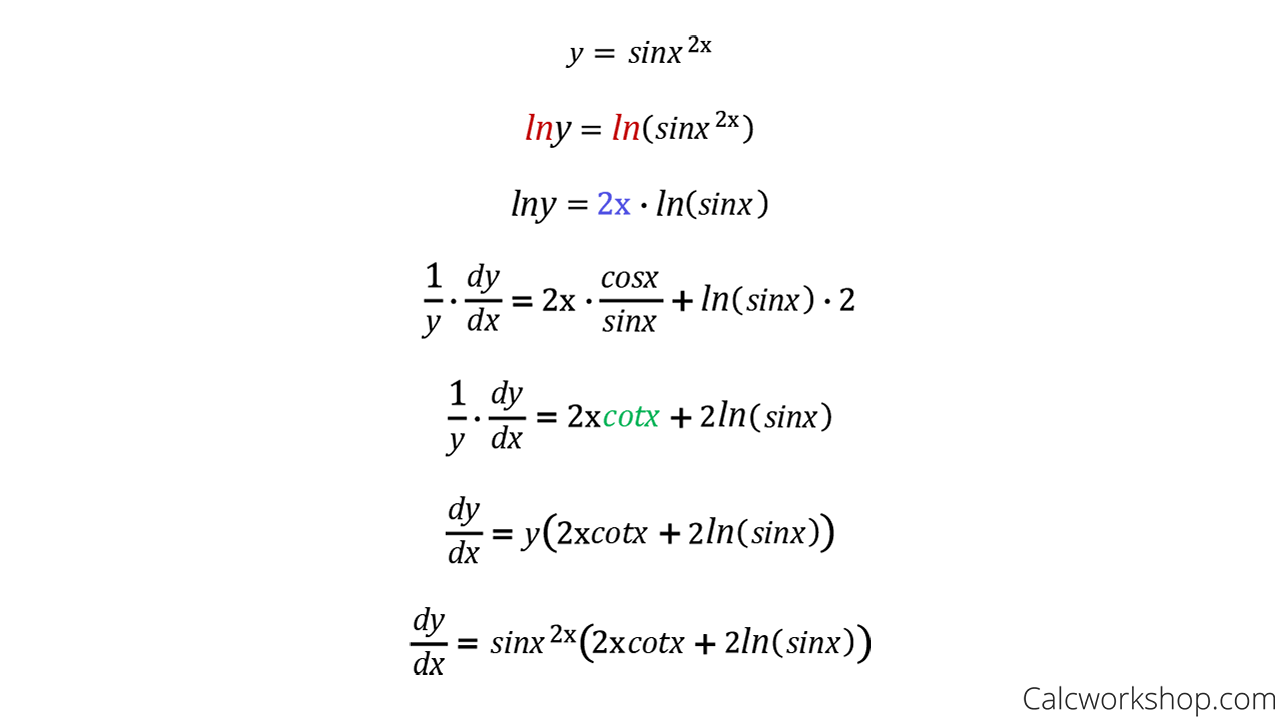
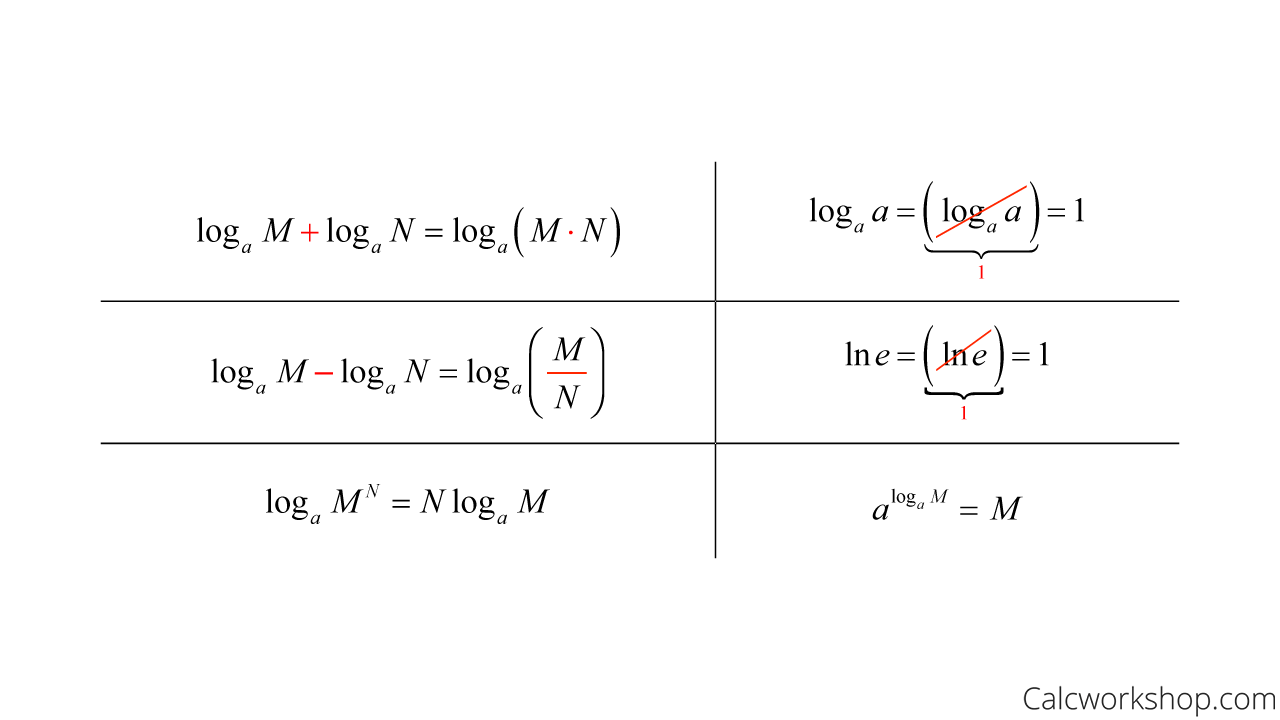
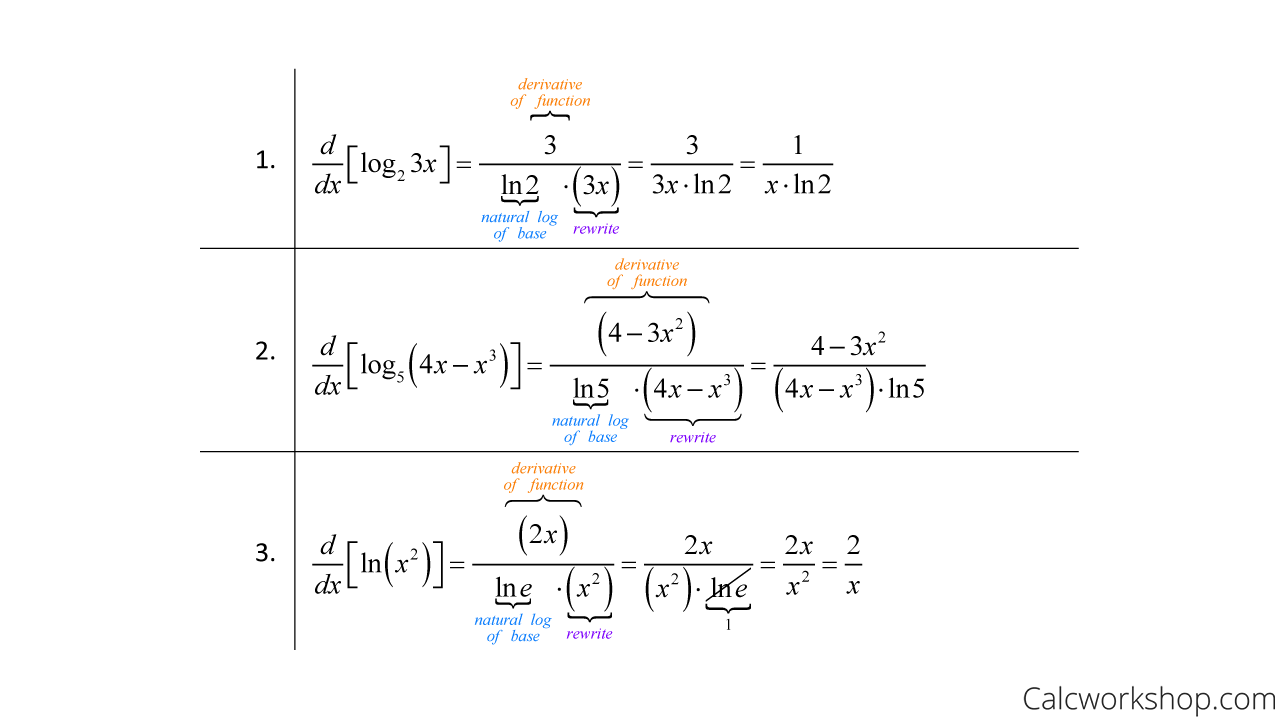
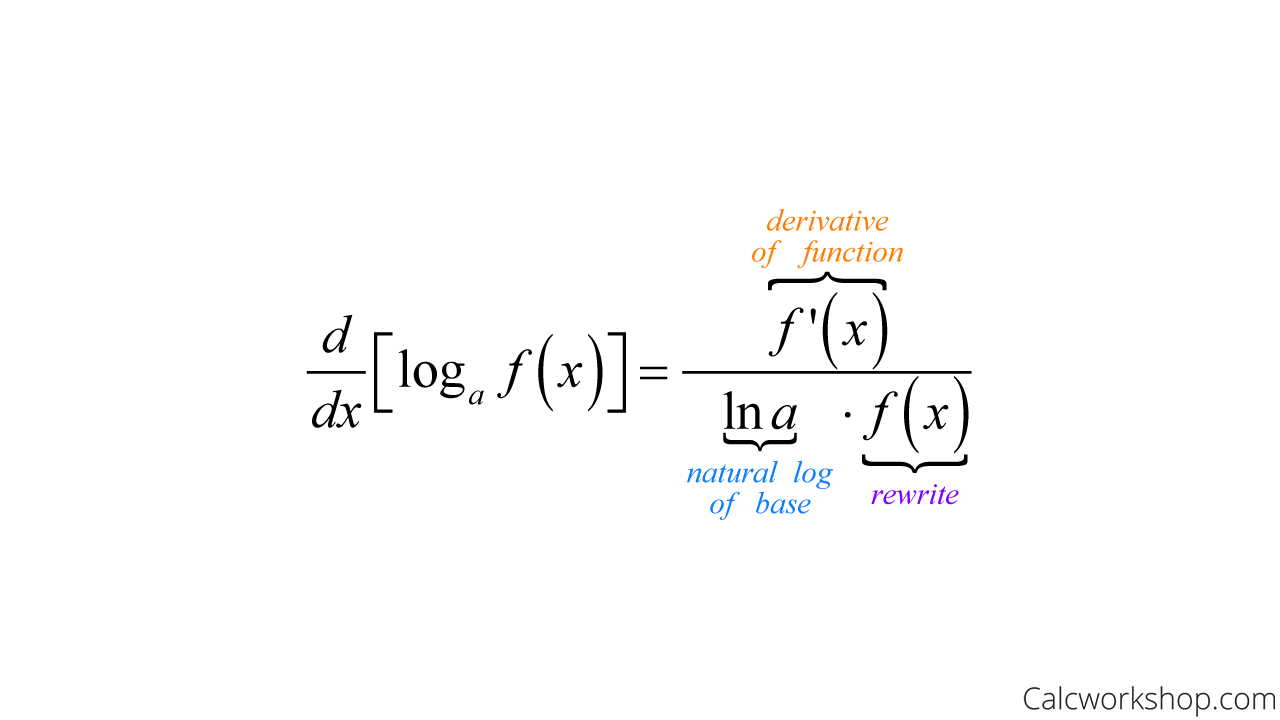
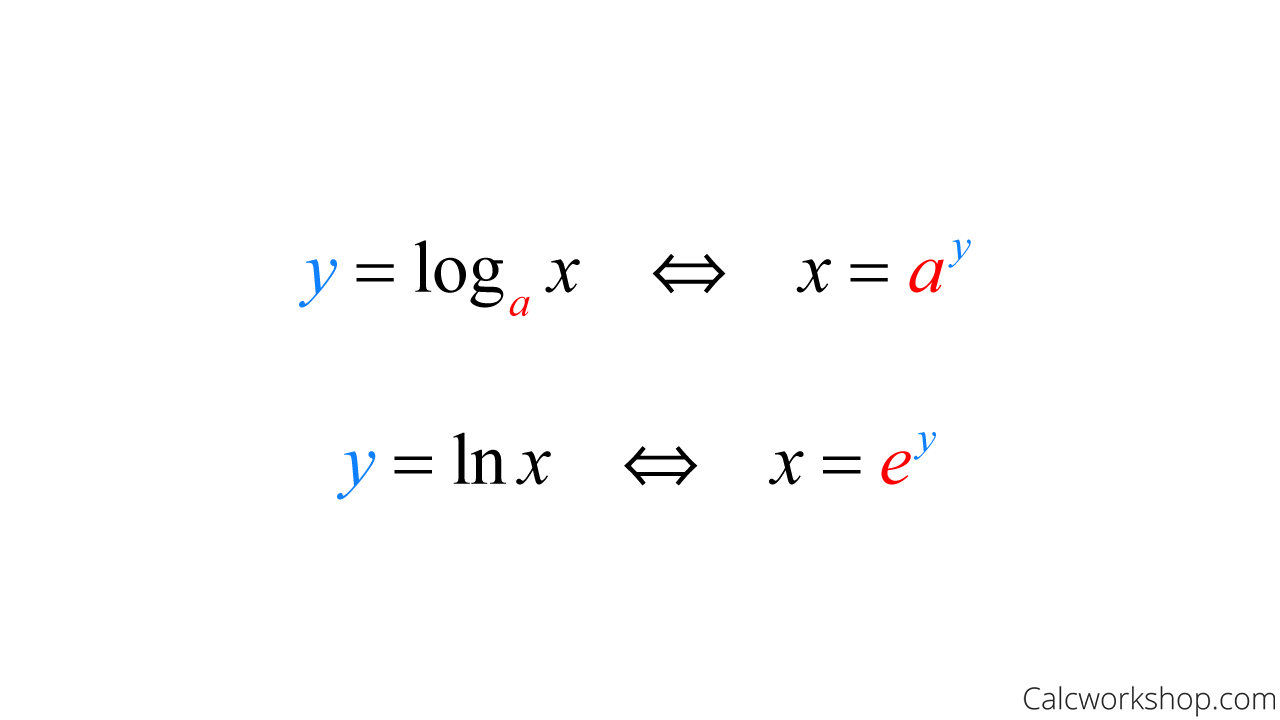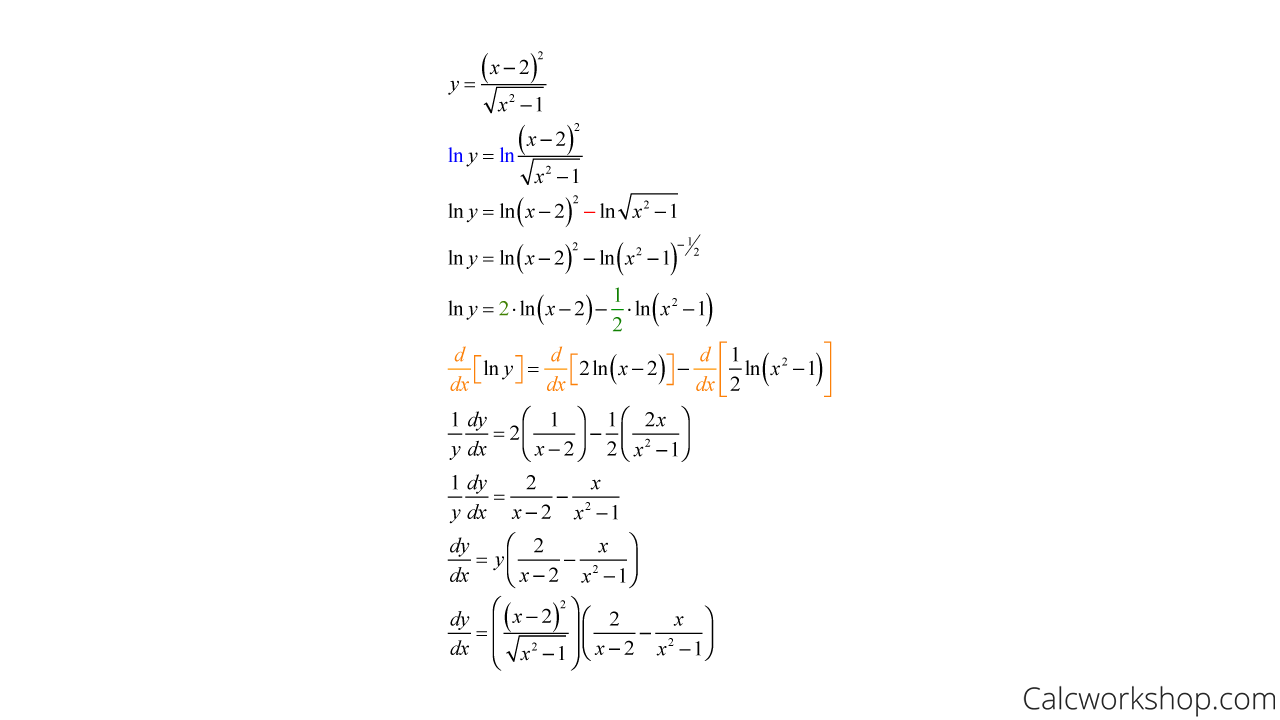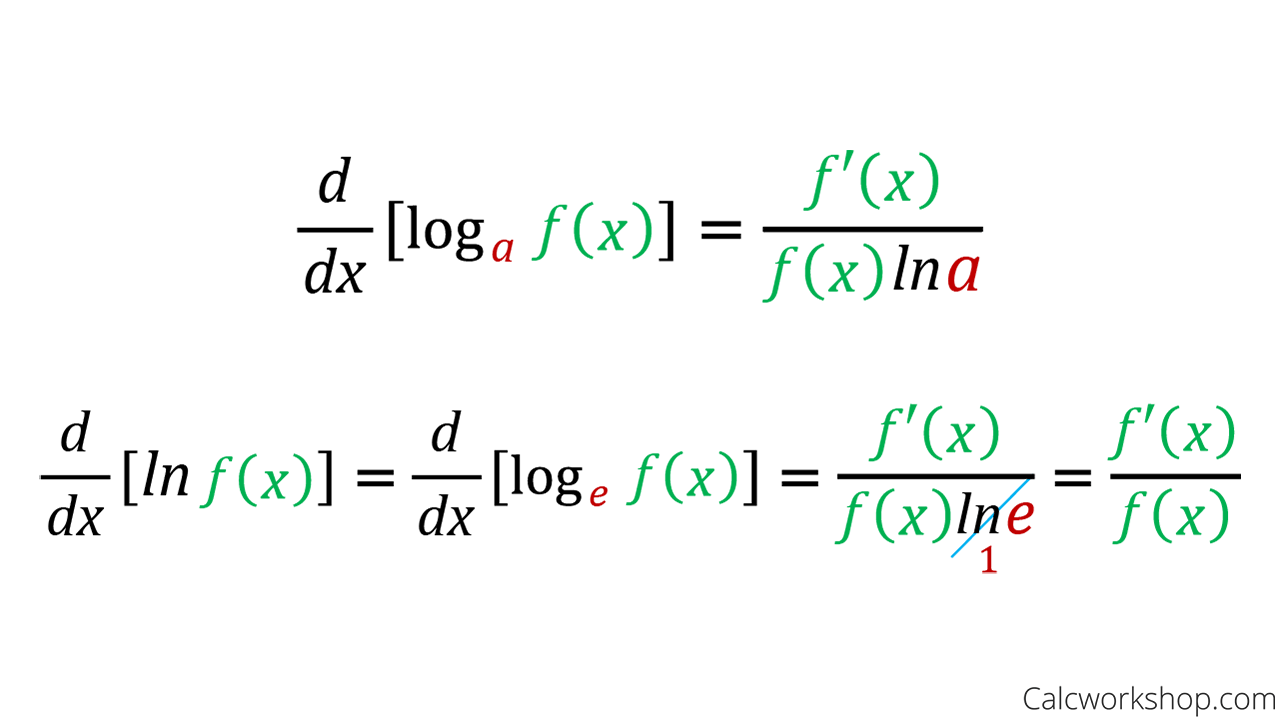Differentiating A Logarithmic Function - However, we can generalize it for any differentiable function with. Derivatives of logarithmic functions are mainly based on the chain rule. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Taking the derivatives of some complicated functions can be simplified by using logarithms. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by.
Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Taking the derivatives of some complicated functions can be simplified by using logarithms. Derivatives of logarithmic functions are mainly based on the chain rule. However, we can generalize it for any differentiable function with. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic.
Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Derivatives of logarithmic functions are mainly based on the chain rule. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. Taking the derivatives of some complicated functions can be simplified by using logarithms. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. However, we can generalize it for any differentiable function with.
50+ derivatives of logarithmic functions worksheets for 11th Year
Derivatives of logarithmic functions are mainly based on the chain rule. Taking the derivatives of some complicated functions can be simplified by using logarithms. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. However, we can.
Logarithmic Function Equation
However, we can generalize it for any differentiable function with. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Taking the derivatives of some complicated functions can be simplified by using logarithms. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Derivatives of logarithmic functions are.
What is Logarithmic Differentiation? (7 Powerful Examples!)
Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. However, we can generalize it for any differentiable function with. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Taking.
Logarithmic Differentiation (w/ 7 StepbyStep Examples!)
Derivatives of logarithmic functions are mainly based on the chain rule. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic..
Logarithmic Function Formula
Derivatives of logarithmic functions are mainly based on the chain rule. Taking the derivatives of some complicated functions can be simplified by using logarithms. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. However, we can generalize.
Logarithmic Function Formula
Taking the derivatives of some complicated functions can be simplified by using logarithms. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. However, we can generalize it for any differentiable function with. Derivatives of logarithmic functions are mainly based on the chain rule. Method of finding a function’s derivative by first taking the.
Logarithmic Differentiation (w/ 7 StepbyStep Examples!)
Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. However, we can generalize it for any differentiable function with. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Derivatives.
Derivatives of Logarithmic Functions (Fully Explained!)
Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. Taking the derivatives of some complicated functions can be simplified by using logarithms. Derivatives of logarithmic functions are mainly based on the chain rule. However, we can generalize it for any differentiable function with. Logarithmic differentiation allows us to differentiate functions of the.
PPT The Natural Logarithmic Function PowerPoint Presentation, free
Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. However, we can generalize it for any differentiable function with. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Taking.
Derivatives of Logarithmic Functions
However, we can generalize it for any differentiable function with. Derivatives of logarithmic functions are mainly based on the chain rule. Taking the derivatives of some complicated functions can be simplified by using logarithms. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Method of finding a function’s derivative by first taking the.
Derivatives Of Logarithmic Functions Are Mainly Based On The Chain Rule.
However, we can generalize it for any differentiable function with. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Method of finding a function’s derivative by first taking the logarithm and then differentiating is called logarithmic. Taking the derivatives of some complicated functions can be simplified by using logarithms.