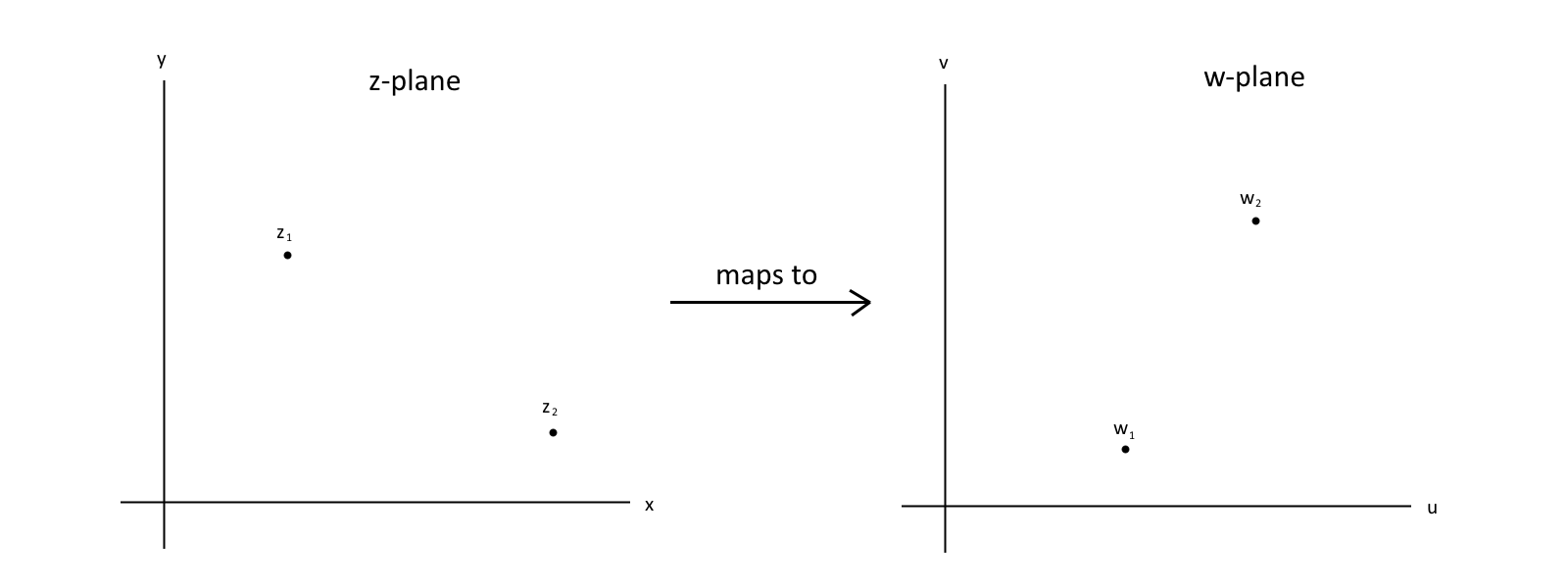
Differentiation Of Complex Functions - A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part. In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable. A book chapter that introduces the definition, properties and examples of complex differentiation.
A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part. A book chapter that introduces the definition, properties and examples of complex differentiation. In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable.
In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable. A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part. A book chapter that introduces the definition, properties and examples of complex differentiation.
Complex Variables Differentiation and Integration of
A book chapter that introduces the definition, properties and examples of complex differentiation. A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part. In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable.
Complex Functions
In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable. A book chapter that introduces the definition, properties and examples of complex differentiation. A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part.
Differentiation With Complex Functions
A book chapter that introduces the definition, properties and examples of complex differentiation. A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part. In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable.
Problem Set 4, Complex FunctionsDifferential EquationsAssignment
A book chapter that introduces the definition, properties and examples of complex differentiation. In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable. A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part.
Complex Functions
A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part. A book chapter that introduces the definition, properties and examples of complex differentiation. In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable.
Analysis of Complex Functions and Their Properties PDF Continuous
A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part. In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable. A book chapter that introduces the definition, properties and examples of complex differentiation.
Complex Functions Complex Plane COMPLEX FUNCTIONS ASSIGNMENT 2
A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part. A book chapter that introduces the definition, properties and examples of complex differentiation. In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable.
Problems On Differentiation of Complex Functions PDF
A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part. A book chapter that introduces the definition, properties and examples of complex differentiation. In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable.
Complex Functions An Algebraic and Geometric Viewpoint 1, Jones
A book chapter that introduces the definition, properties and examples of complex differentiation. In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable. A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part.
Complex Derivative Detailed Explanation and Examples The Story of
In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable. A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part. A book chapter that introduces the definition, properties and examples of complex differentiation.
A Book Chapter That Introduces The Definition, Properties And Examples Of Complex Differentiation.
In the post, we will learn about complex differentiation where we study the derivative of functions of a complex variable. A complex function \(f(z)=u(x,y)+iv(x,y)\) has a complex derivative \(f′(z)\) if and only if its real and imaginary part.