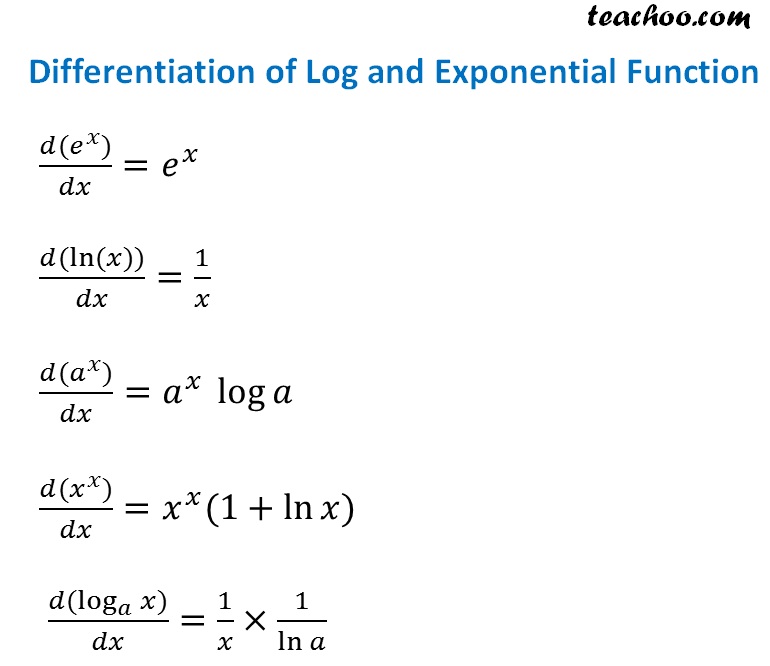
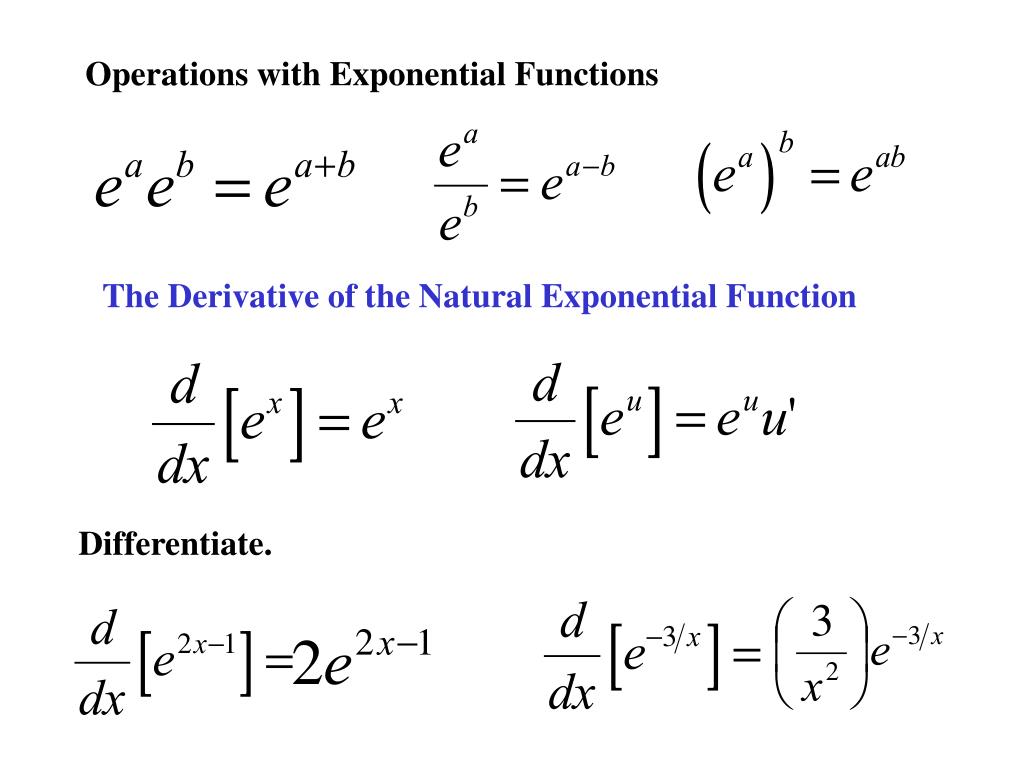
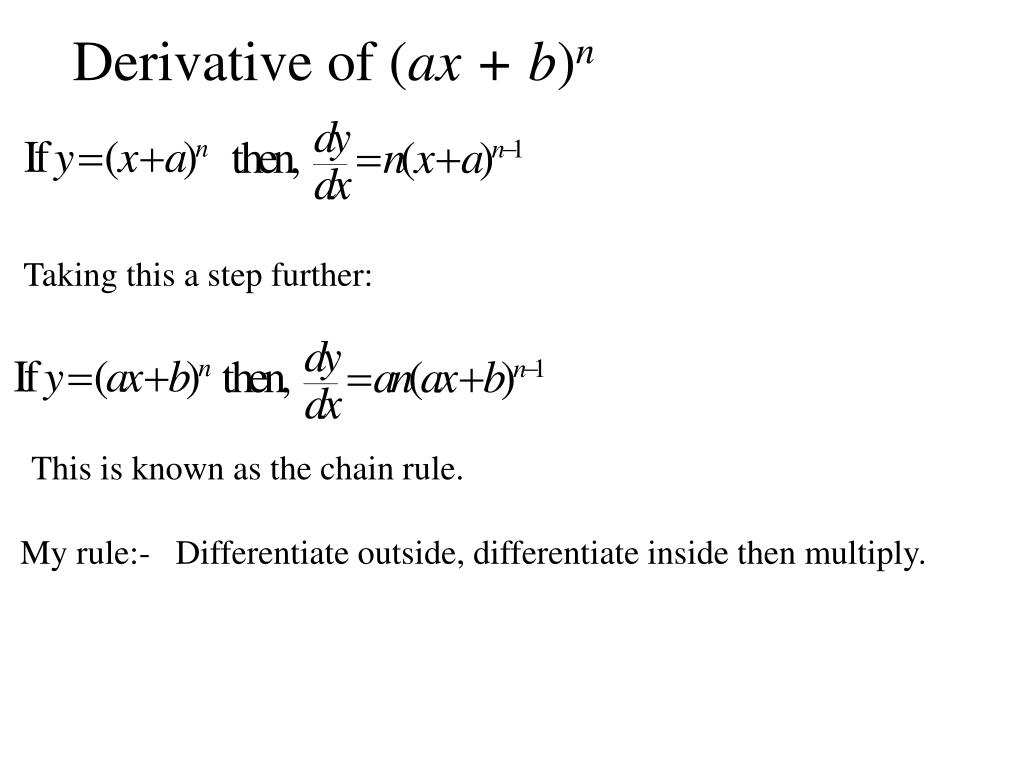
Differentiation Of E Ax - \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more What is the first derivative of e^ {ax} ? \[y=e^x \nonumber \] is defined as the inverse of \[\ln x.\nonumber \] therefore \[\ln(e^x) = x \nonumber \] and \[e^{\ln x} =x. The derivative of #e^(f(x))# (with respect to #x#) is #e^(f(x)) f'(x)#. The differentiation of an exponential function is done by using the standard formula for differentiation of exponential function, the. In this case #f(x)=ax# and #f'(x)=a#. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = ex f (x) = e x and g(x).
The derivative of #e^(f(x))# (with respect to #x#) is #e^(f(x)) f'(x)#. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = ex f (x) = e x and g(x). The differentiation of an exponential function is done by using the standard formula for differentiation of exponential function, the. \[y=e^x \nonumber \] is defined as the inverse of \[\ln x.\nonumber \] therefore \[\ln(e^x) = x \nonumber \] and \[e^{\ln x} =x. What is the first derivative of e^ {ax} ? \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more In this case #f(x)=ax# and #f'(x)=a#.
\int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more In this case #f(x)=ax# and #f'(x)=a#. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = ex f (x) = e x and g(x). \[y=e^x \nonumber \] is defined as the inverse of \[\ln x.\nonumber \] therefore \[\ln(e^x) = x \nonumber \] and \[e^{\ln x} =x. The derivative of #e^(f(x))# (with respect to #x#) is #e^(f(x)) f'(x)#. What is the first derivative of e^ {ax} ? The differentiation of an exponential function is done by using the standard formula for differentiation of exponential function, the.
Differentiation Rules
Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = ex f (x) = e x and g(x). In this case #f(x)=ax# and #f'(x)=a#. The differentiation of an exponential function is done by using the standard formula for.
Differentiation Rules
\[y=e^x \nonumber \] is defined as the inverse of \[\ln x.\nonumber \] therefore \[\ln(e^x) = x \nonumber \] and \[e^{\ln x} =x. In this case #f(x)=ax# and #f'(x)=a#. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more What is the first derivative of e^ {ax} ? Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f.
PPT 5.4 Exponential Functions Differentiation and Integration
Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = ex f (x) = e x and g(x). \[y=e^x \nonumber \] is defined as the inverse of \[\ln x.\nonumber \] therefore \[\ln(e^x) = x \nonumber \] and \[e^{\ln.
Differentiation of Tan Inverse x Explanation, Formula, Examples
The derivative of #e^(f(x))# (with respect to #x#) is #e^(f(x)) f'(x)#. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = ex f (x) = e x and g(x). \[y=e^x \nonumber \] is defined as the inverse of.
PPT Further Differentiation and Integration PowerPoint Presentation
The differentiation of an exponential function is done by using the standard formula for differentiation of exponential function, the. The derivative of #e^(f(x))# (with respect to #x#) is #e^(f(x)) f'(x)#. \[y=e^x \nonumber \] is defined as the inverse of \[\ln x.\nonumber \] therefore \[\ln(e^x) = x \nonumber \] and \[e^{\ln x} =x. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Differentiate.
Derivative of log ax psadonavigator
\int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more What is the first derivative of e^ {ax} ? The derivative of #e^(f(x))# (with respect to #x#) is #e^(f(x)) f'(x)#. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = ex.
If y=e−αx, then find double differentiation of y Filo
The differentiation of an exponential function is done by using the standard formula for differentiation of exponential function, the. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = ex f (x) = e x and g(x). In.
Ex 7.2, 5 Integrate sin (ax + b) cos (ax + b) Teachoo
What is the first derivative of e^ {ax} ? In this case #f(x)=ax# and #f'(x)=a#. The differentiation of an exponential function is done by using the standard formula for differentiation of exponential function, the. \[y=e^x \nonumber \] is defined as the inverse of \[\ln x.\nonumber \] therefore \[\ln(e^x) = x \nonumber \] and \[e^{\ln x} =x. The derivative of #e^(f(x))#.
Solved I do not understand why e^axdx=e^ax/a, I think
In this case #f(x)=ax# and #f'(x)=a#. \[y=e^x \nonumber \] is defined as the inverse of \[\ln x.\nonumber \] therefore \[\ln(e^x) = x \nonumber \] and \[e^{\ln x} =x. The differentiation of an exponential function is done by using the standard formula for differentiation of exponential function, the. What is the first derivative of e^ {ax} ? Differentiate using the chain.
Example 31 Derivative of a^x Chapter 5 Class 12 Logarithmic Diff
Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = ex f (x) = e x and g(x). \[y=e^x \nonumber \] is defined as the inverse of \[\ln x.\nonumber \] therefore \[\ln(e^x) = x \nonumber \] and \[e^{\ln.
Differentiate Using The Chain Rule, Which States That D Dx [F (G(X))] D D X [F (G (X))] Is F '(G(X))G'(X) F ′ (G (X)) G ′ (X) Where F (X) = Ex F (X) = E X And G(X).
\[y=e^x \nonumber \] is defined as the inverse of \[\ln x.\nonumber \] therefore \[\ln(e^x) = x \nonumber \] and \[e^{\ln x} =x. In this case #f(x)=ax# and #f'(x)=a#. The differentiation of an exponential function is done by using the standard formula for differentiation of exponential function, the. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more
What Is The First Derivative Of E^ {Ax} ?
The derivative of #e^(f(x))# (with respect to #x#) is #e^(f(x)) f'(x)#.