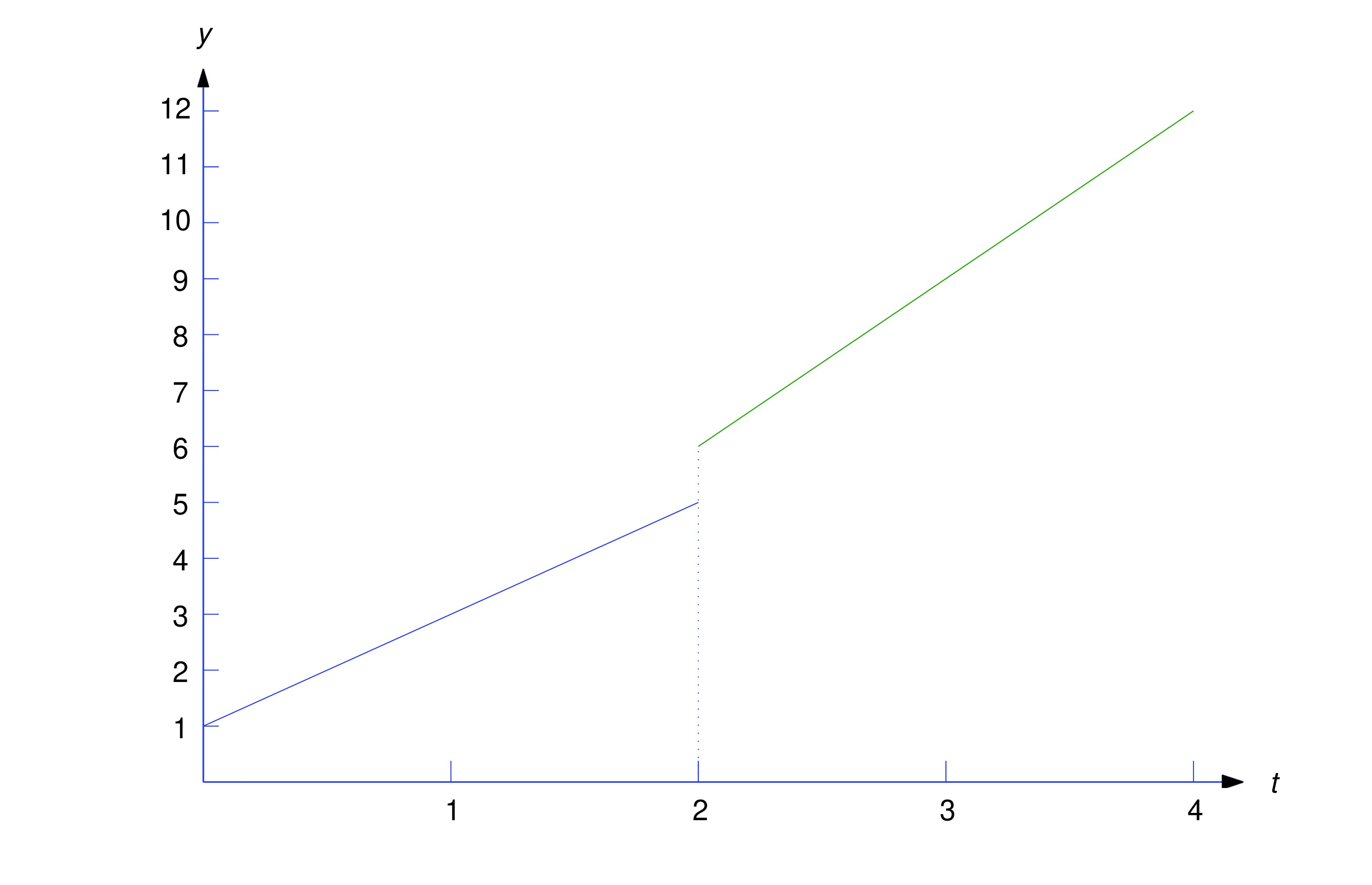
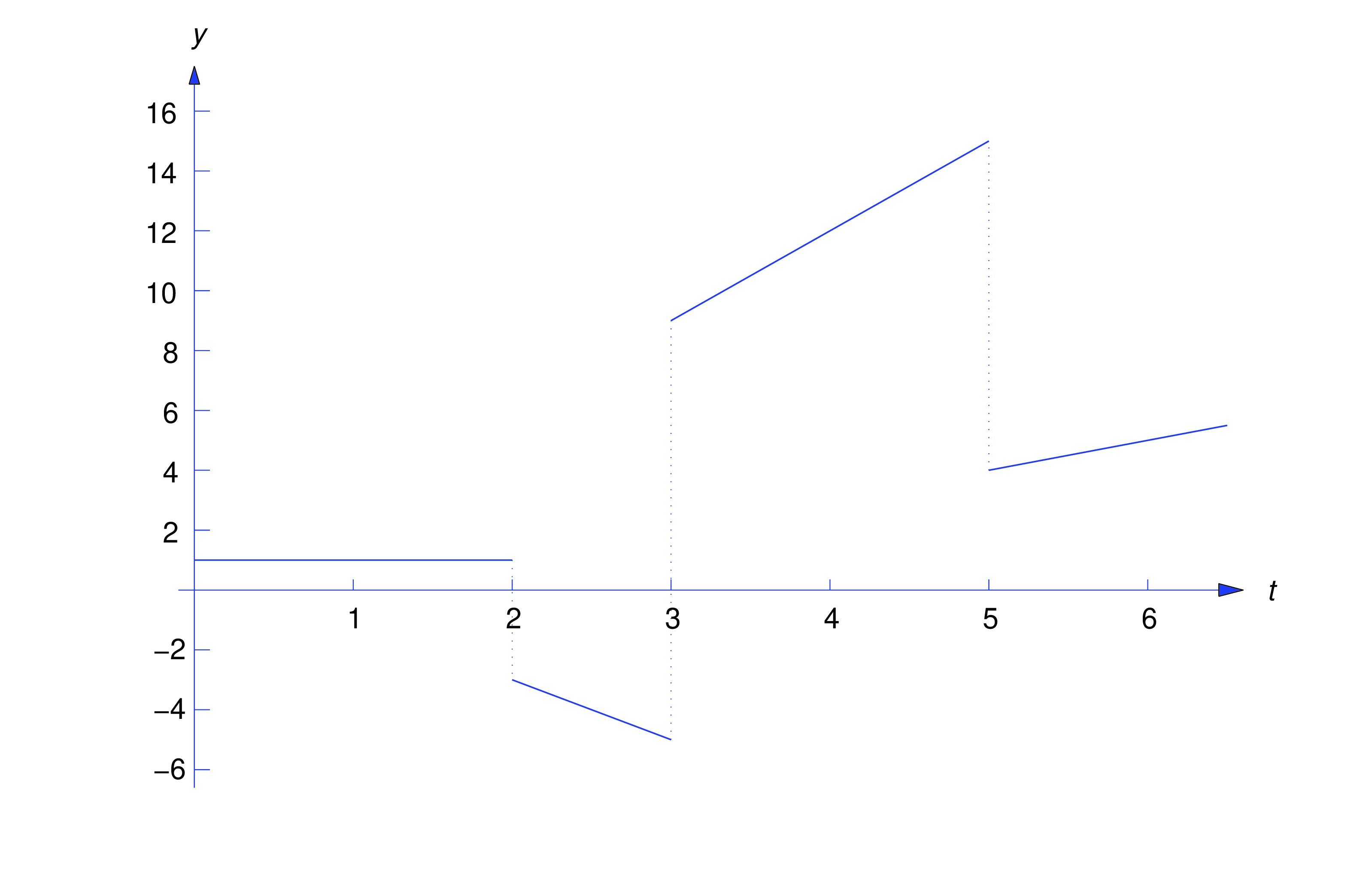
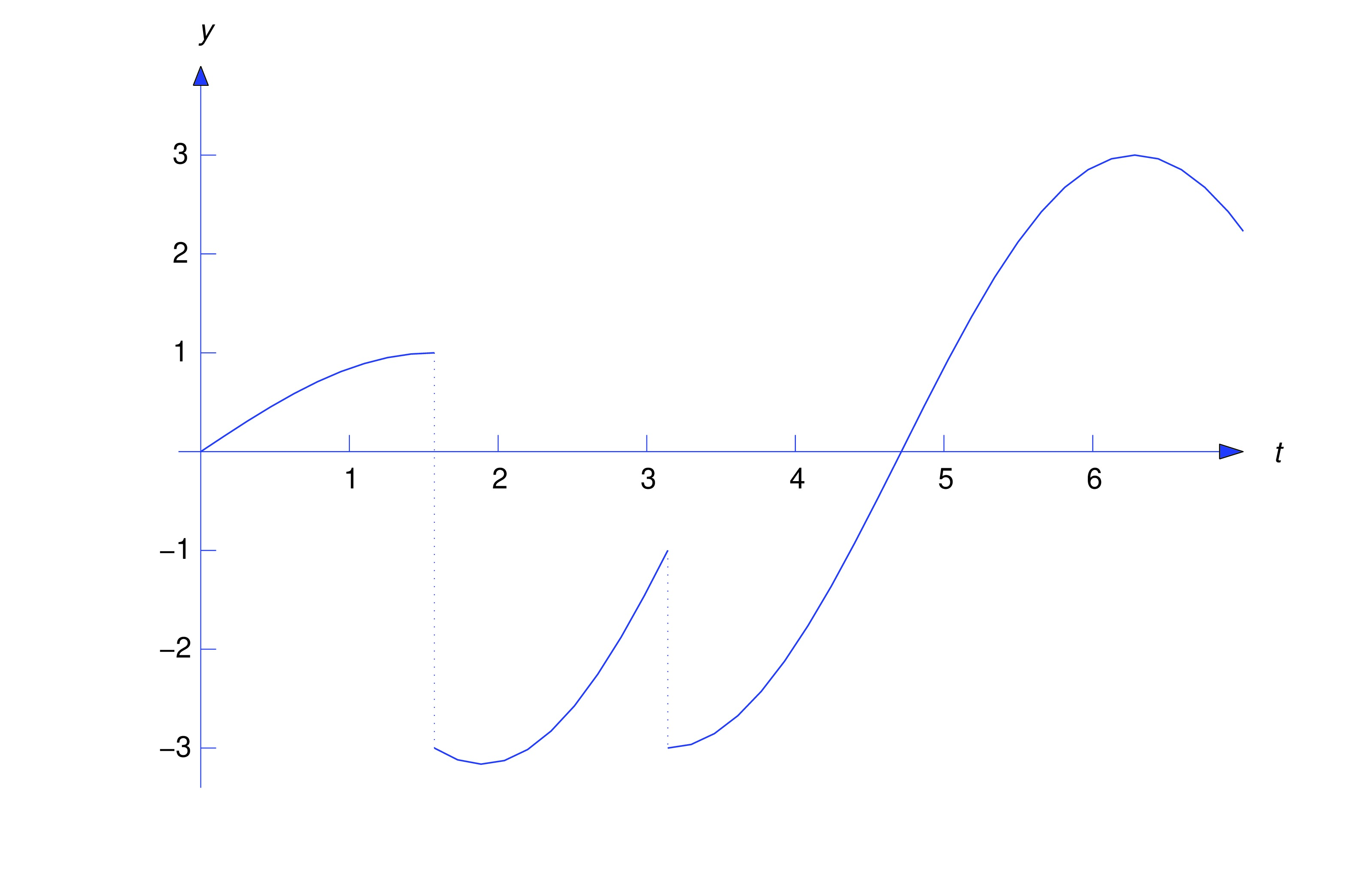
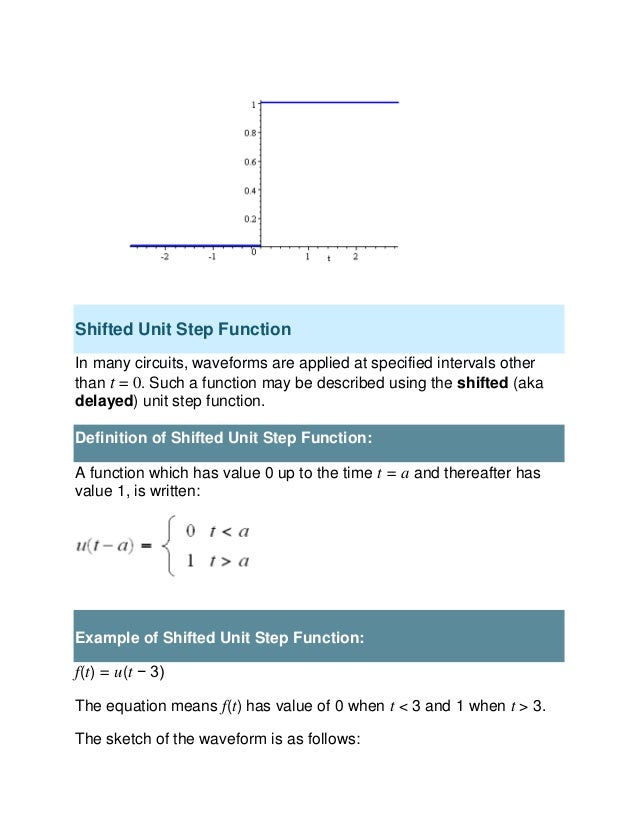
Differentiation Of Unit Step Function - The derivative of the unit step function (or heaviside function) is the dirac delta, which is a generalized function (or a. Where t = 0, the derivative of the unit step. Ut %() what is its derivative? () which has unit area. Now let's look at a signal: Just like the unit step function, the function is really an idealized view of nature. For this reason, the derivative of the unit step function is 0 at all points t, except where t = 0. The derivative of unit step $u(t)$ is dirac delta function $\delta(t)$, since an alternative definition of the unit step is using. Actually, with an appropriate mode of convergence, when a sequence of differentiable functions converge to the unit step, it can be. In reality, a delta function is nearly a spike near 0 which.
Ut %() what is its derivative? Where t = 0, the derivative of the unit step. For this reason, the derivative of the unit step function is 0 at all points t, except where t = 0. Just like the unit step function, the function is really an idealized view of nature. The derivative of unit step $u(t)$ is dirac delta function $\delta(t)$, since an alternative definition of the unit step is using. Now let's look at a signal: The derivative of the unit step function (or heaviside function) is the dirac delta, which is a generalized function (or a. In reality, a delta function is nearly a spike near 0 which. () which has unit area. Actually, with an appropriate mode of convergence, when a sequence of differentiable functions converge to the unit step, it can be.
In reality, a delta function is nearly a spike near 0 which. For this reason, the derivative of the unit step function is 0 at all points t, except where t = 0. Actually, with an appropriate mode of convergence, when a sequence of differentiable functions converge to the unit step, it can be. () which has unit area. The derivative of unit step $u(t)$ is dirac delta function $\delta(t)$, since an alternative definition of the unit step is using. Now let's look at a signal: The derivative of the unit step function (or heaviside function) is the dirac delta, which is a generalized function (or a. Ut %() what is its derivative? Where t = 0, the derivative of the unit step. Just like the unit step function, the function is really an idealized view of nature.
8.4 The Unit Step Function Ximera
In reality, a delta function is nearly a spike near 0 which. Actually, with an appropriate mode of convergence, when a sequence of differentiable functions converge to the unit step, it can be. For this reason, the derivative of the unit step function is 0 at all points t, except where t = 0. Ut %() what is its derivative?.
Unit Step Function Laplace Transform
Just like the unit step function, the function is really an idealized view of nature. Now let's look at a signal: Where t = 0, the derivative of the unit step. The derivative of the unit step function (or heaviside function) is the dirac delta, which is a generalized function (or a. () which has unit area.
Unit Step Function
For this reason, the derivative of the unit step function is 0 at all points t, except where t = 0. In reality, a delta function is nearly a spike near 0 which. Just like the unit step function, the function is really an idealized view of nature. The derivative of unit step $u(t)$ is dirac delta function $\delta(t)$, since.
8.4 The Unit Step Function Ximera
In reality, a delta function is nearly a spike near 0 which. Now let's look at a signal: Ut %() what is its derivative? () which has unit area. The derivative of unit step $u(t)$ is dirac delta function $\delta(t)$, since an alternative definition of the unit step is using.
Unit step function
The derivative of unit step $u(t)$ is dirac delta function $\delta(t)$, since an alternative definition of the unit step is using. Ut %() what is its derivative? Just like the unit step function, the function is really an idealized view of nature. Now let's look at a signal: Where t = 0, the derivative of the unit step.
Unit step function
The derivative of unit step $u(t)$ is dirac delta function $\delta(t)$, since an alternative definition of the unit step is using. () which has unit area. Where t = 0, the derivative of the unit step. For this reason, the derivative of the unit step function is 0 at all points t, except where t = 0. Just like the.
Unit step function
() which has unit area. For this reason, the derivative of the unit step function is 0 at all points t, except where t = 0. Now let's look at a signal: Actually, with an appropriate mode of convergence, when a sequence of differentiable functions converge to the unit step, it can be. In reality, a delta function is nearly.
8.4 The Unit Step Function Ximera
Actually, with an appropriate mode of convergence, when a sequence of differentiable functions converge to the unit step, it can be. () which has unit area. Where t = 0, the derivative of the unit step. Just like the unit step function, the function is really an idealized view of nature. The derivative of unit step $u(t)$ is dirac delta.
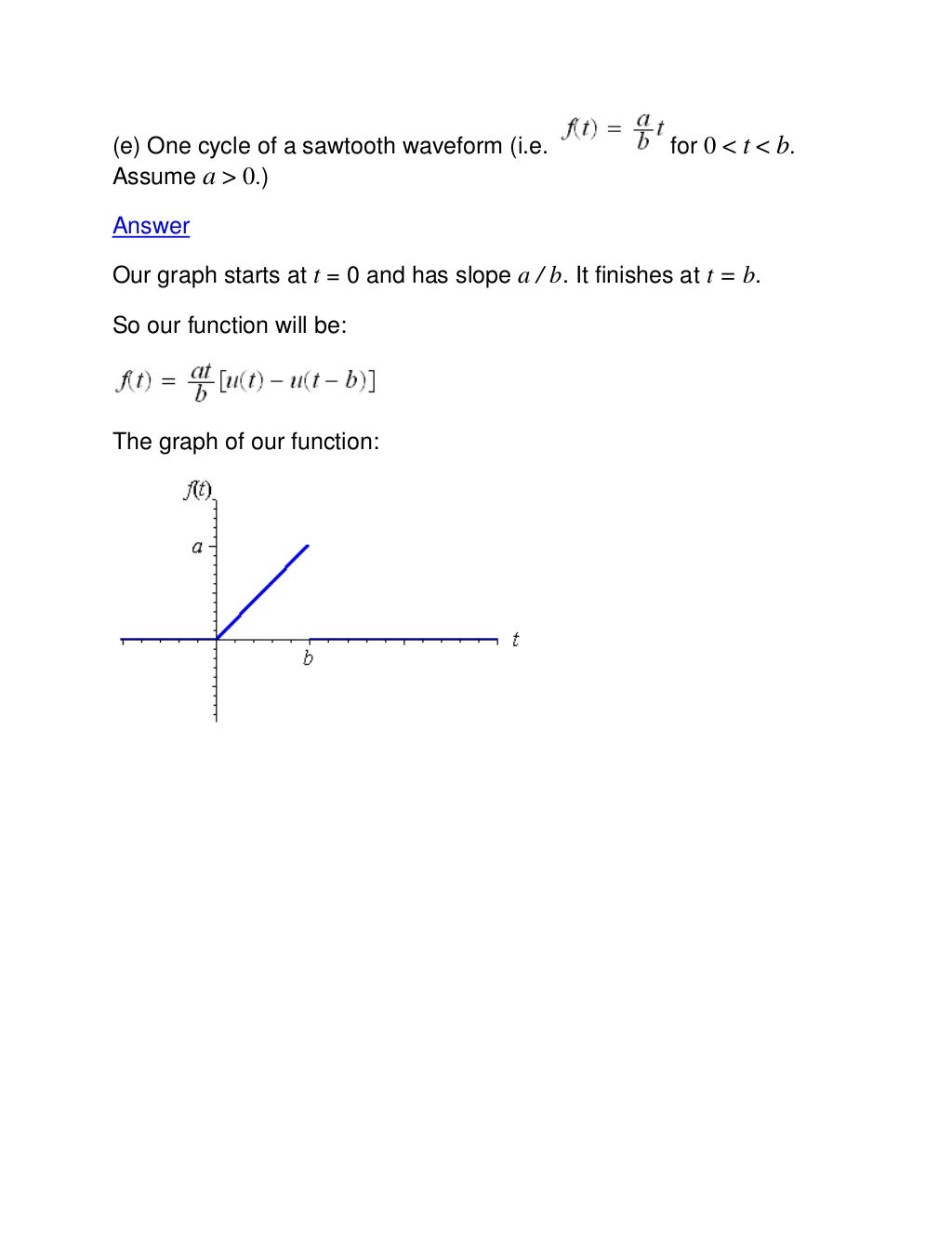
REPRESENTATION OF SIGNALUNIT RAMP FUNCTIONUNIT STEP, 46 OFF
The derivative of unit step $u(t)$ is dirac delta function $\delta(t)$, since an alternative definition of the unit step is using. () which has unit area. Actually, with an appropriate mode of convergence, when a sequence of differentiable functions converge to the unit step, it can be. Now let's look at a signal: Ut %() what is its derivative?
Unit step function
Ut %() what is its derivative? Now let's look at a signal: () which has unit area. Actually, with an appropriate mode of convergence, when a sequence of differentiable functions converge to the unit step, it can be. The derivative of unit step $u(t)$ is dirac delta function $\delta(t)$, since an alternative definition of the unit step is using.
The Derivative Of Unit Step $U(T)$ Is Dirac Delta Function $\Delta(T)$, Since An Alternative Definition Of The Unit Step Is Using.
Where t = 0, the derivative of the unit step. () which has unit area. For this reason, the derivative of the unit step function is 0 at all points t, except where t = 0. Actually, with an appropriate mode of convergence, when a sequence of differentiable functions converge to the unit step, it can be.
Now Let's Look At A Signal:
Just like the unit step function, the function is really an idealized view of nature. In reality, a delta function is nearly a spike near 0 which. The derivative of the unit step function (or heaviside function) is the dirac delta, which is a generalized function (or a. Ut %() what is its derivative?