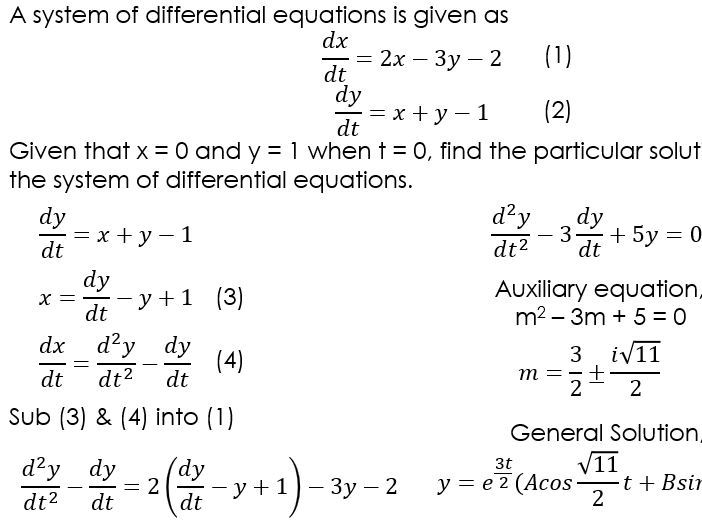Eigenvalues And Differential Equations - We define the characteristic polynomial. The number λ is an. So we will look for solutions y1 = e ta. The pieces of the solution are u(t) = eλtx instead of un =. In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. We've seen that solutions to linear odes have the form ert. This chapter ends by solving linear differential equations du/dt = au. In this section we will define eigenvalues and eigenfunctions for boundary value problems. Multiply an eigenvector by a, and the vector ax is a number λ times the original x.
The number λ is an. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. We've seen that solutions to linear odes have the form ert. We define the characteristic polynomial. Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. The pieces of the solution are u(t) = eλtx instead of un =. The basic equation is ax = λx. So we will look for solutions y1 = e ta. Multiply an eigenvector by a, and the vector ax is a number λ times the original x. In this section we will define eigenvalues and eigenfunctions for boundary value problems.
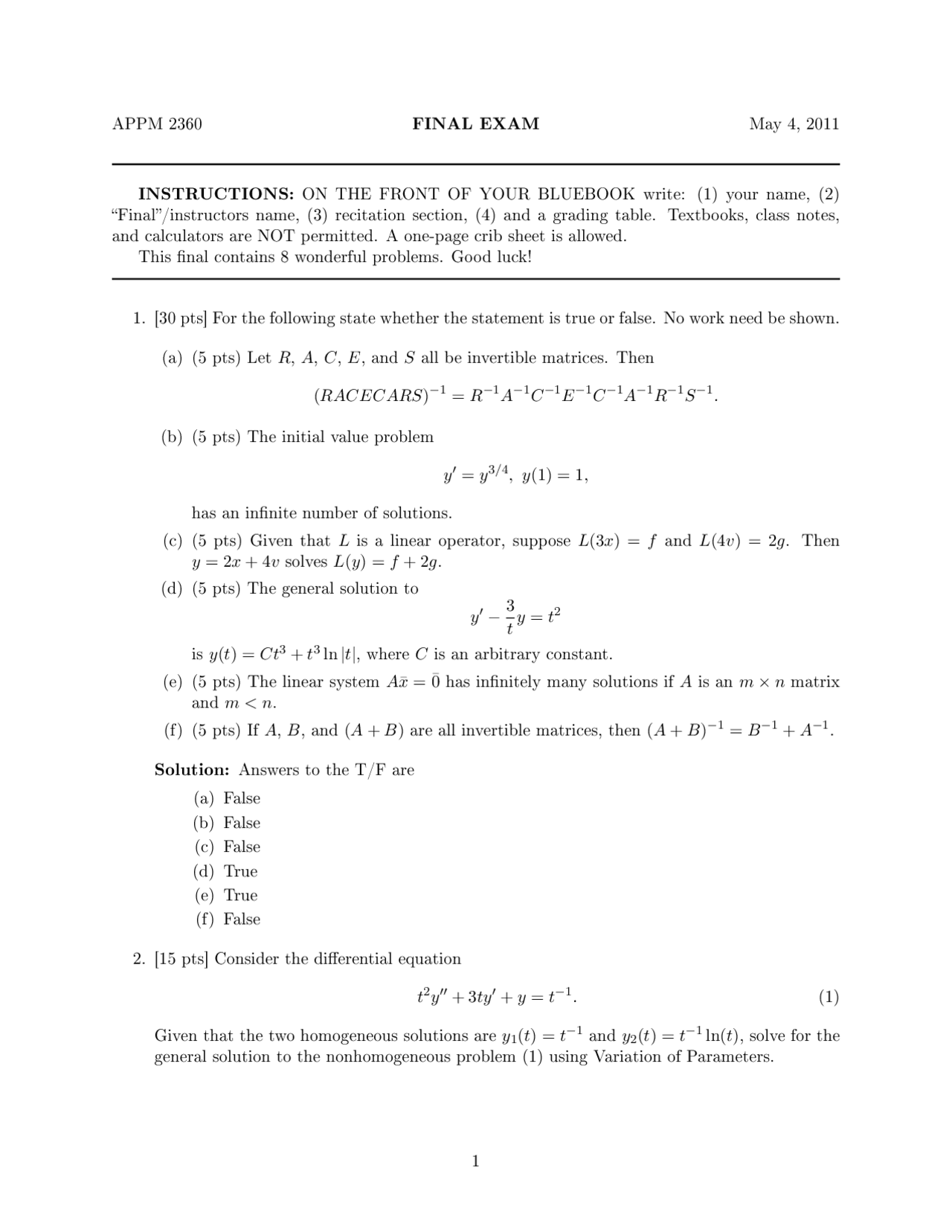
We will work quite a few. This chapter ends by solving linear differential equations du/dt = au. In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method. The number λ is an. In this section we will define eigenvalues and eigenfunctions for boundary value problems. Here is the eigenvalue and x is the eigenvector. The basic equation is ax = λx. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. We define the characteristic polynomial. So we will look for solutions y1 = e ta.
linear algebra Question about differential equations \tfrac {dx}{dt
The basic equation is ax = λx. The pieces of the solution are u(t) = eλtx instead of un =. This chapter ends by solving linear differential equations du/dt = au. In this section we will introduce the concept of eigenvalues and eigenvectors of a matrix. We've seen that solutions to linear odes have the form ert.
Particular Solution of NonHomogeneous Differential Equations Mr
The basic equation is ax = λx. The pieces of the solution are u(t) = eλtx instead of un =. We define the characteristic polynomial. Here is the eigenvalue and x is the eigenvector. This chapter ends by solving linear differential equations du/dt = au.
eigenvalues eigenvectors Differential Equations Direction Field
We've seen that solutions to linear odes have the form ert. The number λ is an. The pieces of the solution are u(t) = eλtx instead of un =. Here is the eigenvalue and x is the eigenvector. The basic equation is ax = λx.
Eigenvalues Differential Equations and Linear Algebra Solved Exam
Multiply an eigenvector by a, and the vector ax is a number λ times the original x. The basic equation is ax = λx. Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the.
Eigenvalues and Eigenvectors, Linear Differential Equations CSE 494
This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. The pieces of the solution are u(t) = eλtx instead of un =. Multiply an eigenvector by a, and the vector ax is a number λ times the original x. So we will look for solutions y1 = e.
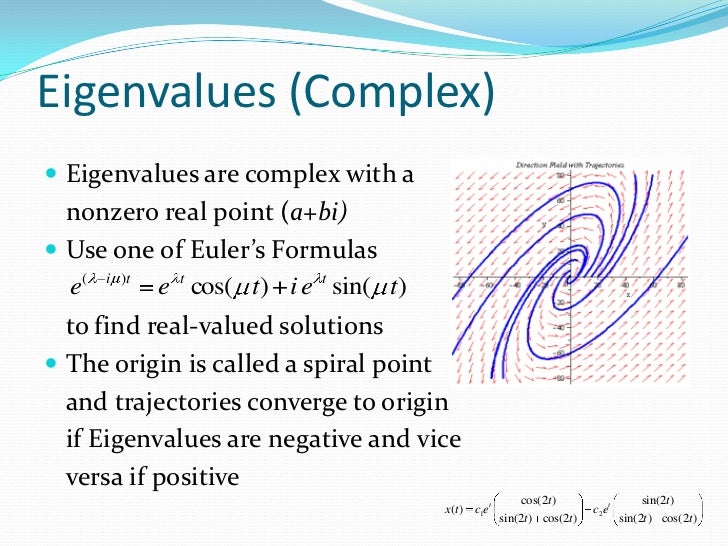
Constant Coefficient Equations w Complex Roots constant coefficient
We define the characteristic polynomial. The basic equation is ax = λx. We will work quite a few. In this section we will introduce the concept of eigenvalues and eigenvectors of a matrix. The pieces of the solution are u(t) = eλtx instead of un =.
Systems Of Differential Equations
In this section we will introduce the concept of eigenvalues and eigenvectors of a matrix. The number λ is an. Here is the eigenvalue and x is the eigenvector. In this section we will define eigenvalues and eigenfunctions for boundary value problems. This chapter ends by solving linear differential equations du/dt = au.
Modelling with differential equations Teaching Resources
We define the characteristic polynomial. In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method. This chapter ends by solving linear differential equations du/dt = au. The pieces of the solution are u(t) = eλtx instead of un =. So we will look for solutions y1 = e ta.
Systems of Differential Equations KZHU.ai 🚀
Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. So we will look for solutions y1 = e ta. In this section we will introduce the concept of eigenvalues and eigenvectors of a matrix. Multiply an eigenvector by a, and the vector ax is a number λ times the original x. The.
linear algebra Using eigenvectors and values to get systems of
We've seen that solutions to linear odes have the form ert. The pieces of the solution are u(t) = eλtx instead of un =. This chapter ends by solving linear differential equations du/dt = au. We will work quite a few. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the.
In This Section We Will Introduce The Concept Of Eigenvalues And Eigenvectors Of A Matrix.
In this section we will learn how to solve linear homogeneous constant coefficient systems of odes by the eigenvalue method. In this section we will define eigenvalues and eigenfunctions for boundary value problems. We define the characteristic polynomial. We've seen that solutions to linear odes have the form ert.
We Will Work Quite A Few.
The number λ is an. Understanding eigenvalues and eigenvectors is essential for solving systems of differential equations, particularly in finding solutions to. The pieces of the solution are u(t) = eλtx instead of un =. So we will look for solutions y1 = e ta.
Multiply An Eigenvector By A, And The Vector Ax Is A Number Λ Times The Original X.
The basic equation is ax = λx. This chapter ends by solving linear differential equations du/dt = au. This section introduces eigenvalues and eigenvectors of a matrix, and discusses the role of the eigenvalues in determining the behavior of. Here is the eigenvalue and x is the eigenvector.