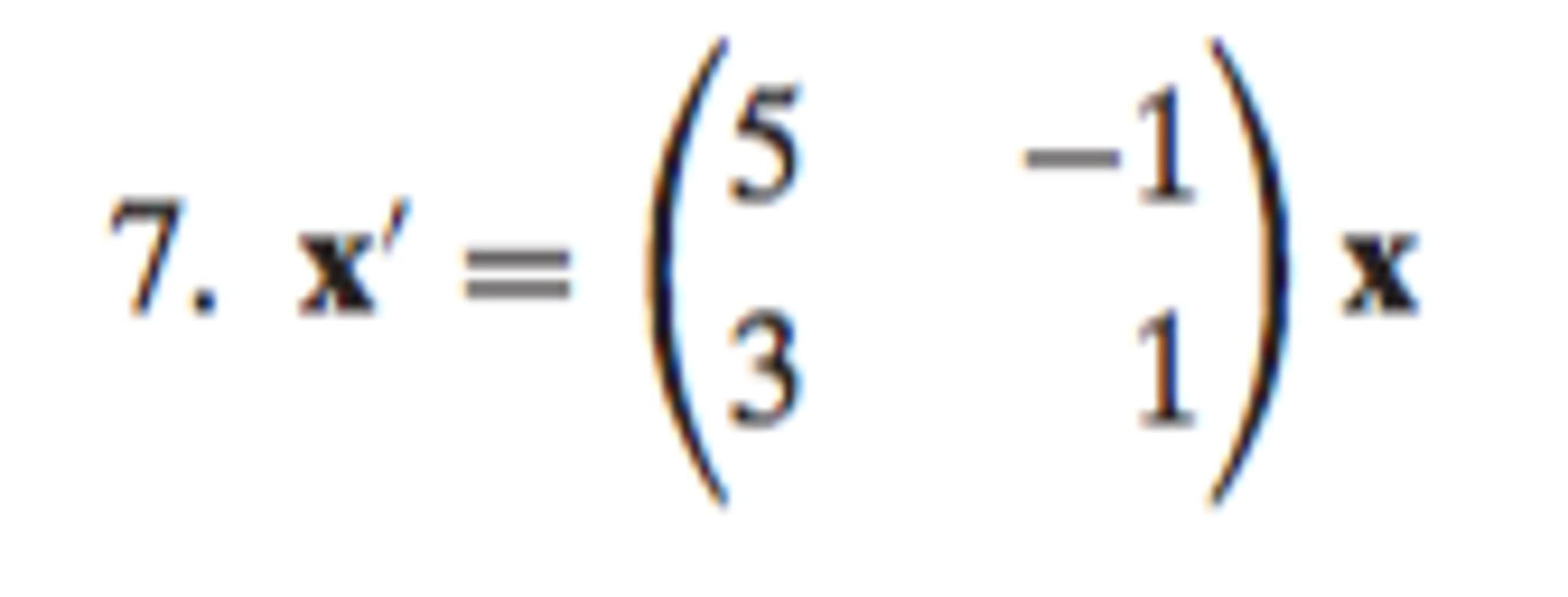Fundamental Matrix Differential Equations - This section is devoted to fundamental matrices for linear differential equations. It is therefore useful to have a. A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a first order differential equation for an unknown. There are many ways to pick two independent solu tions of x = a x to form the columns of φ. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t). The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. As t varies, the point x(t) traces out a curve in rn. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <.
It is therefore useful to have a. A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a first order differential equation for an unknown. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t). Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. There are many ways to pick two independent solu tions of x = a x to form the columns of φ. This section is devoted to fundamental matrices for linear differential equations. As t varies, the point x(t) traces out a curve in rn.
The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t). There are many ways to pick two independent solu tions of x = a x to form the columns of φ. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. It is therefore useful to have a. As t varies, the point x(t) traces out a curve in rn. This section is devoted to fundamental matrices for linear differential equations. A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a first order differential equation for an unknown.
Matrix differential equation Alchetron, the free social encyclopedia
A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a first order differential equation for an unknown. It is therefore useful to have a. There are many ways to pick two independent solu tions of x = a x to form the columns of φ. Fundamental matrix suppose.
(PDF) FourthOrder Approximation of the Fundamental Matrix of Linear
A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a first order differential equation for an unknown. This section is devoted to fundamental matrices for linear differential equations. It is therefore useful to have a. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to.
Fundamental PrinciplesDifferential Equations and Their Solutions
It is therefore useful to have a. A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a first order differential equation for an unknown. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form.
Textbooks Differential Equations Freeup
Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. As t varies, the point x(t) traces out a curve in rn. It is therefore useful to have a. A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ 0 (t) = p(t)ψ(t) (2) note that.
arrays Implement solution of differential equations system using
It is therefore useful to have a. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t). There are many ways to pick two independent solu tions of x = a x to form the columns of φ. A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ 0 (t) =.
Complex Solution and Fundamental MatrixDifferential Equations and
As t varies, the point x(t) traces out a curve in rn. This section is devoted to fundamental matrices for linear differential equations. It is therefore useful to have a. The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ.
(PDF) On graph differential equations and its associated matrix
This section is devoted to fundamental matrices for linear differential equations. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t). Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. The matrix valued function \( x (t) \) is called.
Modelling Motion with Differential Equations
There are many ways to pick two independent solu tions of x = a x to form the columns of φ. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. It is therefore useful to have a. This section is devoted to fundamental matrices for linear differential.
Systems of Matrix Differential Equations for Surfaces
The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. It is therefore useful to have a. There are many ways to pick two independent solu tions of x = a x to form the columns of φ. Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation.
Solved Find a fundamental matrix for the given system of
As t varies, the point x(t) traces out a curve in rn. A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a first order differential equation for an unknown. This section is devoted to fundamental matrices for linear differential equations. It is therefore useful to have a. Fundamental.
As T Varies, The Point X(T) Traces Out A Curve In Rn.
Fundamental matrix suppose that x(1)(t);:::;x(n)(t) form a fundamental set of solutions for the equation x0= p(t)x (1) on some interval <t <. It is therefore useful to have a. There are many ways to pick two independent solu tions of x = a x to form the columns of φ. A fundamental matrix for (1) is any matrix ψ(t) that satisfies ψ 0 (t) = p(t)ψ(t) (2) note that (2) is a first order differential equation for an unknown.
This Section Is Devoted To Fundamental Matrices For Linear Differential Equations.
The matrix valued function \( x (t) \) is called the fundamental matrix, or the fundamental matrix solution. The fundamental matrix φ(t,t0) is a mapping of the initial condition a to the solution vector x(t).



/aae14611c6e6e45b3bd99783f827f01c.jpg)