Homogeneous Vs Nonhomogeneous Differential Equation - The terminology and methods are different from. The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: This is another way of classifying differential equations. In this section, we examine how to solve nonhomogeneous differential equations. That is, if no term is a. If \( f(x) = 0 \), then it is called a homogeneous equation. A linear equation may further be called homogeneous if all terms depend on the dependent variable.
The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: That is, if no term is a. The terminology and methods are different from. If \( f(x) = 0 \), then it is called a homogeneous equation. This is another way of classifying differential equations. A linear equation may further be called homogeneous if all terms depend on the dependent variable. In this section, we examine how to solve nonhomogeneous differential equations.
The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: That is, if no term is a. If \( f(x) = 0 \), then it is called a homogeneous equation. A linear equation may further be called homogeneous if all terms depend on the dependent variable. The terminology and methods are different from. In this section, we examine how to solve nonhomogeneous differential equations. This is another way of classifying differential equations.
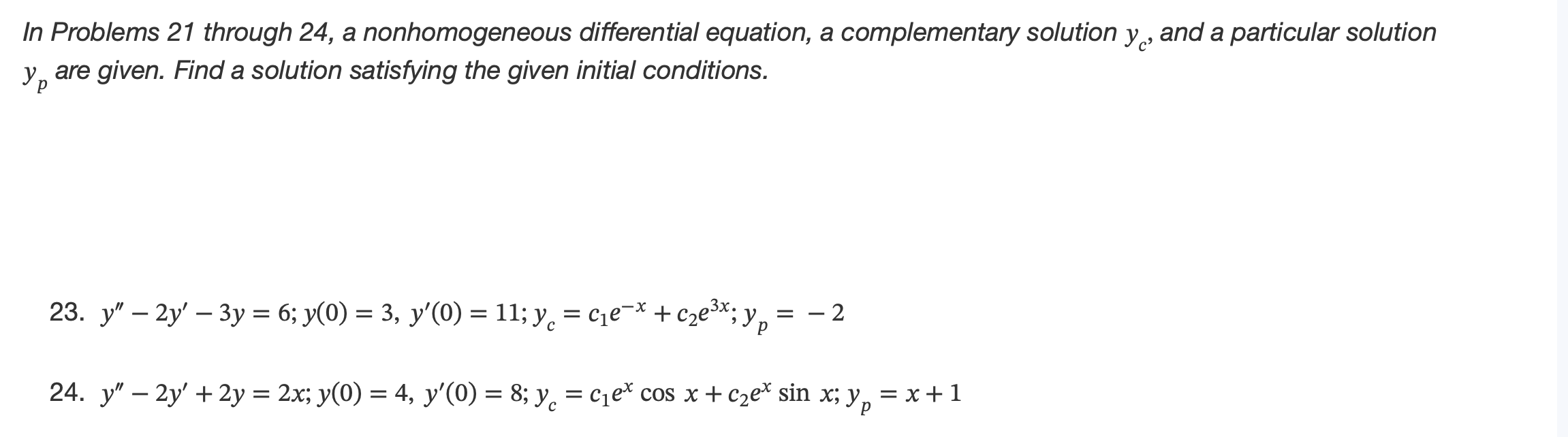
Solved In Problems 21 through 24, a nonhomogeneous
In this section, we examine how to solve nonhomogeneous differential equations. If \( f(x) = 0 \), then it is called a homogeneous equation. That is, if no term is a. The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: The terminology and methods are different from.
[Solved] A nonhomogeneous differential equation, a complementary
The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following: If \( f(x) = 0 \), then it is called a homogeneous equation. In this section, we examine how to solve nonhomogeneous differential equations. This is another way of classifying differential equations. The terminology and methods are different from.
Solving a nonhomogeneous equation
The terminology and methods are different from. This is another way of classifying differential equations. That is, if no term is a. If \( f(x) = 0 \), then it is called a homogeneous equation. In this section, we examine how to solve nonhomogeneous differential equations.
The homogeneous differential equation (Equation (16)) has non trivial
This is another way of classifying differential equations. A linear equation may further be called homogeneous if all terms depend on the dependent variable. In this section, we examine how to solve nonhomogeneous differential equations. The terminology and methods are different from. That is, if no term is a.
[Solved] Find the general solution to the nonhomogeneous differential
The terminology and methods are different from. If \( f(x) = 0 \), then it is called a homogeneous equation. That is, if no term is a. This is another way of classifying differential equations. The simplest test of homogeneity, and definition at the same time, not only for differential equations, is the following:
[Differential Equations] NonHomogeneous Equation Solution r/learnmath
If \( f(x) = 0 \), then it is called a homogeneous equation. This is another way of classifying differential equations. In this section, we examine how to solve nonhomogeneous differential equations. That is, if no term is a. The terminology and methods are different from.
Particular Solution of NonHomogeneous Differential Equations Mr
In this section, we examine how to solve nonhomogeneous differential equations. If \( f(x) = 0 \), then it is called a homogeneous equation. The terminology and methods are different from. A linear equation may further be called homogeneous if all terms depend on the dependent variable. That is, if no term is a.
Differential Equations Lecture NonHomogeneous Linear Differential E…
This is another way of classifying differential equations. If \( f(x) = 0 \), then it is called a homogeneous equation. A linear equation may further be called homogeneous if all terms depend on the dependent variable. In this section, we examine how to solve nonhomogeneous differential equations. The terminology and methods are different from.
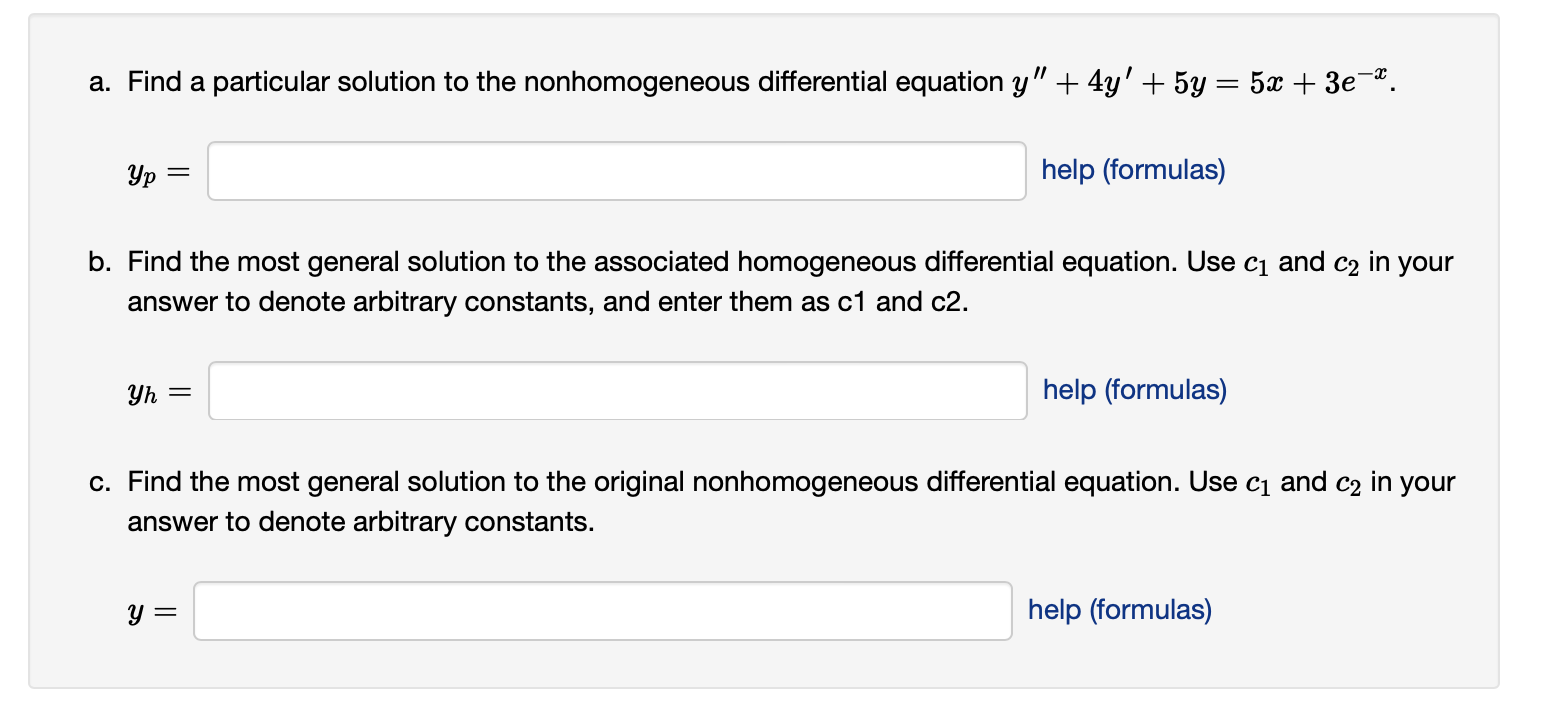
Solved a. Find a particular solution to the nonhomogeneous
In this section, we examine how to solve nonhomogeneous differential equations. If \( f(x) = 0 \), then it is called a homogeneous equation. A linear equation may further be called homogeneous if all terms depend on the dependent variable. This is another way of classifying differential equations. The terminology and methods are different from.
PPT Homogeneous Differential Equation NonHomogeneous Differential
A linear equation may further be called homogeneous if all terms depend on the dependent variable. In this section, we examine how to solve nonhomogeneous differential equations. The terminology and methods are different from. If \( f(x) = 0 \), then it is called a homogeneous equation. That is, if no term is a.
The Simplest Test Of Homogeneity, And Definition At The Same Time, Not Only For Differential Equations, Is The Following:
A linear equation may further be called homogeneous if all terms depend on the dependent variable. This is another way of classifying differential equations. That is, if no term is a. If \( f(x) = 0 \), then it is called a homogeneous equation.
In This Section, We Examine How To Solve Nonhomogeneous Differential Equations.
The terminology and methods are different from.
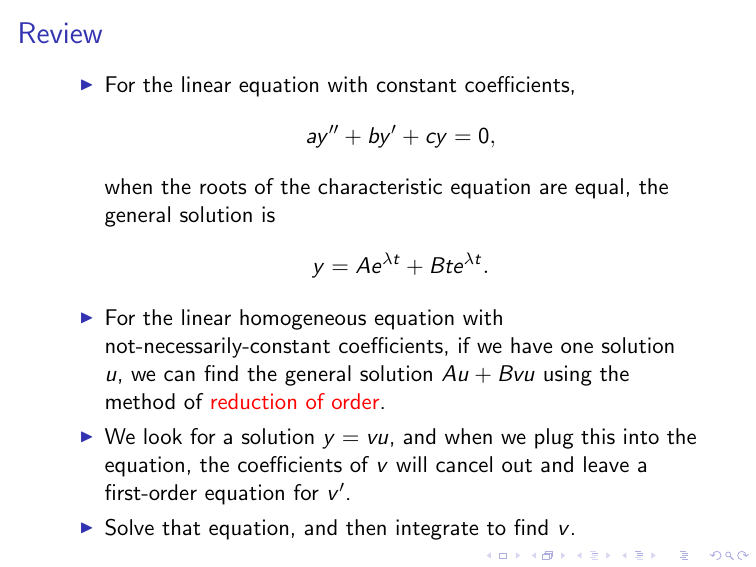

![[Differential Equations] NonHomogeneous Equation Solution r/learnmath](https://i.imgur.com/6lFKSuI.png)