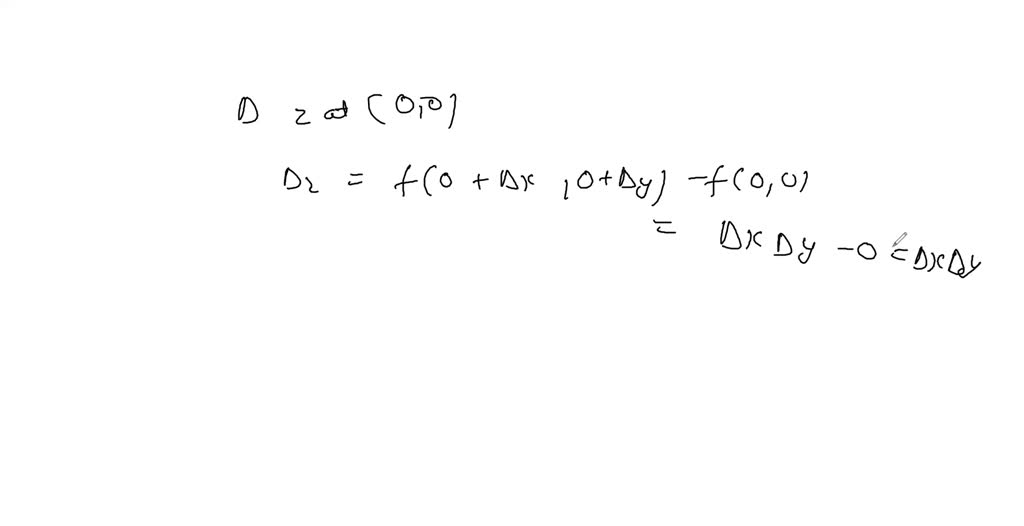How To Prove Differentiability Of A Function - To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. Determining the differentiability of a function involves assessing whether the function meets. To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit.
Determining the differentiability of a function involves assessing whether the function meets. To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit. To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$.
To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. Determining the differentiability of a function involves assessing whether the function meets. To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit.
real analysis To prove differentiability of a function at a point
To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit. Determining the differentiability of a function involves assessing whether the function meets.
SOLVEDUse the definition of differentiability to prove that the
To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit. Determining the differentiability of a function involves assessing whether the function meets.
if FX is differentiable at X equal to a prove that it will be
Determining the differentiability of a function involves assessing whether the function meets. To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit.
calculus Differentiability of a piecewise function confusion about
To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. Determining the differentiability of a function involves assessing whether the function meets. To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit.
PPT 3.2 Differentiability PowerPoint Presentation, free download ID
To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. Determining the differentiability of a function involves assessing whether the function meets. To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit.
calculus Need some help to understand differentiability of function
To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit. Determining the differentiability of a function involves assessing whether the function meets.
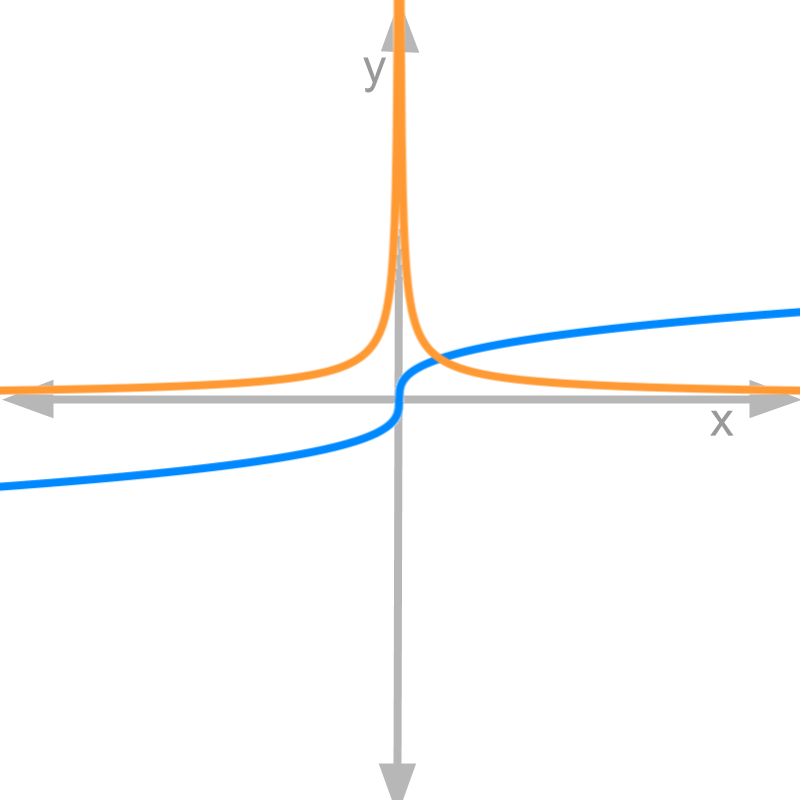
derivatives Differentiability Implies Continuity (Multivariable
To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit. Determining the differentiability of a function involves assessing whether the function meets.
calculus The Differentiability of a Piecewise Function Mathematics
To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit. To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. Determining the differentiability of a function involves assessing whether the function meets.
Differential Calculus Differentiability of Functions
To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit. To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. Determining the differentiability of a function involves assessing whether the function meets.
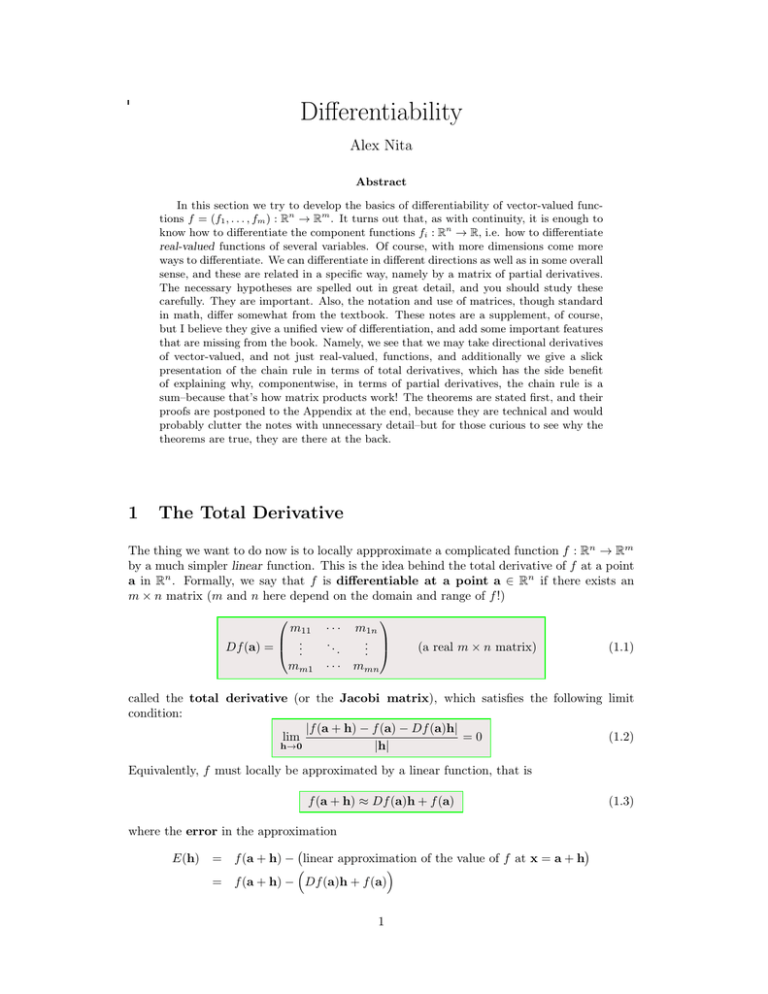
Differentiability
To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit. To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$. Determining the differentiability of a function involves assessing whether the function meets.
Determining The Differentiability Of A Function Involves Assessing Whether The Function Meets.
To prove that a function is differentiable at a point $x \in \mathbb{r}$ we must prove that the limit. To show that $f$ is differentiable at all $x \in \bbb r$, we must show that $f'(x)$.