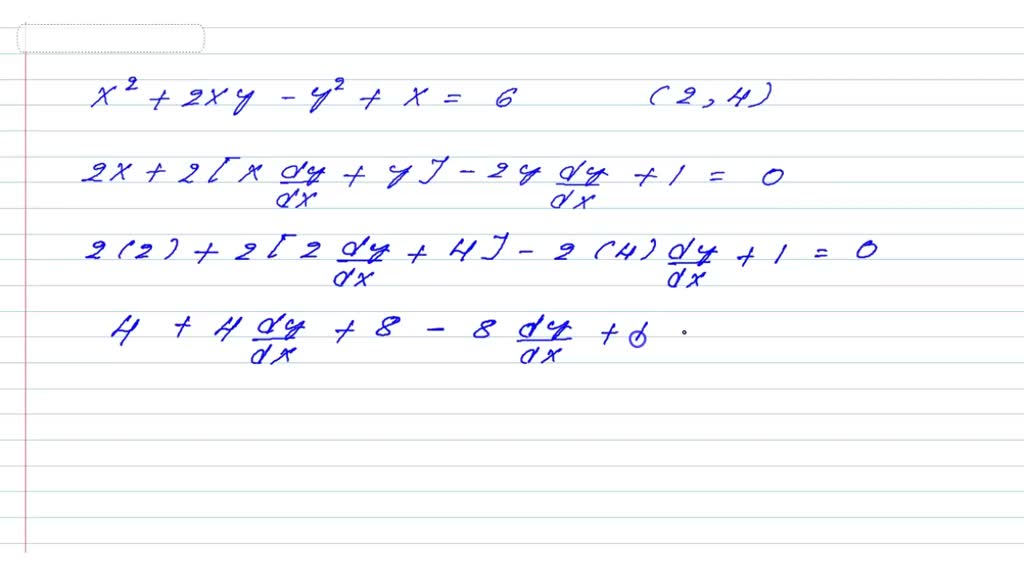Implicit Differentiation Trigonometric - An important application of implicit differentiation is to finding the derivatives of inverse. Here is a set of practice problems to accompany the implicit differentiation. Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x).
Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x). Here is a set of practice problems to accompany the implicit differentiation. An important application of implicit differentiation is to finding the derivatives of inverse.
Here is a set of practice problems to accompany the implicit differentiation. An important application of implicit differentiation is to finding the derivatives of inverse. Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x).
SOLUTION Implicit differentiation inverse trigonometric functions
Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x). An important application of implicit differentiation is to finding the derivatives of inverse. Here is a set of practice problems to accompany the implicit differentiation.
Implicit Differentiation PDF Mathematical Analysis Differential
Here is a set of practice problems to accompany the implicit differentiation. An important application of implicit differentiation is to finding the derivatives of inverse. Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x).
intro to Implicit differentiation PDF Free Download
An important application of implicit differentiation is to finding the derivatives of inverse. Here is a set of practice problems to accompany the implicit differentiation. Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x).
SOLVED Use implicit differentiation to find an equation of the tangent
Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x). Here is a set of practice problems to accompany the implicit differentiation. An important application of implicit differentiation is to finding the derivatives of inverse.
Section 3.5 Implicit Differentiation 1 Implicit Differentiation 2 3
Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x). Here is a set of practice problems to accompany the implicit differentiation. An important application of implicit differentiation is to finding the derivatives of inverse.
Implicit Differentiation For Inverse Trigonometric Functions PDF
Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x). An important application of implicit differentiation is to finding the derivatives of inverse. Here is a set of practice problems to accompany the implicit differentiation.
SOLUTION Implicit differentiation for inverse trigonometric functions
Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x). An important application of implicit differentiation is to finding the derivatives of inverse. Here is a set of practice problems to accompany the implicit differentiation.
Implicit Differentiation Practice Quiz
Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x). An important application of implicit differentiation is to finding the derivatives of inverse. Here is a set of practice problems to accompany the implicit differentiation.
SOLUTION Lesson 1 implicit differentiation Studypool
An important application of implicit differentiation is to finding the derivatives of inverse. Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x). Here is a set of practice problems to accompany the implicit differentiation.
22 Differentiation (Implicit) PDF Trigonometric Functions Derivative
An important application of implicit differentiation is to finding the derivatives of inverse. Here is a set of practice problems to accompany the implicit differentiation. Once we rewrite f′(y) f ′ (y) in terms of x x, we have the derivative of f−1(x) f − 1 (x).
Once We Rewrite F′(Y) F ′ (Y) In Terms Of X X, We Have The Derivative Of F−1(X) F − 1 (X).
An important application of implicit differentiation is to finding the derivatives of inverse. Here is a set of practice problems to accompany the implicit differentiation.