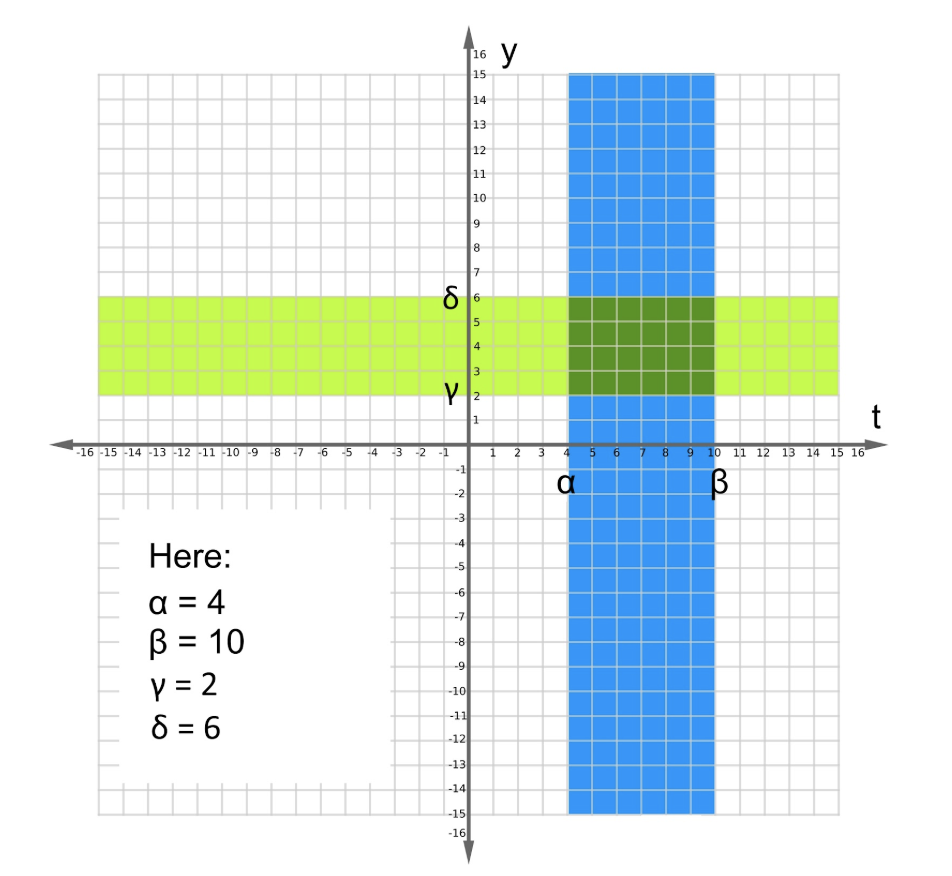
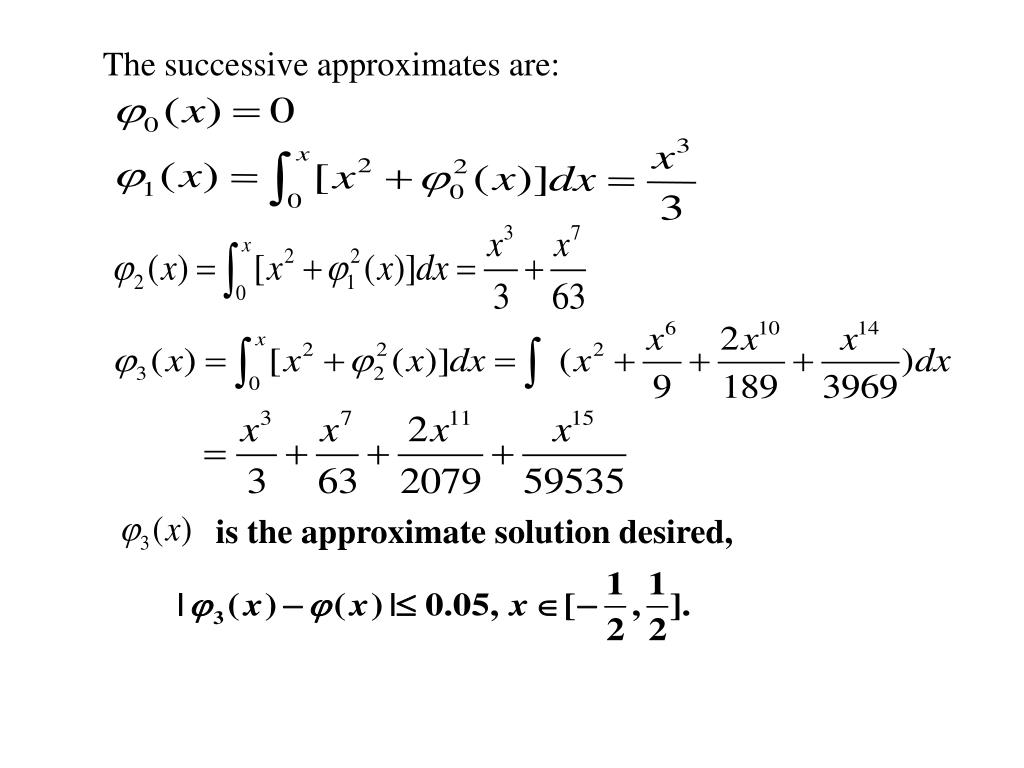
Interval Of Existence Differential Equations - $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =. The interval of existence is thus ( 1;2): In this chapter we introduce the notion of an initial value problem (ivp) for first order systems of. Intervals of existence of solutions of. We want to find an interval on which a solution surely exists. In this section we will give an in depth look at intervals of validity as well as an. The general solution is the same for any initial. I have a really simple differential equation: Find the maximal interval of existence of the solution. Here our function f is defined by.
In this section we will give an in depth look at intervals of validity as well as an. Intervals of existence of solutions of. The general solution is the same for any initial. Find the maximal interval of existence of the solution. We want to find an interval on which a solution surely exists. The interval of existence is thus ( 1;2): I have a really simple differential equation: Here our function f is defined by. In this chapter we introduce the notion of an initial value problem (ivp) for first order systems of. $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =.
We want to find an interval on which a solution surely exists. I have a really simple differential equation: $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =. The general solution is the same for any initial. Find the maximal interval of existence of the solution. The interval of existence is thus ( 1;2): Here our function f is defined by. Intervals of existence of solutions of. In this section we will give an in depth look at intervals of validity as well as an. In this chapter we introduce the notion of an initial value problem (ivp) for first order systems of.
(PDF) Existence of extremal solutions to intervalvalued delay
Intervals of existence of solutions of. $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =. The general solution is the same for any initial. Find the maximal interval of existence of the solution. In this section we will give an in depth look at intervals of validity as well as an.
Interval of validity StudyPug
In this section we will give an in depth look at intervals of validity as well as an. $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =. Find the maximal interval of existence of the solution. Intervals of existence of solutions of. The interval of existence is thus ( 1;2):
(PDF) Some Existence and Uniqueness Results for a Class of Fractional
In this chapter we introduce the notion of an initial value problem (ivp) for first order systems of. The general solution is the same for any initial. We want to find an interval on which a solution surely exists. Intervals of existence of solutions of. I have a really simple differential equation:
(PDF) Existence of Periodic Solutions to QuaternionValued Impulsive
In this section we will give an in depth look at intervals of validity as well as an. The general solution is the same for any initial. In this chapter we introduce the notion of an initial value problem (ivp) for first order systems of. The interval of existence is thus ( 1;2): Find the maximal interval of existence of.
(PDF) Global existence of solutions for intervalvalued secondorder
In this section we will give an in depth look at intervals of validity as well as an. Find the maximal interval of existence of the solution. I have a really simple differential equation: The interval of existence is thus ( 1;2): In this chapter we introduce the notion of an initial value problem (ivp) for first order systems of.
(PDF) Existence and Uniqueness of Solution for a Class of Stochastic
Intervals of existence of solutions of. $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =. I have a really simple differential equation: Here our function f is defined by. In this section we will give an in depth look at intervals of validity as well as an.
PPT Chapter 2 Theory of First Order Differential Equations PowerPoint
Intervals of existence of solutions of. In this section we will give an in depth look at intervals of validity as well as an. We want to find an interval on which a solution surely exists. $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =. In this chapter we introduce the notion of an initial value problem (ivp) for first order.
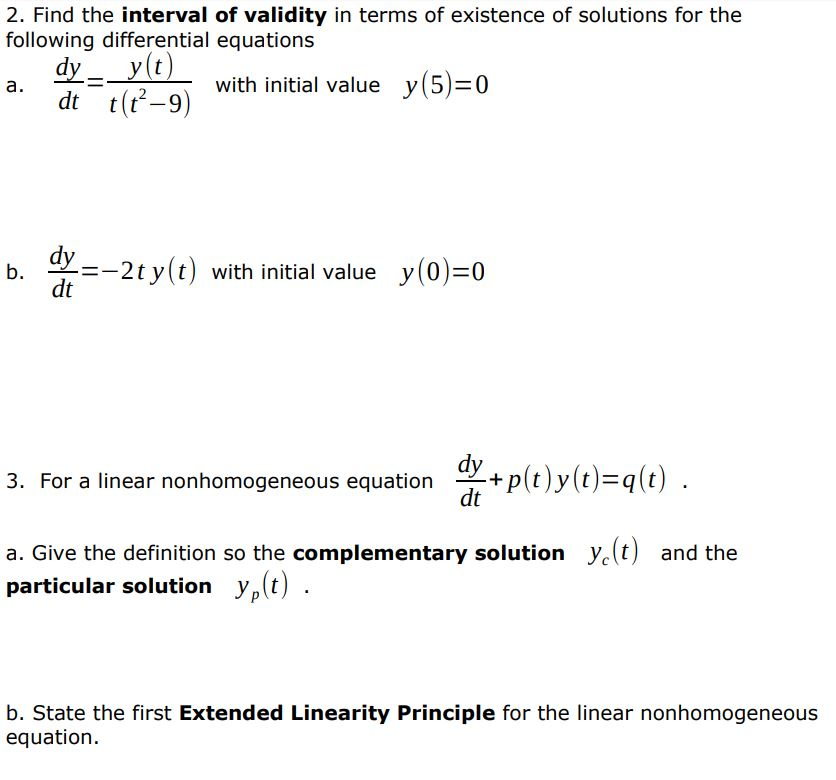
Solved Find the interval of validity in terms of existence
The interval of existence is thus ( 1;2): We want to find an interval on which a solution surely exists. $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =. The general solution is the same for any initial. Here our function f is defined by.
SOLVED Fundamental Existence Theorem for Linear Differential Equations
We want to find an interval on which a solution surely exists. In this chapter we introduce the notion of an initial value problem (ivp) for first order systems of. $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =. Find the maximal interval of existence of the solution. Intervals of existence of solutions of.
(PDF) Global existence and uniqueness of the solution to the impulsive
Intervals of existence of solutions of. Find the maximal interval of existence of the solution. $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =. In this chapter we introduce the notion of an initial value problem (ivp) for first order systems of. We want to find an interval on which a solution surely exists.
In This Chapter We Introduce The Notion Of An Initial Value Problem (Ivp) For First Order Systems Of.
Find the maximal interval of existence of the solution. Intervals of existence of solutions of. Here our function f is defined by. $\frac{dx}{dt} = x^2.t$ with initial value $x(0) =.
I Have A Really Simple Differential Equation:
The general solution is the same for any initial. The interval of existence is thus ( 1;2): In this section we will give an in depth look at intervals of validity as well as an. We want to find an interval on which a solution surely exists.