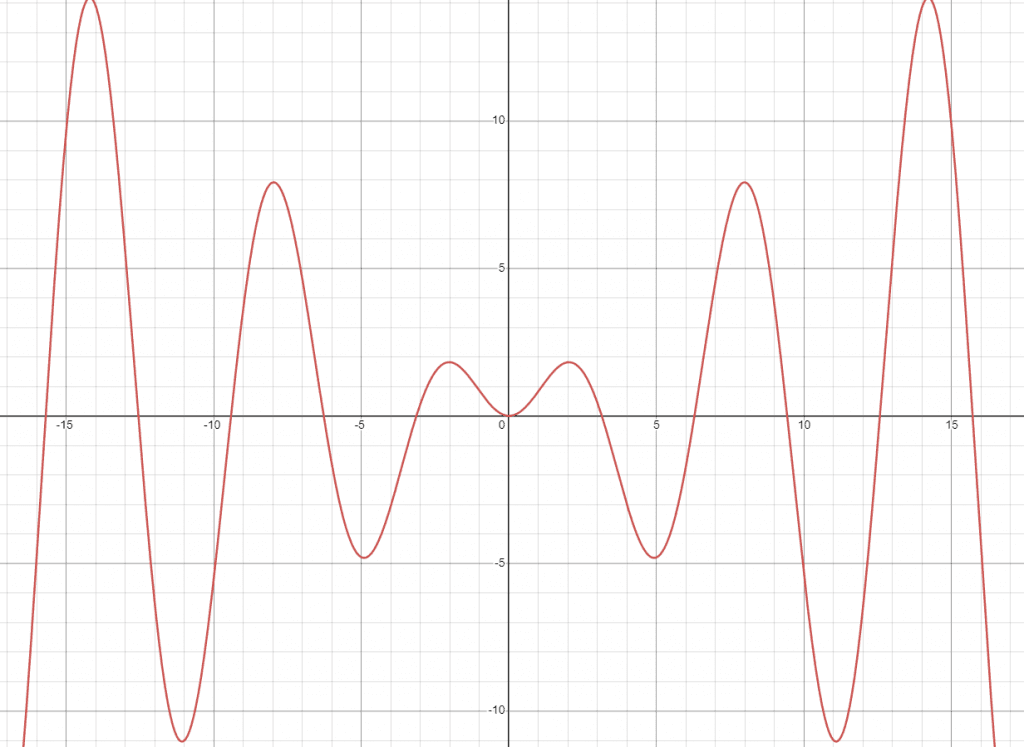
Linear Vs Nonlinear Differential Equations - A differential equation is linear if and only if it is in the following form or is mathematically equivalent to said form: We explain the distinction between linear and nonlinear differential equations and why it matters. This can be rewritten as | y | = ex + ln | x | + c = exeln | x | ec = c | x | ex = cxex. A (x)*y + b (x)*y' + c (x)*y'' +. In this section we compare the answers to the two main questions in differential equations for linear and nonlinear first order differential equations. Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x + ln | x | + c. A first order differential equation is said to be linear if it is a linear combination of terms. Linear just means that the variable in an equation appears only with a power of one.
Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x + ln | x | + c. Linear just means that the variable in an equation appears only with a power of one. A first order differential equation is said to be linear if it is a linear combination of terms. We explain the distinction between linear and nonlinear differential equations and why it matters. A (x)*y + b (x)*y' + c (x)*y'' +. This can be rewritten as | y | = ex + ln | x | + c = exeln | x | ec = c | x | ex = cxex. A differential equation is linear if and only if it is in the following form or is mathematically equivalent to said form: In this section we compare the answers to the two main questions in differential equations for linear and nonlinear first order differential equations.
A differential equation is linear if and only if it is in the following form or is mathematically equivalent to said form: Linear just means that the variable in an equation appears only with a power of one. In this section we compare the answers to the two main questions in differential equations for linear and nonlinear first order differential equations. This can be rewritten as | y | = ex + ln | x | + c = exeln | x | ec = c | x | ex = cxex. Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x + ln | x | + c. We explain the distinction between linear and nonlinear differential equations and why it matters. A first order differential equation is said to be linear if it is a linear combination of terms. A (x)*y + b (x)*y' + c (x)*y'' +.
Linear vs Function Explanation and Examples The Story of
We explain the distinction between linear and nonlinear differential equations and why it matters. A differential equation is linear if and only if it is in the following form or is mathematically equivalent to said form: A first order differential equation is said to be linear if it is a linear combination of terms. In this section we compare the.
Linear vs Function Explanation and Examples The Story of
This can be rewritten as | y | = ex + ln | x | + c = exeln | x | ec = c | x | ex = cxex. A first order differential equation is said to be linear if it is a linear combination of terms. We explain the distinction between linear and nonlinear differential equations and.
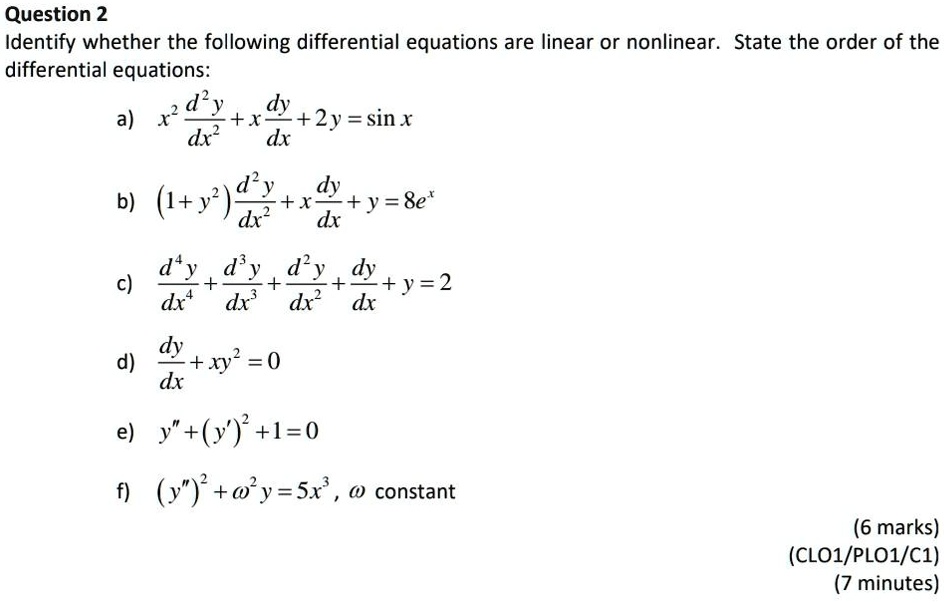
SOLVEDQuestion 2 Identify whether the following differential equations
A (x)*y + b (x)*y' + c (x)*y'' +. Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x + ln | x | + c. A differential equation is linear if and only if it is in the following form or is mathematically.
Solved a Determine whether the following secondorder
A differential equation is linear if and only if it is in the following form or is mathematically equivalent to said form: We explain the distinction between linear and nonlinear differential equations and why it matters. This can be rewritten as | y | = ex + ln | x | + c = exeln | x | ec =.
Linear Vs Differential Ppt Powerpoint Presentation File
Linear just means that the variable in an equation appears only with a power of one. A differential equation is linear if and only if it is in the following form or is mathematically equivalent to said form: In this section we compare the answers to the two main questions in differential equations for linear and nonlinear first order differential.
SOLUTION linear and non linear differential equation examples
This can be rewritten as | y | = ex + ln | x | + c = exeln | x | ec = c | x | ex = cxex. A first order differential equation is said to be linear if it is a linear combination of terms. Linear just means that the variable in an equation appears only.
Linear And Functions Handout Worksheet Worksheets Library
A first order differential equation is said to be linear if it is a linear combination of terms. A (x)*y + b (x)*y' + c (x)*y'' +. This can be rewritten as | y | = ex + ln | x | + c = exeln | x | ec = c | x | ex = cxex. Using the.
Solved Classify each differential equations as linear or
Linear just means that the variable in an equation appears only with a power of one. In this section we compare the answers to the two main questions in differential equations for linear and nonlinear first order differential equations. A differential equation is linear if and only if it is in the following form or is mathematically equivalent to said.
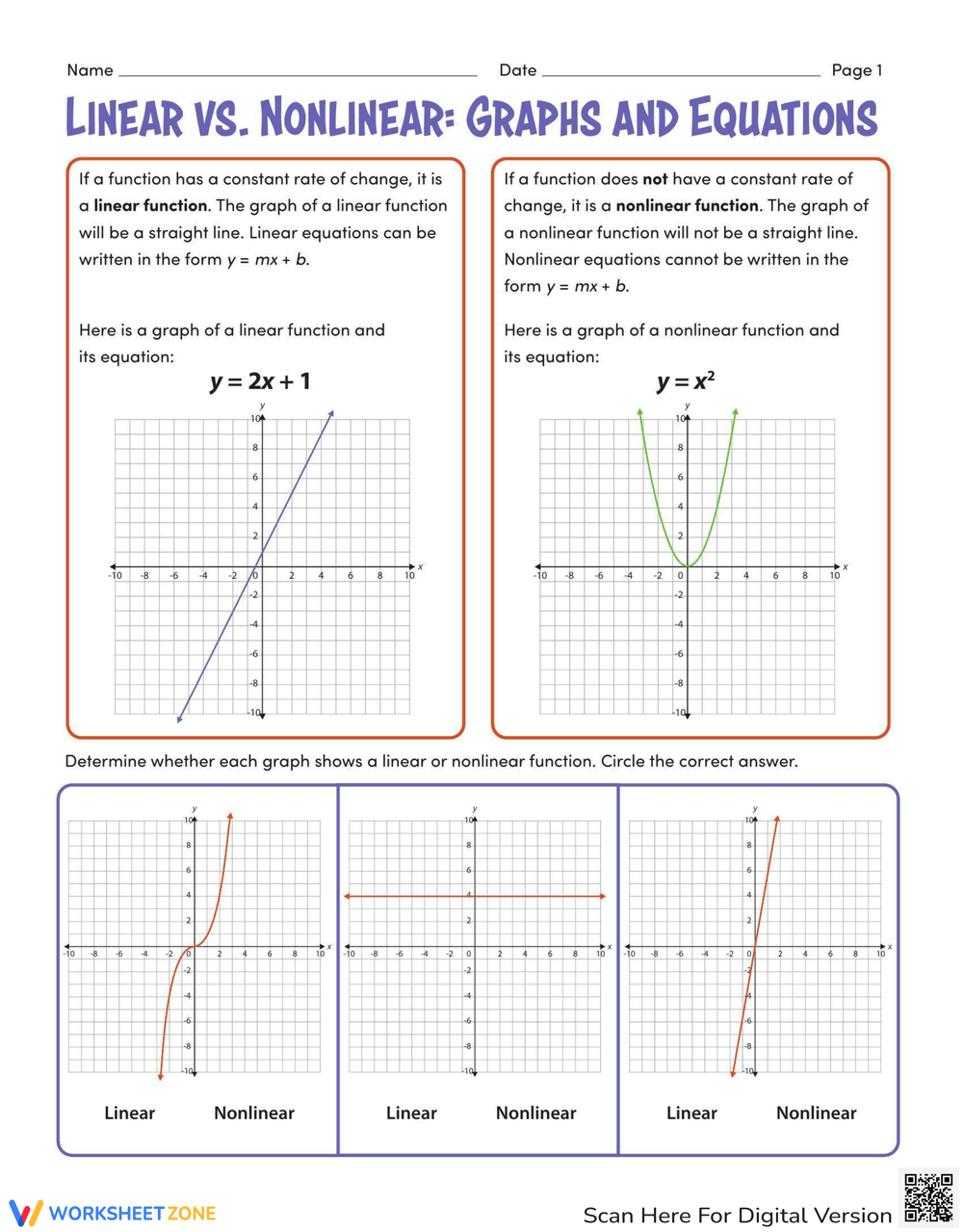
Linear Vs. Graphs And Equations Worksheet
We explain the distinction between linear and nonlinear differential equations and why it matters. A differential equation is linear if and only if it is in the following form or is mathematically equivalent to said form: Linear just means that the variable in an equation appears only with a power of one. In this section we compare the answers to.
Differential Equations Owlcation
In this section we compare the answers to the two main questions in differential equations for linear and nonlinear first order differential equations. A (x)*y + b (x)*y' + c (x)*y'' +. A first order differential equation is said to be linear if it is a linear combination of terms. Using the separable method we have ∫ dy y =.
Linear Just Means That The Variable In An Equation Appears Only With A Power Of One.
A differential equation is linear if and only if it is in the following form or is mathematically equivalent to said form: We explain the distinction between linear and nonlinear differential equations and why it matters. In this section we compare the answers to the two main questions in differential equations for linear and nonlinear first order differential equations. A (x)*y + b (x)*y' + c (x)*y'' +.
This Can Be Rewritten As | Y | = Ex + Ln | X | + C = Exeln | X | Ec = C | X | Ex = Cxex.
Using the separable method we have ∫ dy y = ∫ (1 + 1 x)dx, then after integrating we have ln | y | = x + ln | x | + c. A first order differential equation is said to be linear if it is a linear combination of terms.