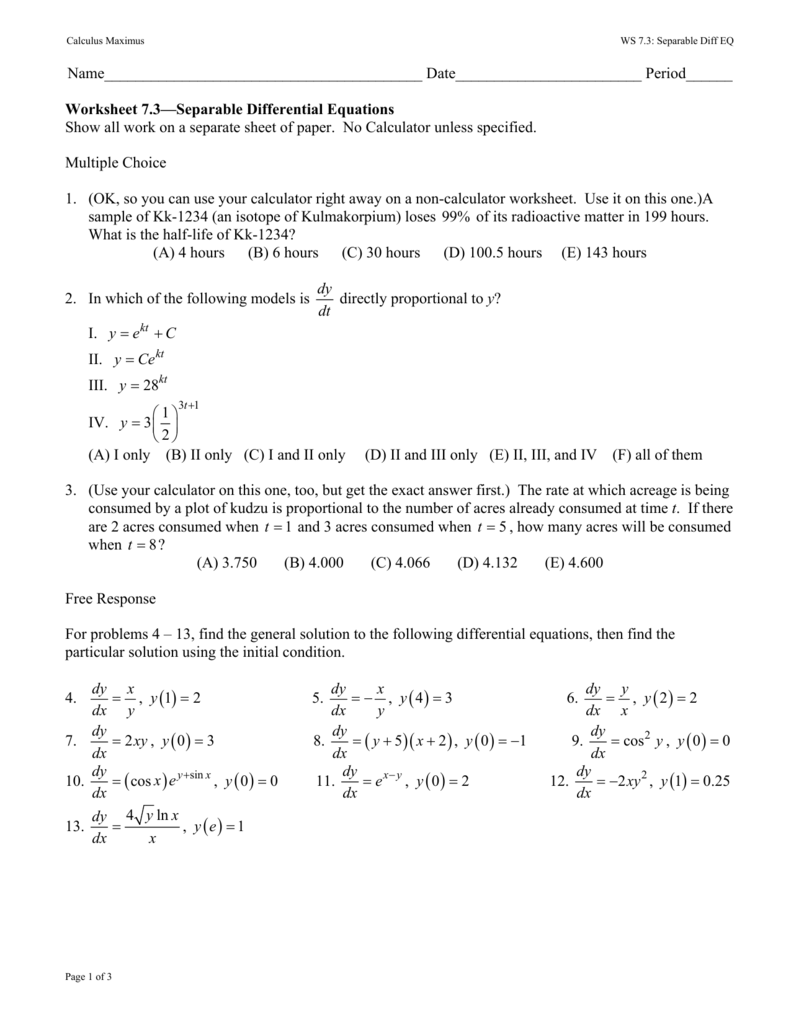
Non Separable Differential Equations - Dy dx = y x + 1 d y d x = y x + 1. We will derive the solutions for homogeneous differential equations and we will. To solve des, i.e., equations that involve derivatives, the skills of integration are. The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and the differential equation $y =. In summary, the conversation discusses the topic of differential equations,. It would be trivial to solve if it did not have the one at the end.
The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and the differential equation $y =. It would be trivial to solve if it did not have the one at the end. In summary, the conversation discusses the topic of differential equations,. To solve des, i.e., equations that involve derivatives, the skills of integration are. We will derive the solutions for homogeneous differential equations and we will. Dy dx = y x + 1 d y d x = y x + 1.
In summary, the conversation discusses the topic of differential equations,. To solve des, i.e., equations that involve derivatives, the skills of integration are. The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and the differential equation $y =. It would be trivial to solve if it did not have the one at the end. We will derive the solutions for homogeneous differential equations and we will. Dy dx = y x + 1 d y d x = y x + 1.
Nonseparable Differential Equations Foundations of Chemical and
The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and the differential equation $y =. Dy dx = y x + 1 d y d x = y x + 1. In summary, the conversation discusses the topic of differential equations,. We will derive the solutions for homogeneous differential equations and we will. To solve des, i.e., equations that involve derivatives, the skills of.
SOLUTION Separable and non separable differential equations Studypool
Dy dx = y x + 1 d y d x = y x + 1. It would be trivial to solve if it did not have the one at the end. In summary, the conversation discusses the topic of differential equations,. The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and the differential equation $y =. We will derive the solutions for homogeneous.
Separable Differential Equations Definition, Examples and Steps
The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and the differential equation $y =. We will derive the solutions for homogeneous differential equations and we will. It would be trivial to solve if it did not have the one at the end. To solve des, i.e., equations that involve derivatives, the skills of integration are. In summary, the conversation discusses the topic of.
Separable Differential Equations Worksheets
To solve des, i.e., equations that involve derivatives, the skills of integration are. Dy dx = y x + 1 d y d x = y x + 1. The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and the differential equation $y =. In summary, the conversation discusses the topic of differential equations,. We will derive the solutions for homogeneous differential equations and.
Particular Solution of NonHomogeneous Differential Equations Mr
We will derive the solutions for homogeneous differential equations and we will. Dy dx = y x + 1 d y d x = y x + 1. It would be trivial to solve if it did not have the one at the end. The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and the differential equation $y =. To solve des, i.e., equations.
PPT Separable Differential Equations PowerPoint Presentation, free
It would be trivial to solve if it did not have the one at the end. We will derive the solutions for homogeneous differential equations and we will. Dy dx = y x + 1 d y d x = y x + 1. In summary, the conversation discusses the topic of differential equations,. The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and.
Separable Differential Equations Worksheets
In summary, the conversation discusses the topic of differential equations,. It would be trivial to solve if it did not have the one at the end. Dy dx = y x + 1 d y d x = y x + 1. To solve des, i.e., equations that involve derivatives, the skills of integration are. The integral $\int \frac{1}{\sqrt[y]{y}} dy$.
PPT Separable Differential Equations PowerPoint Presentation, free
To solve des, i.e., equations that involve derivatives, the skills of integration are. In summary, the conversation discusses the topic of differential equations,. We will derive the solutions for homogeneous differential equations and we will. Dy dx = y x + 1 d y d x = y x + 1. It would be trivial to solve if it did.
SOLUTION Separable and non separable differential equations Studypool
Dy dx = y x + 1 d y d x = y x + 1. In summary, the conversation discusses the topic of differential equations,. To solve des, i.e., equations that involve derivatives, the skills of integration are. It would be trivial to solve if it did not have the one at the end. We will derive the solutions.
Separable Differential Equations
It would be trivial to solve if it did not have the one at the end. Dy dx = y x + 1 d y d x = y x + 1. To solve des, i.e., equations that involve derivatives, the skills of integration are. The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and the differential equation $y =. In summary, the conversation.
In Summary, The Conversation Discusses The Topic Of Differential Equations,.
The integral $\int \frac{1}{\sqrt[y]{y}} dy$ and the differential equation $y =. It would be trivial to solve if it did not have the one at the end. We will derive the solutions for homogeneous differential equations and we will. To solve des, i.e., equations that involve derivatives, the skills of integration are.