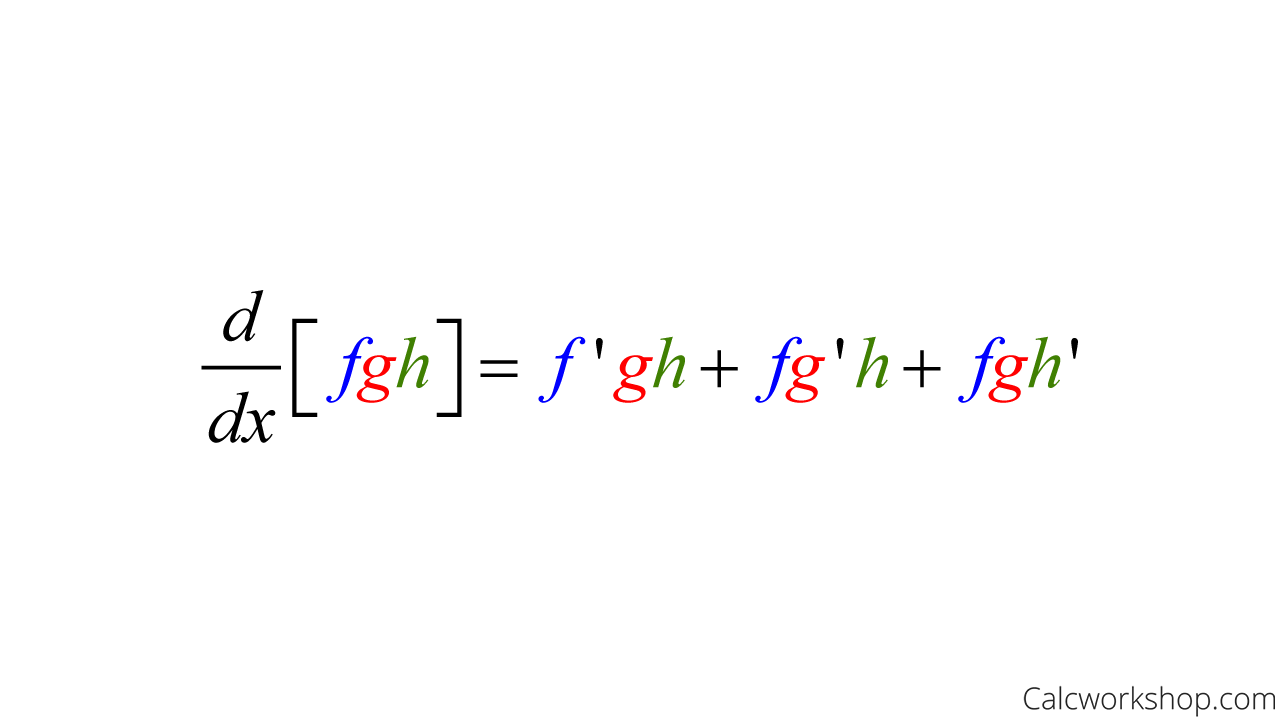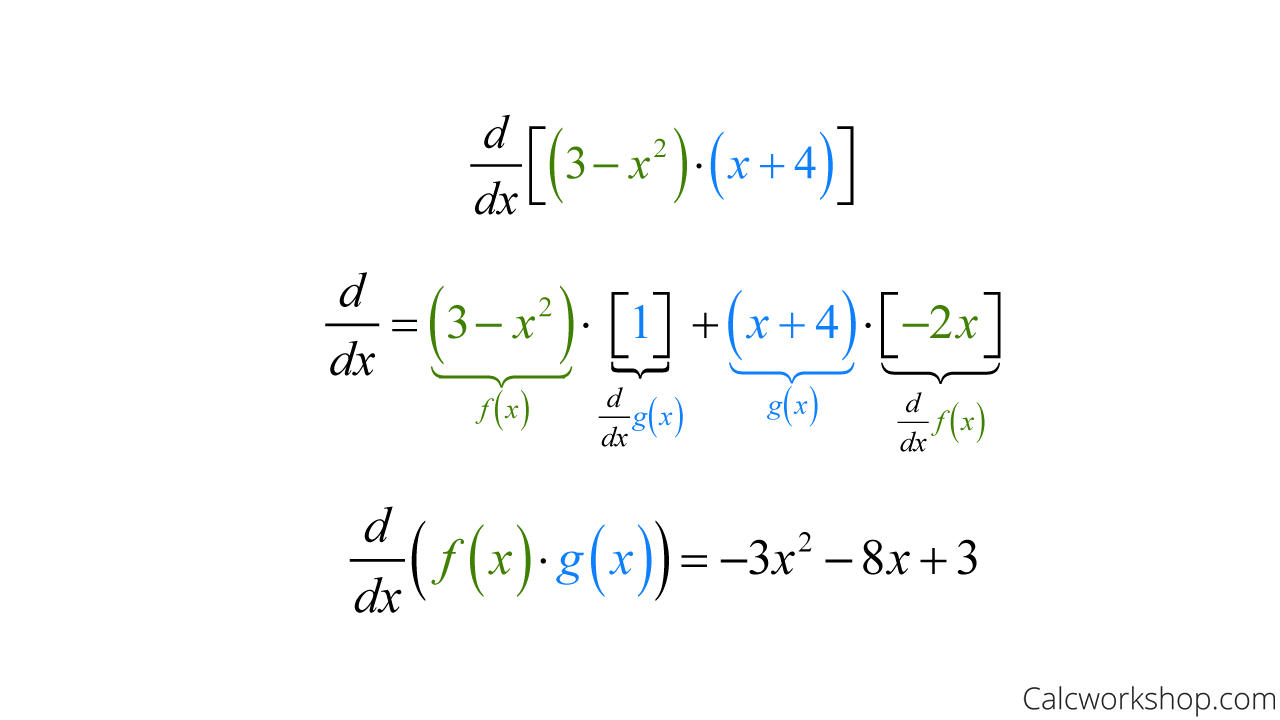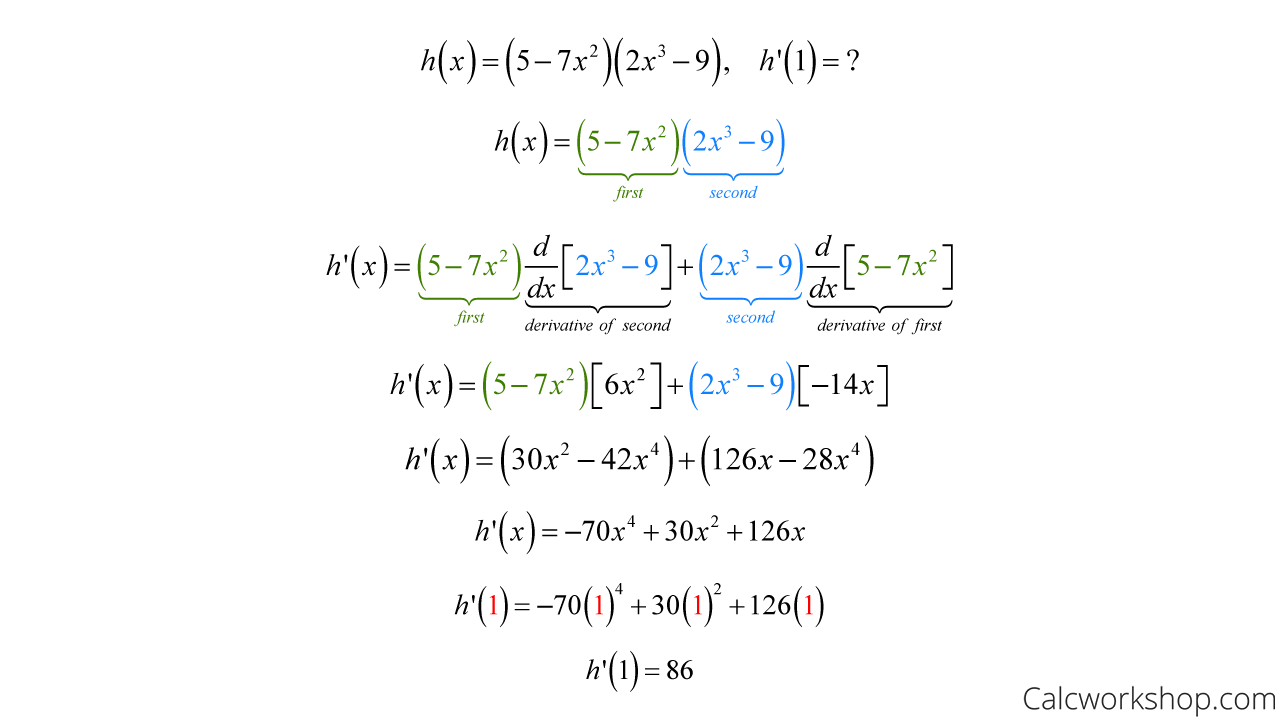Proof Of Product Rule Of Differentiation - Product rule in calculus is a method to find the derivative or differentiation of a function given in the form of a ratio or division of two. Consider the “difference quotient” i.e. Rewrite it in the form (because we only know. The product rule is a common rule for the differentiating problems where one function is multiplied by another function. Steps of the proof of product rule 1. ( +ℎ) ( +ℎ)− ( ) ( ) ℎ 2. It follows from the definition of derivative that if j j and k k are both differentiable on the interval i i, then: All we need to do is use the definition of the derivative alongside a simple algebraic trick. How i do i prove the product rule for derivatives? In calculus, the product rule (or leibniz rule[1] or leibniz product rule) is a formula used to find the derivatives of products of two or more.
The product rule is a common rule for the differentiating problems where one function is multiplied by another function. Steps of the proof of product rule 1. \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. It follows from the definition of derivative that if j j and k k are both differentiable on the interval i i, then: In calculus, the product rule (or leibniz rule[1] or leibniz product rule) is a formula used to find the derivatives of products of two or more. Consider the “difference quotient” i.e. ( +ℎ) ( +ℎ)− ( ) ( ) ℎ 2. All we need to do is use the definition of the derivative alongside a simple algebraic trick. Product rule in calculus is a method to find the derivative or differentiation of a function given in the form of a ratio or division of two. How i do i prove the product rule for derivatives?
\({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. The product rule is a common rule for the differentiating problems where one function is multiplied by another function. Product rule in calculus is a method to find the derivative or differentiation of a function given in the form of a ratio or division of two. It follows from the definition of derivative that if j j and k k are both differentiable on the interval i i, then: All we need to do is use the definition of the derivative alongside a simple algebraic trick. Rewrite it in the form (because we only know. Consider the “difference quotient” i.e. Steps of the proof of product rule 1. How i do i prove the product rule for derivatives? In calculus, the product rule (or leibniz rule[1] or leibniz product rule) is a formula used to find the derivatives of products of two or more.
Proof of Product Rule of Differentiation
\({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. Rewrite it in the form (because we only know. Steps of the proof of product rule 1. Product rule in calculus is a method to find the derivative or differentiation of a function given in the form of a ratio.
Differentiation, Product rule Teaching Resources
Product rule in calculus is a method to find the derivative or differentiation of a function given in the form of a ratio or division of two. ( +ℎ) ( +ℎ)− ( ) ( ) ℎ 2. How i do i prove the product rule for derivatives? Rewrite it in the form (because we only know. Consider the “difference quotient”.
Animated proof of the product rule Download Scientific Diagram
How i do i prove the product rule for derivatives? Rewrite it in the form (because we only know. ( +ℎ) ( +ℎ)− ( ) ( ) ℎ 2. \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. Consider the “difference quotient” i.e.
How to differentiate a product of two functions Basic Algebra
Rewrite it in the form (because we only know. Consider the “difference quotient” i.e. Product rule in calculus is a method to find the derivative or differentiation of a function given in the form of a ratio or division of two. The product rule is a common rule for the differentiating problems where one function is multiplied by another function..
Product Rule For Calculus (w/ StepbyStep Examples!)
How i do i prove the product rule for derivatives? Rewrite it in the form (because we only know. It follows from the definition of derivative that if j j and k k are both differentiable on the interval i i, then: Product rule in calculus is a method to find the derivative or differentiation of a function given in.
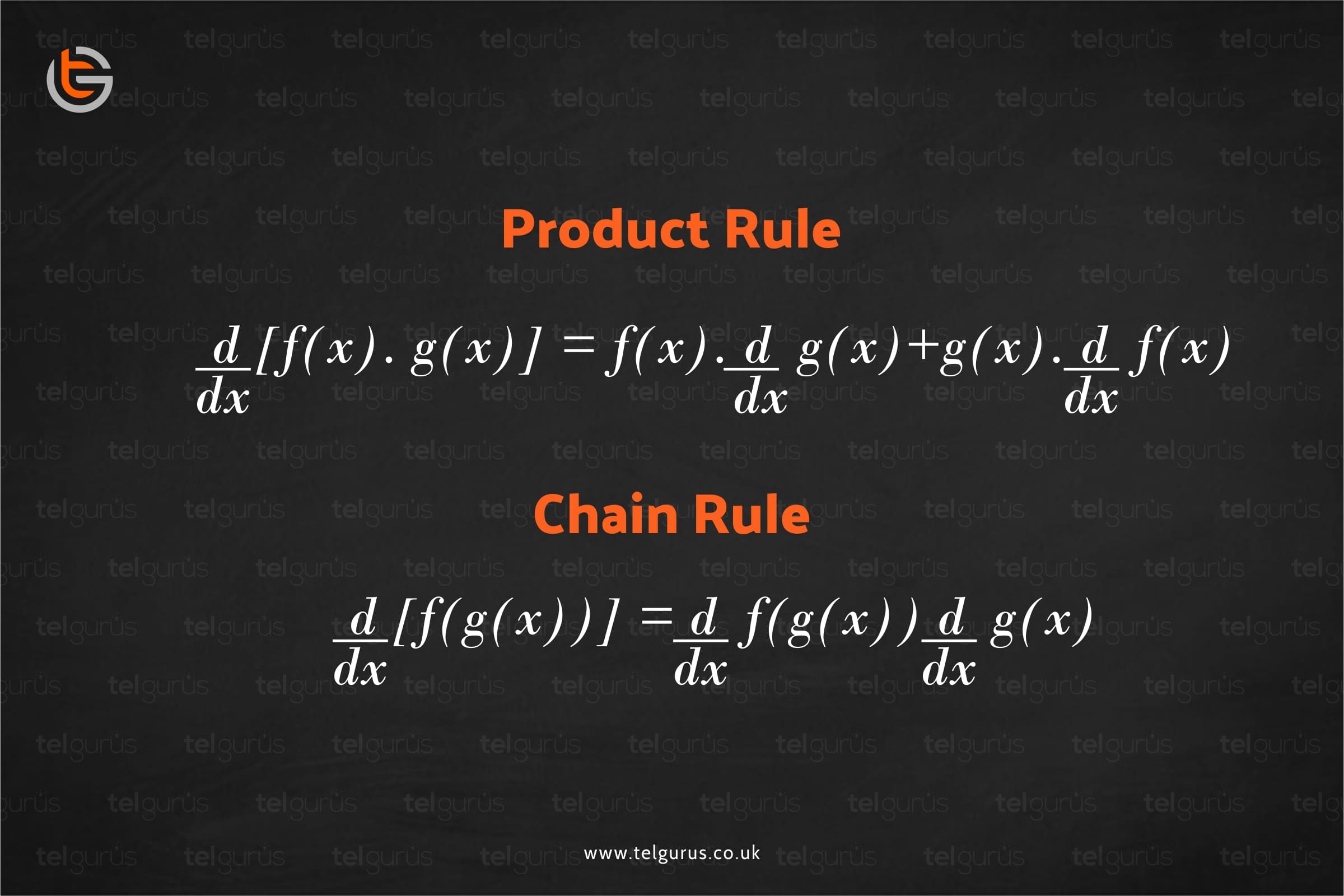
Chain Rule Vs Product Rule
Steps of the proof of product rule 1. It follows from the definition of derivative that if j j and k k are both differentiable on the interval i i, then: How i do i prove the product rule for derivatives? Consider the “difference quotient” i.e. \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above,.
Product Rule For Calculus (w/ StepbyStep Examples!)
Steps of the proof of product rule 1. Product rule in calculus is a method to find the derivative or differentiation of a function given in the form of a ratio or division of two. All we need to do is use the definition of the derivative alongside a simple algebraic trick. Consider the “difference quotient” i.e. \({\left( {f\,g} \right)^\prime.
When do I use the chain rule and when do I use the product rule in
\({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. It follows from the definition of derivative that if j j and k k are both differentiable on the interval i i, then: How i do i prove the product rule for derivatives? Rewrite it in the form (because we.
Proof Differentiation PDF
It follows from the definition of derivative that if j j and k k are both differentiable on the interval i i, then: Rewrite it in the form (because we only know. All we need to do is use the definition of the derivative alongside a simple algebraic trick. \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the.
Product Rule For Calculus (w/ StepbyStep Examples!)
It follows from the definition of derivative that if j j and k k are both differentiable on the interval i i, then: The product rule is a common rule for the differentiating problems where one function is multiplied by another function. \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can.
In Calculus, The Product Rule (Or Leibniz Rule[1] Or Leibniz Product Rule) Is A Formula Used To Find The Derivatives Of Products Of Two Or More.
Steps of the proof of product rule 1. Consider the “difference quotient” i.e. It follows from the definition of derivative that if j j and k k are both differentiable on the interval i i, then: \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved.
( +ℎ) ( +ℎ)− ( ) ( ) ℎ 2.
All we need to do is use the definition of the derivative alongside a simple algebraic trick. The product rule is a common rule for the differentiating problems where one function is multiplied by another function. How i do i prove the product rule for derivatives? Product rule in calculus is a method to find the derivative or differentiation of a function given in the form of a ratio or division of two.