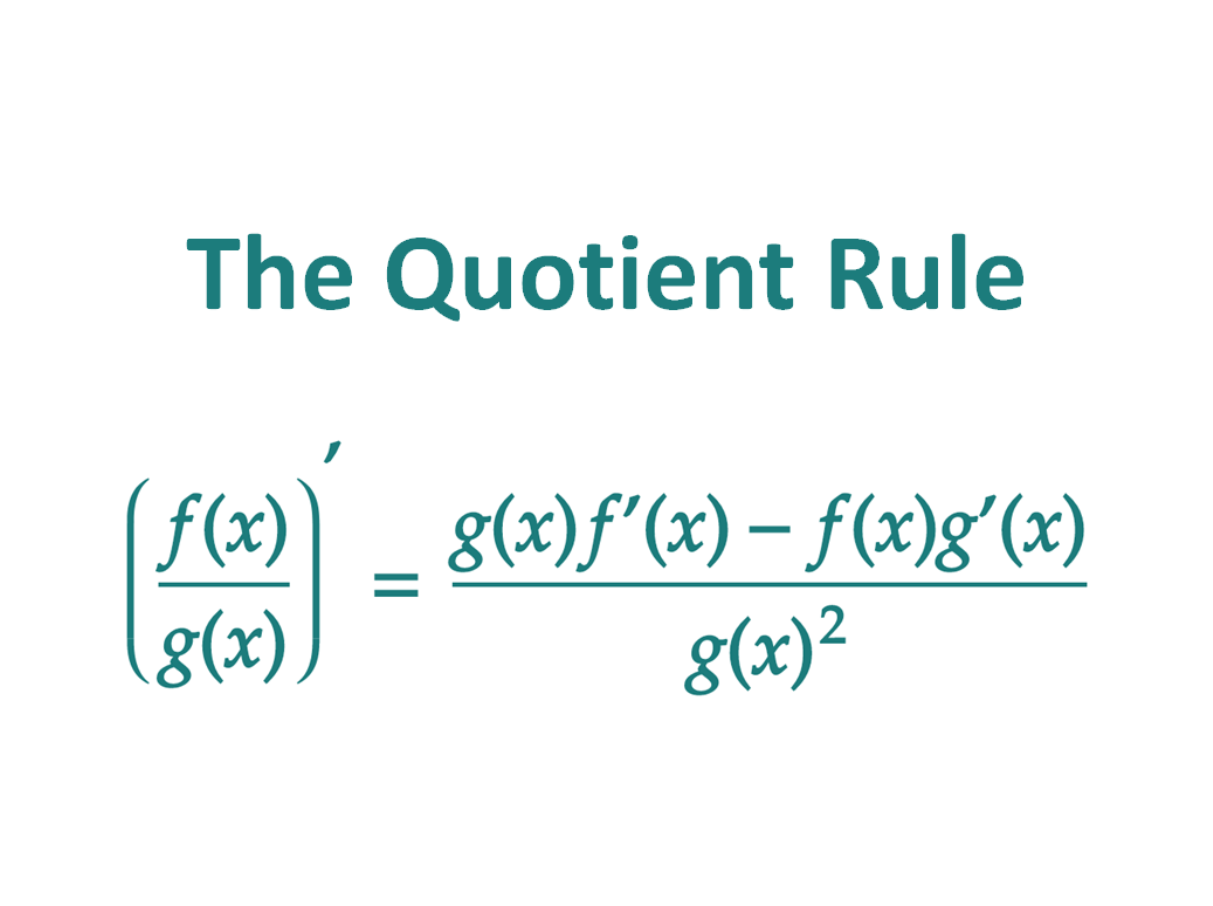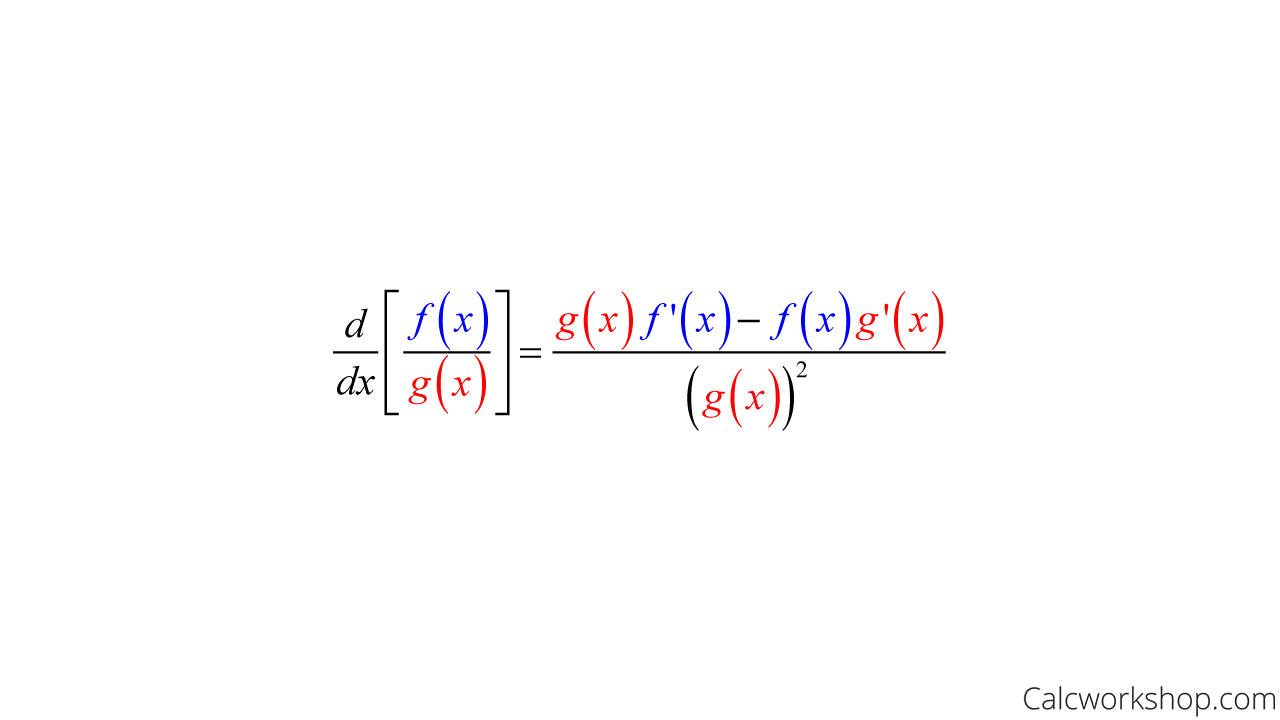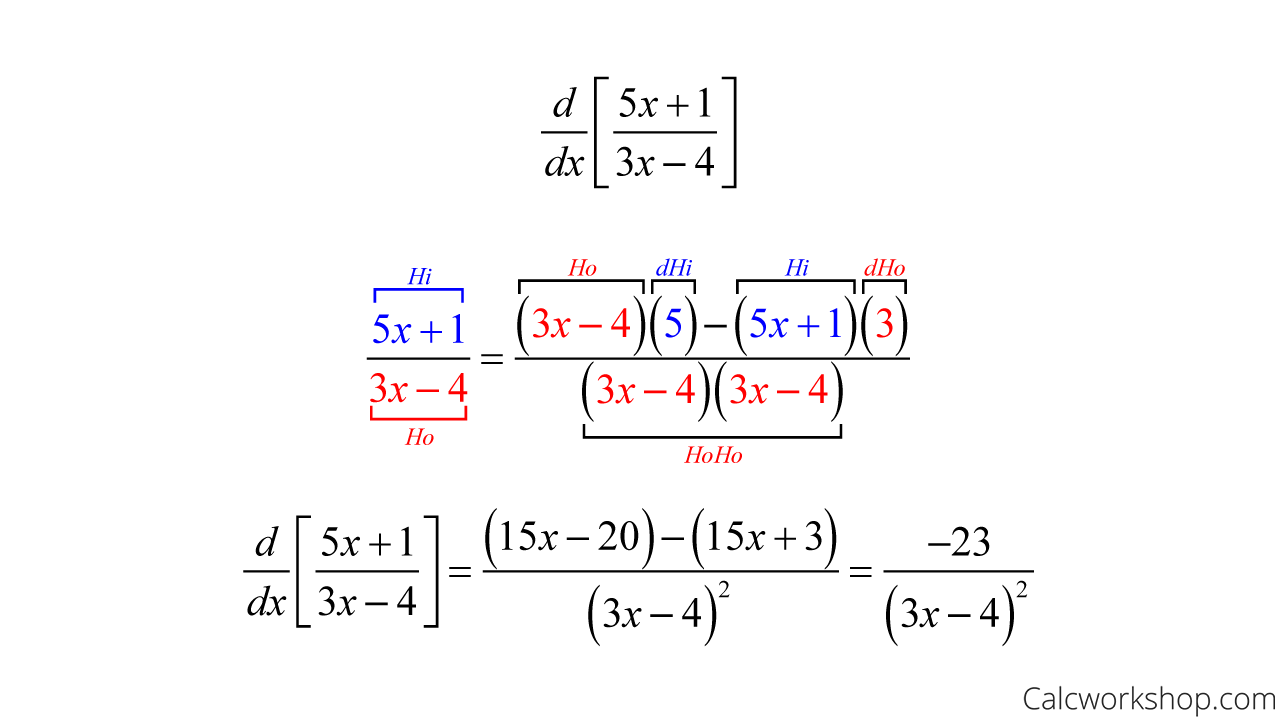Prove The Quotient Rule Of Differentiation - Let h ( x ) = f ( x ) g ( x ). Let ξ ∈ i ξ ∈ i be a point in i i at which both j j and k k. The proof of the quotient rule is shown in the proof of various derivative formulas section of the extras chapter. The quotient rule can be proved either by using the definition of the derivative, or thinking of the quotient \frac {f (x)} {g (x)} as the product f (x). Let j(x), k(x) j (x), k (x) be real functions defined on the open interval i i. In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions.
The quotient rule can be proved either by using the definition of the derivative, or thinking of the quotient \frac {f (x)} {g (x)} as the product f (x). Let j(x), k(x) j (x), k (x) be real functions defined on the open interval i i. Let h ( x ) = f ( x ) g ( x ). In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions. Let ξ ∈ i ξ ∈ i be a point in i i at which both j j and k k. The proof of the quotient rule is shown in the proof of various derivative formulas section of the extras chapter.
The quotient rule can be proved either by using the definition of the derivative, or thinking of the quotient \frac {f (x)} {g (x)} as the product f (x). The proof of the quotient rule is shown in the proof of various derivative formulas section of the extras chapter. Let j(x), k(x) j (x), k (x) be real functions defined on the open interval i i. In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions. Let ξ ∈ i ξ ∈ i be a point in i i at which both j j and k k. Let h ( x ) = f ( x ) g ( x ).
Quotient Rule Definition
In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions. The proof of the quotient rule is shown in the proof of various derivative formulas section of the extras chapter. Let ξ ∈ i ξ ∈ i be a point in i i at which both j.
Differentiation, Quotient rule Teaching Resources
Let j(x), k(x) j (x), k (x) be real functions defined on the open interval i i. The proof of the quotient rule is shown in the proof of various derivative formulas section of the extras chapter. Let h ( x ) = f ( x ) g ( x ). In calculus, the quotient rule is a method of.
Quotient Rule Differentiation Worksheet
Let ξ ∈ i ξ ∈ i be a point in i i at which both j j and k k. In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions. Let h ( x ) = f ( x ) g ( x ). Let j(x),.
Using the rule differentiation quotient of two functions, prove that d
The quotient rule can be proved either by using the definition of the derivative, or thinking of the quotient \frac {f (x)} {g (x)} as the product f (x). Let h ( x ) = f ( x ) g ( x ). Let ξ ∈ i ξ ∈ i be a point in i i at which both j.
The Quotient Rule DerivativeIt
In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions. The quotient rule can be proved either by using the definition of the derivative, or thinking of the quotient \frac {f (x)} {g (x)} as the product f (x). Let ξ ∈ i ξ ∈ i be.
Quotient Rule For Calculus (w/ StepbyStep Examples!)
Let h ( x ) = f ( x ) g ( x ). The quotient rule can be proved either by using the definition of the derivative, or thinking of the quotient \frac {f (x)} {g (x)} as the product f (x). In calculus, the quotient rule is a method of finding the derivative of a function that is.
Differentiation Product & Quotient Rule Kappa Maths Resources for A
Let j(x), k(x) j (x), k (x) be real functions defined on the open interval i i. In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions. Let ξ ∈ i ξ ∈ i be a point in i i at which both j j and k.
Quotient Rule Derivative
Let j(x), k(x) j (x), k (x) be real functions defined on the open interval i i. In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions. The quotient rule can be proved either by using the definition of the derivative, or thinking of the quotient \frac.
vi) Using the rule differentiation quotient of two functions, prove
Let h ( x ) = f ( x ) g ( x ). The proof of the quotient rule is shown in the proof of various derivative formulas section of the extras chapter. Let j(x), k(x) j (x), k (x) be real functions defined on the open interval i i. In calculus, the quotient rule is a method of.
Quotient Rule Formula, Definition, Proof, And Examples, 55 OFF
Let ξ ∈ i ξ ∈ i be a point in i i at which both j j and k k. In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions. The quotient rule can be proved either by using the definition of the derivative, or thinking.
In Calculus, The Quotient Rule Is A Method Of Finding The Derivative Of A Function That Is The Ratio Of Two Differentiable Functions.
Let ξ ∈ i ξ ∈ i be a point in i i at which both j j and k k. The quotient rule can be proved either by using the definition of the derivative, or thinking of the quotient \frac {f (x)} {g (x)} as the product f (x). The proof of the quotient rule is shown in the proof of various derivative formulas section of the extras chapter. Let j(x), k(x) j (x), k (x) be real functions defined on the open interval i i.