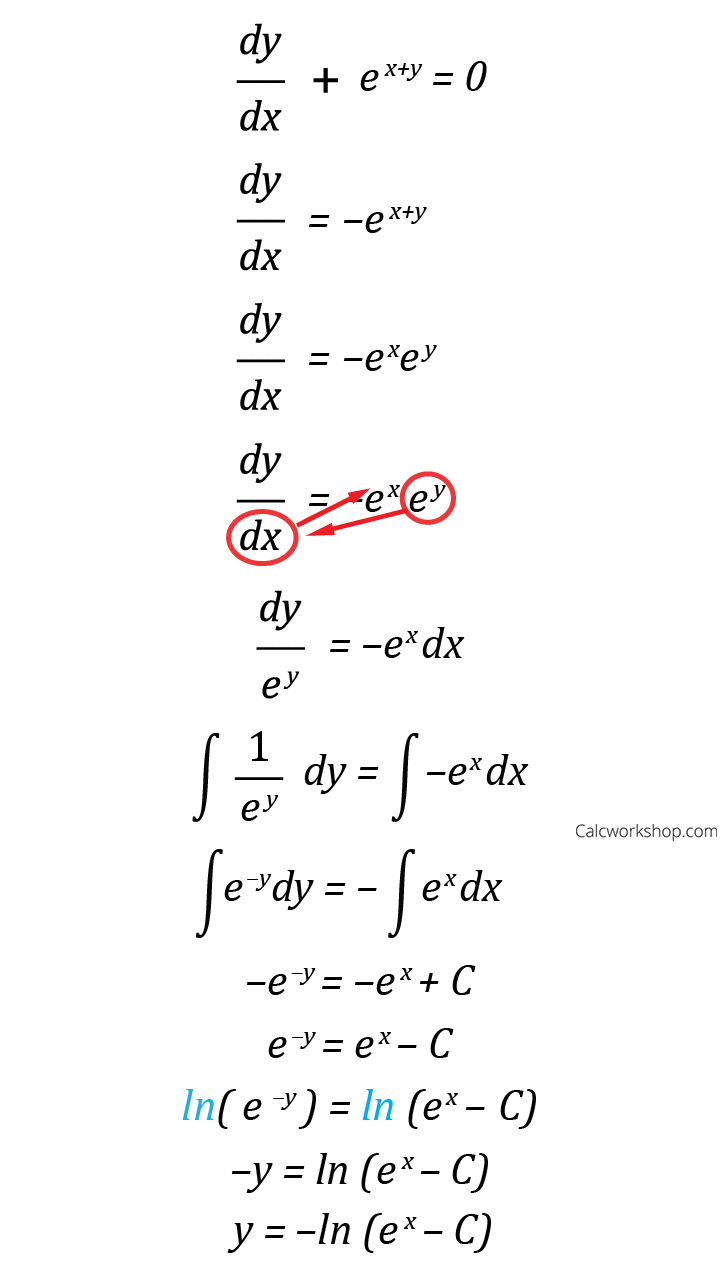
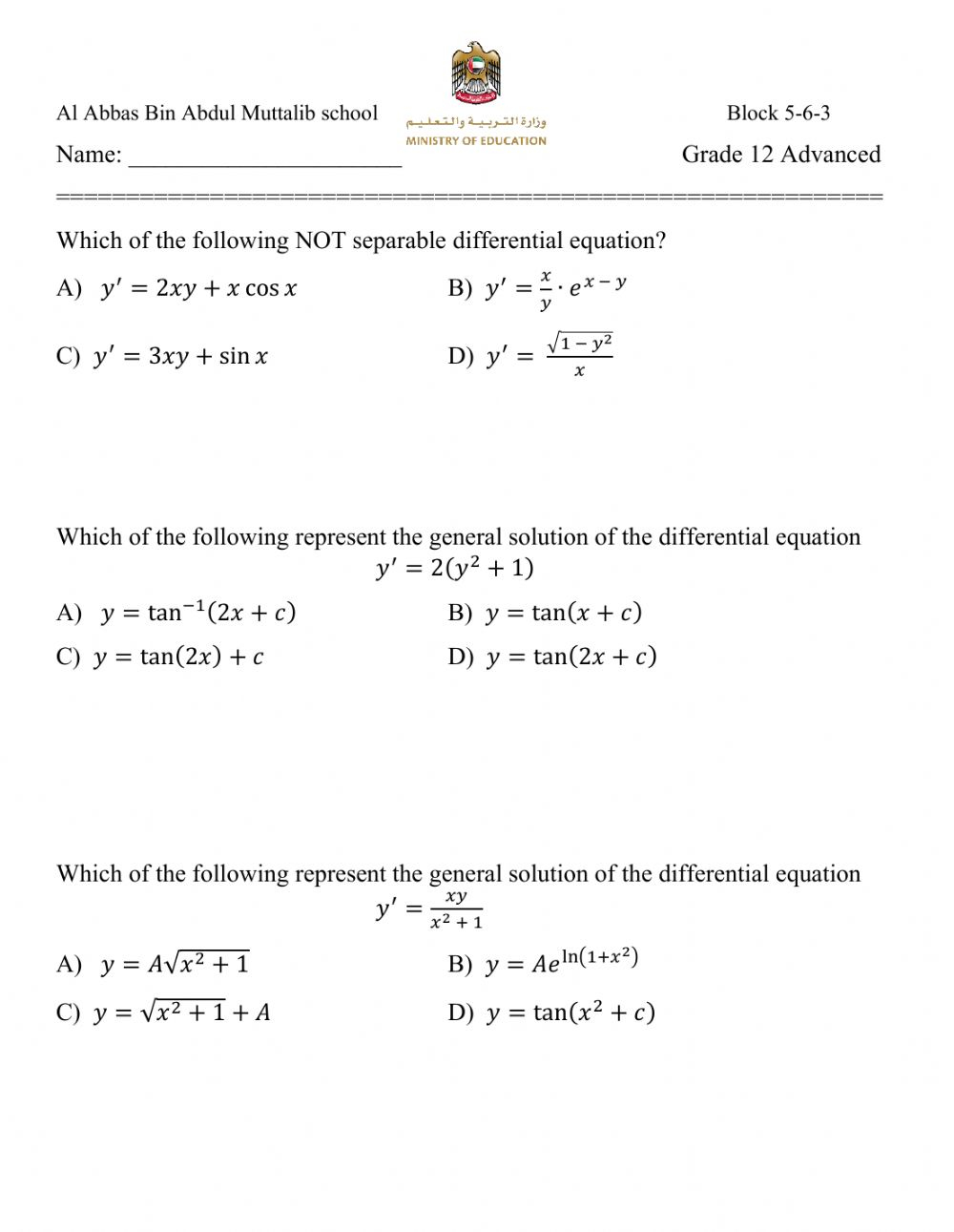
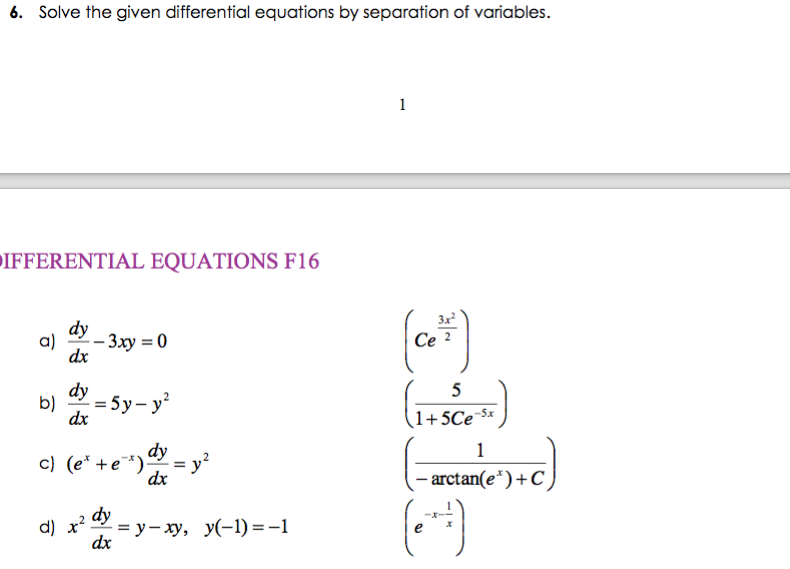
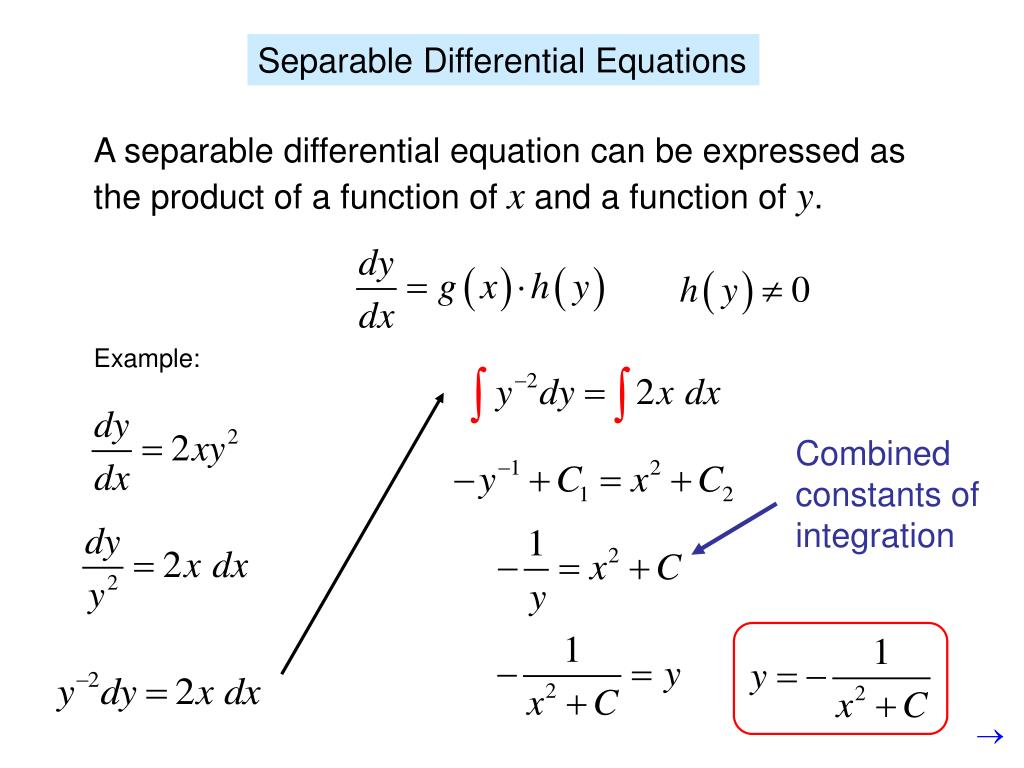
Separation Differential Equations - Z eydy = z 3x2dx i.e. We will now learn our first technique for solving differential equation. In this section we solve separable first order differential equations, i.e. Differential equations in the form n(y) y' = m(x). G(y) = e−y, so we can separate the variables and then integrate, i.e. In this section show how the method of separation of variables can be applied to a partial differential equation to reduce the. Ey = x3 +a (where a = arbitrary constant).
Ey = x3 +a (where a = arbitrary constant). In this section show how the method of separation of variables can be applied to a partial differential equation to reduce the. We will now learn our first technique for solving differential equation. G(y) = e−y, so we can separate the variables and then integrate, i.e. In this section we solve separable first order differential equations, i.e. Differential equations in the form n(y) y' = m(x). Z eydy = z 3x2dx i.e.
We will now learn our first technique for solving differential equation. In this section we solve separable first order differential equations, i.e. Ey = x3 +a (where a = arbitrary constant). G(y) = e−y, so we can separate the variables and then integrate, i.e. In this section show how the method of separation of variables can be applied to a partial differential equation to reduce the. Differential equations in the form n(y) y' = m(x). Z eydy = z 3x2dx i.e.
[Solved] Use separation of variables to solve the differential
In this section show how the method of separation of variables can be applied to a partial differential equation to reduce the. Ey = x3 +a (where a = arbitrary constant). We will now learn our first technique for solving differential equation. Differential equations in the form n(y) y' = m(x). In this section we solve separable first order differential.
Separable Equations
Z eydy = z 3x2dx i.e. Ey = x3 +a (where a = arbitrary constant). Differential equations in the form n(y) y' = m(x). We will now learn our first technique for solving differential equation. G(y) = e−y, so we can separate the variables and then integrate, i.e.
Separable Differential Equations Worksheet Equations Worksheets
G(y) = e−y, so we can separate the variables and then integrate, i.e. Z eydy = z 3x2dx i.e. Ey = x3 +a (where a = arbitrary constant). Differential equations in the form n(y) y' = m(x). We will now learn our first technique for solving differential equation.
SOLUTION Differential equations separation of variables Studypool
In this section show how the method of separation of variables can be applied to a partial differential equation to reduce the. Z eydy = z 3x2dx i.e. Differential equations in the form n(y) y' = m(x). In this section we solve separable first order differential equations, i.e. We will now learn our first technique for solving differential equation.
[Solved] Solve the given differential equation by separation of
Ey = x3 +a (where a = arbitrary constant). In this section we solve separable first order differential equations, i.e. We will now learn our first technique for solving differential equation. Z eydy = z 3x2dx i.e. Differential equations in the form n(y) y' = m(x).
[Solved] Solve the given differential equation by separation of
In this section we solve separable first order differential equations, i.e. Z eydy = z 3x2dx i.e. In this section show how the method of separation of variables can be applied to a partial differential equation to reduce the. G(y) = e−y, so we can separate the variables and then integrate, i.e. We will now learn our first technique for.
[Solved] Solve the given differential equation by separation of
In this section we solve separable first order differential equations, i.e. Differential equations in the form n(y) y' = m(x). Z eydy = z 3x2dx i.e. G(y) = e−y, so we can separate the variables and then integrate, i.e. Ey = x3 +a (where a = arbitrary constant).
Solved Solve the given differential equations by separation
G(y) = e−y, so we can separate the variables and then integrate, i.e. In this section we solve separable first order differential equations, i.e. Ey = x3 +a (where a = arbitrary constant). We will now learn our first technique for solving differential equation. In this section show how the method of separation of variables can be applied to a.
Using separation of variables in solving partial differential equations
Ey = x3 +a (where a = arbitrary constant). We will now learn our first technique for solving differential equation. Z eydy = z 3x2dx i.e. In this section show how the method of separation of variables can be applied to a partial differential equation to reduce the. G(y) = e−y, so we can separate the variables and then integrate,.
Separable Differential Equations Worksheet With Answers Equations
In this section we solve separable first order differential equations, i.e. In this section show how the method of separation of variables can be applied to a partial differential equation to reduce the. G(y) = e−y, so we can separate the variables and then integrate, i.e. We will now learn our first technique for solving differential equation. Z eydy =.
Ey = X3 +A (Where A = Arbitrary Constant).
Differential equations in the form n(y) y' = m(x). G(y) = e−y, so we can separate the variables and then integrate, i.e. We will now learn our first technique for solving differential equation. Z eydy = z 3x2dx i.e.
In This Section We Solve Separable First Order Differential Equations, I.e.
In this section show how the method of separation of variables can be applied to a partial differential equation to reduce the.