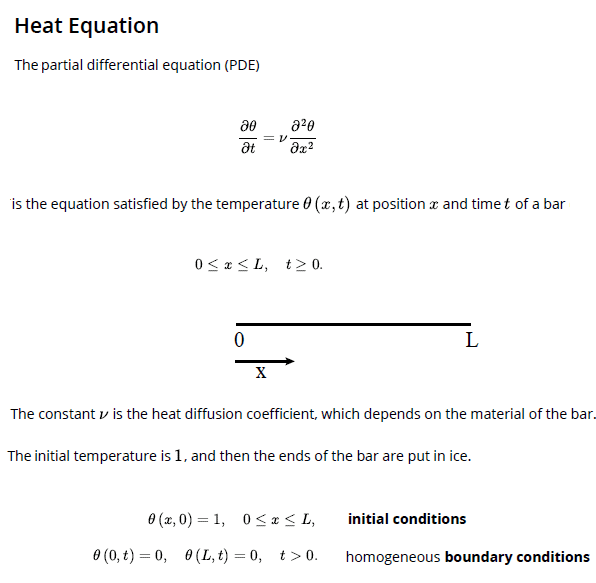
Series And Differential Equations - In this section we define ordinary and singular points for a differential equation. Example 1 use power series to solve the equation y y 0. By writing out the first few terms of (4), you can see that it is the same as (3). Determine the differential equation and choose the point [asciimath]x_0[/asciimath]. We now reach a significant step in solving differential equations via series solutions. We also show who to construct a series solution for. In order for the expression in (8) to hold for all values of. How to find a series solution to a differential equation 1.
By writing out the first few terms of (4), you can see that it is the same as (3). We also show who to construct a series solution for. Example 1 use power series to solve the equation y y 0. In this section we define ordinary and singular points for a differential equation. We now reach a significant step in solving differential equations via series solutions. Determine the differential equation and choose the point [asciimath]x_0[/asciimath]. How to find a series solution to a differential equation 1. In order for the expression in (8) to hold for all values of.
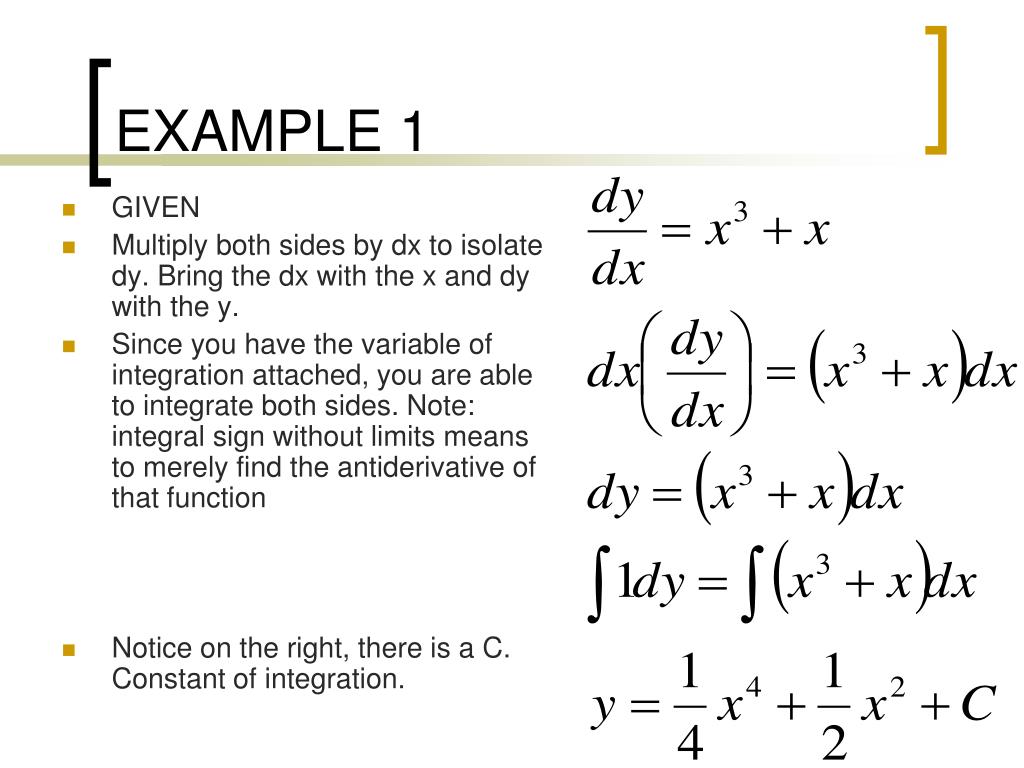
Example 1 use power series to solve the equation y y 0. By writing out the first few terms of (4), you can see that it is the same as (3). Determine the differential equation and choose the point [asciimath]x_0[/asciimath]. We now reach a significant step in solving differential equations via series solutions. We also show who to construct a series solution for. How to find a series solution to a differential equation 1. In this section we define ordinary and singular points for a differential equation. In order for the expression in (8) to hold for all values of.
Show Answer
How to find a series solution to a differential equation 1. In this section we define ordinary and singular points for a differential equation. Example 1 use power series to solve the equation y y 0. We now reach a significant step in solving differential equations via series solutions. We also show who to construct a series solution for.
[Solved] Ordinary Differential equation. Use the power series method to
In this section we define ordinary and singular points for a differential equation. How to find a series solution to a differential equation 1. In order for the expression in (8) to hold for all values of. Determine the differential equation and choose the point [asciimath]x_0[/asciimath]. By writing out the first few terms of (4), you can see that it.
Fourier Series and Differential Equations with some applications in R
In order for the expression in (8) to hold for all values of. By writing out the first few terms of (4), you can see that it is the same as (3). We also show who to construct a series solution for. Example 1 use power series to solve the equation y y 0. Determine the differential equation and choose.
First order differential equations Teaching Resources
By writing out the first few terms of (4), you can see that it is the same as (3). Determine the differential equation and choose the point [asciimath]x_0[/asciimath]. Example 1 use power series to solve the equation y y 0. We also show who to construct a series solution for. In order for the expression in (8) to hold for.
What are the differential equations? Types of Differential Equations
In order for the expression in (8) to hold for all values of. In this section we define ordinary and singular points for a differential equation. Determine the differential equation and choose the point [asciimath]x_0[/asciimath]. How to find a series solution to a differential equation 1. We now reach a significant step in solving differential equations via series solutions.
Power Series Solution Of Differential Equations
Example 1 use power series to solve the equation y y 0. In this section we define ordinary and singular points for a differential equation. In order for the expression in (8) to hold for all values of. Determine the differential equation and choose the point [asciimath]x_0[/asciimath]. How to find a series solution to a differential equation 1.
2nd order differential equations Teaching Resources
In order for the expression in (8) to hold for all values of. By writing out the first few terms of (4), you can see that it is the same as (3). In this section we define ordinary and singular points for a differential equation. Example 1 use power series to solve the equation y y 0. We also show.
What Are Differential Equations Images and Photos finder
Determine the differential equation and choose the point [asciimath]x_0[/asciimath]. By writing out the first few terms of (4), you can see that it is the same as (3). Example 1 use power series to solve the equation y y 0. How to find a series solution to a differential equation 1. In order for the expression in (8) to hold.
Application of fourier series to differential equations
We also show who to construct a series solution for. In order for the expression in (8) to hold for all values of. Determine the differential equation and choose the point [asciimath]x_0[/asciimath]. We now reach a significant step in solving differential equations via series solutions. In this section we define ordinary and singular points for a differential equation.
SOLUTION Differential equations fourier series Studypool
In this section we define ordinary and singular points for a differential equation. In order for the expression in (8) to hold for all values of. Determine the differential equation and choose the point [asciimath]x_0[/asciimath]. How to find a series solution to a differential equation 1. We now reach a significant step in solving differential equations via series solutions.
How To Find A Series Solution To A Differential Equation 1.
Example 1 use power series to solve the equation y y 0. In this section we define ordinary and singular points for a differential equation. We also show who to construct a series solution for. Determine the differential equation and choose the point [asciimath]x_0[/asciimath].
By Writing Out The First Few Terms Of (4), You Can See That It Is The Same As (3).
In order for the expression in (8) to hold for all values of. We now reach a significant step in solving differential equations via series solutions.