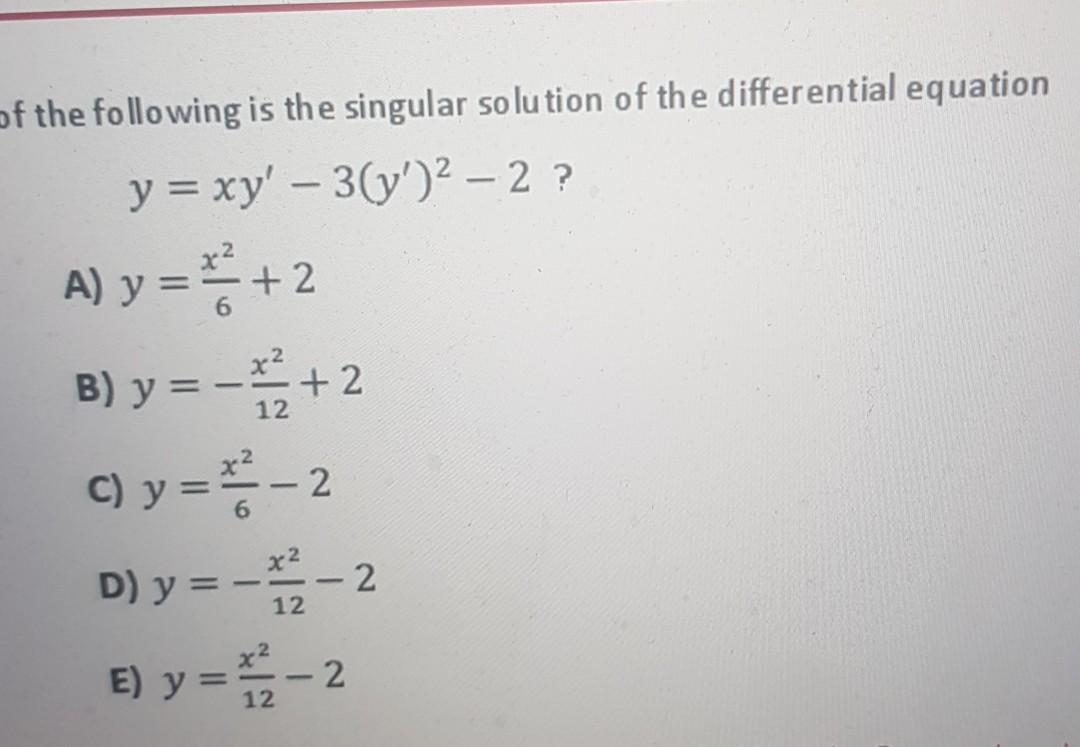Singular Solution Of Differential Equation - See examples with critical points, equilibrium solutions and. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where. Learn how to identify and solve singular solutions of separable differential equations.
Learn how to identify and solve singular solutions of separable differential equations. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where. See examples with critical points, equilibrium solutions and.
See examples with critical points, equilibrium solutions and. Learn how to identify and solve singular solutions of separable differential equations. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where.
[Solved] Determine singular points, of each differential equation and
Learn how to identify and solve singular solutions of separable differential equations. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where. See examples with critical points, equilibrium solutions and.
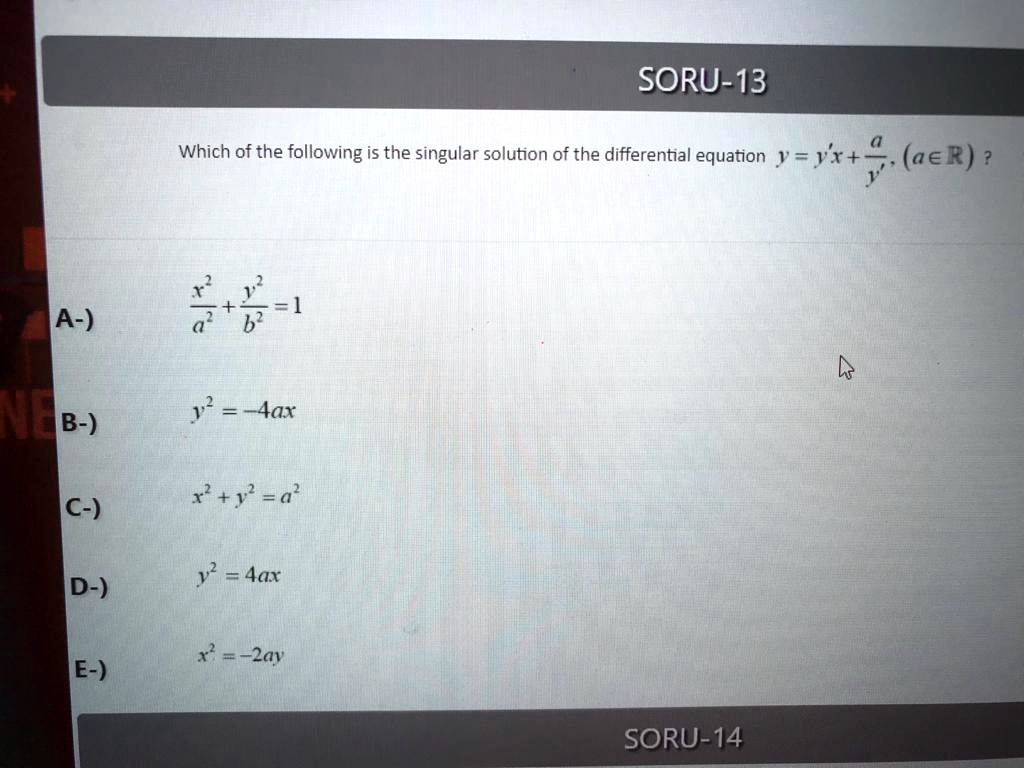
Solved which of the following is the singular solution of
See examples with critical points, equilibrium solutions and. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. Learn how to identify and solve singular solutions of separable differential equations. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where.
[Solved] Determine singular points, of each differential equation and
See examples with critical points, equilibrium solutions and. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. Learn how to identify and solve singular solutions of separable differential equations.
SOLVED 'singular solution SORU13 Which of the following is the
See examples with critical points, equilibrium solutions and. Learn how to identify and solve singular solutions of separable differential equations. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of.
(PDF) Existence of positive solution for singular fractional
Learn how to identify and solve singular solutions of separable differential equations. See examples with critical points, equilibrium solutions and. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where.
(PDF) Singular solutions of a singular differential equation
Learn how to identify and solve singular solutions of separable differential equations. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. See examples with critical points, equilibrium solutions and. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where.
SOLVEDA singular solution of a differential equation is a solution
See examples with critical points, equilibrium solutions and. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where. Learn how to identify and solve singular solutions of separable differential equations. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of.
[Solved] . Find all Singular points of the given differential equation
See examples with critical points, equilibrium solutions and. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where. Learn how to identify and solve singular solutions of separable differential equations. A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of.
[Solved] Determine the singular points of the given differential
A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. Learn how to identify and solve singular solutions of separable differential equations. See examples with critical points, equilibrium solutions and. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where.
Solved of the following is the singular solution of the
A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. See examples with critical points, equilibrium solutions and. Learn how to identify and solve singular solutions of separable differential equations. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where.
Learn How To Identify And Solve Singular Solutions Of Separable Differential Equations.
A solution of the differential equation (1) which cannot be obtained from the general integral, is called a singular solution of. See examples with critical points, equilibrium solutions and. If your given differential equation is of clairaut's form, i.e., of the form $$y=px+f(p)\qquad \text{where.