Systems Of Linear Differential Equations - In this section we will look at some of the basics of systems of differential equations. , xn(t) vectors in vn(i). A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. Linear systems of differential equations “do not worry too much about your difficulties in mathematics, i can assure you that mine are. , xn](t0) 6= 0 for a given. Then vn(i) is a vector space. Section 10.3 deals with the basic theory of homogeneous. We show how to convert a system of. Section 10.2 discusses linear systems of differential equations.
Then vn(i) is a vector space. In this section we will look at some of the basics of systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. Section 10.2 discusses linear systems of differential equations. Linear systems of differential equations “do not worry too much about your difficulties in mathematics, i can assure you that mine are. , xn](t0) 6= 0 for a given. We show how to convert a system of. , xn(t) vectors in vn(i). Section 10.3 deals with the basic theory of homogeneous.
A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. Section 10.3 deals with the basic theory of homogeneous. Then vn(i) is a vector space. We show how to convert a system of. Linear systems of differential equations “do not worry too much about your difficulties in mathematics, i can assure you that mine are. In this section we will look at some of the basics of systems of differential equations. , xn(t) vectors in vn(i). , xn](t0) 6= 0 for a given. Section 10.2 discusses linear systems of differential equations.
Solving Systems of Linear Differential Equations by Elimination
Then vn(i) is a vector space. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. Section 10.3 deals with the basic theory of homogeneous. Section 10.2 discusses linear systems of differential equations. , xn](t0) 6= 0 for a given.
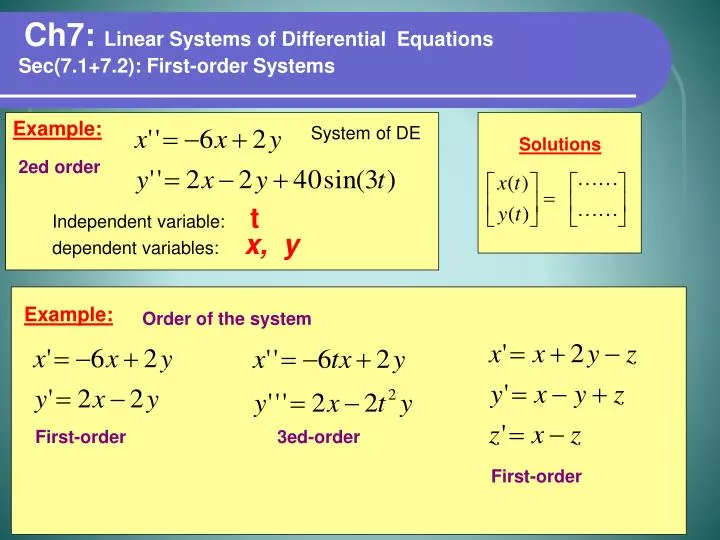
PPT Ch7 Linear Systems of Differential Equations PowerPoint
, xn(t) vectors in vn(i). In this section we will look at some of the basics of systems of differential equations. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. Then vn(i) is a vector space. , xn](t0) 6= 0 for a given.
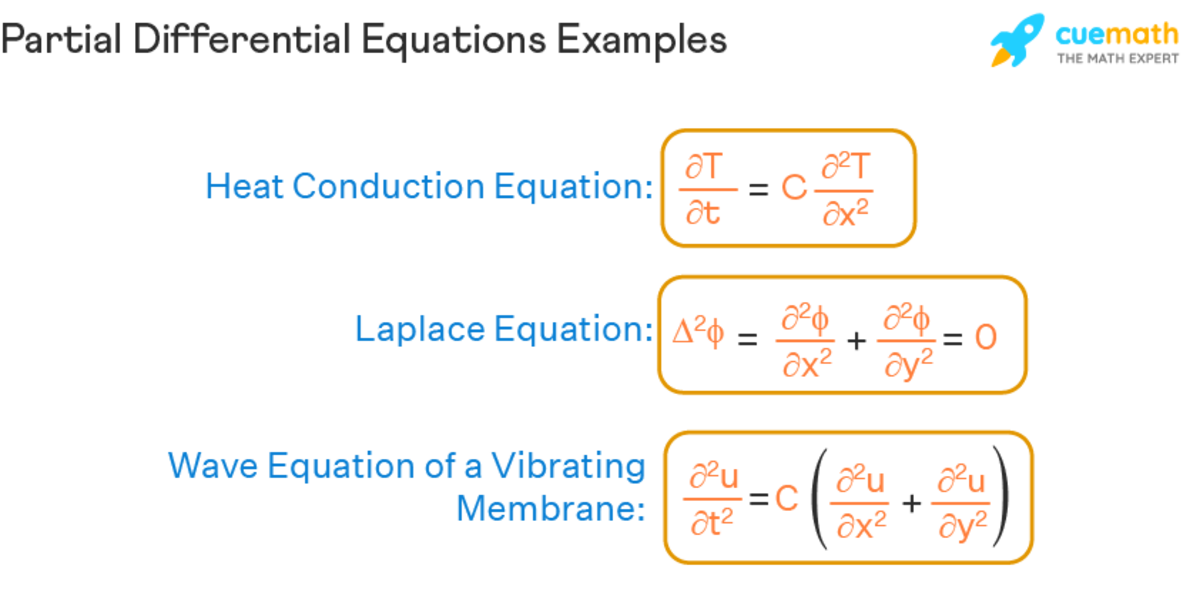
Differential Equations Owlcation
Section 10.3 deals with the basic theory of homogeneous. , xn](t0) 6= 0 for a given. We show how to convert a system of. Section 10.2 discusses linear systems of differential equations. In this section we will look at some of the basics of systems of differential equations.
(PDF) Systems of First Order Linear Differential Equations Utsav
, xn(t) vectors in vn(i). We show how to convert a system of. Then vn(i) is a vector space. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. Linear systems of differential equations “do not worry too much about your difficulties in mathematics, i can assure you that mine.
Systems of Differential Equations KZHU.ai 🚀
, xn(t) vectors in vn(i). , xn](t0) 6= 0 for a given. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. Then vn(i) is a vector space. In this section we will look at some of the basics of systems of differential equations.
PPT Ch7 Linear Systems of Differential Equations PowerPoint
Section 10.2 discusses linear systems of differential equations. Linear systems of differential equations “do not worry too much about your difficulties in mathematics, i can assure you that mine are. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. We show how to convert a system of. In this.
Solved (2) Systems of Linear Differential Equations and
A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. , xn(t) vectors in vn(i). Linear systems of differential equations “do not worry too much about your difficulties in mathematics, i can assure you that mine are. , xn](t0) 6= 0 for a given. In this section we will look.
Chapter 3 Linear Differential Equation PDF Equations Differential
Section 10.3 deals with the basic theory of homogeneous. Linear systems of differential equations “do not worry too much about your difficulties in mathematics, i can assure you that mine are. Section 10.2 discusses linear systems of differential equations. , xn(t) vectors in vn(i). Then vn(i) is a vector space.
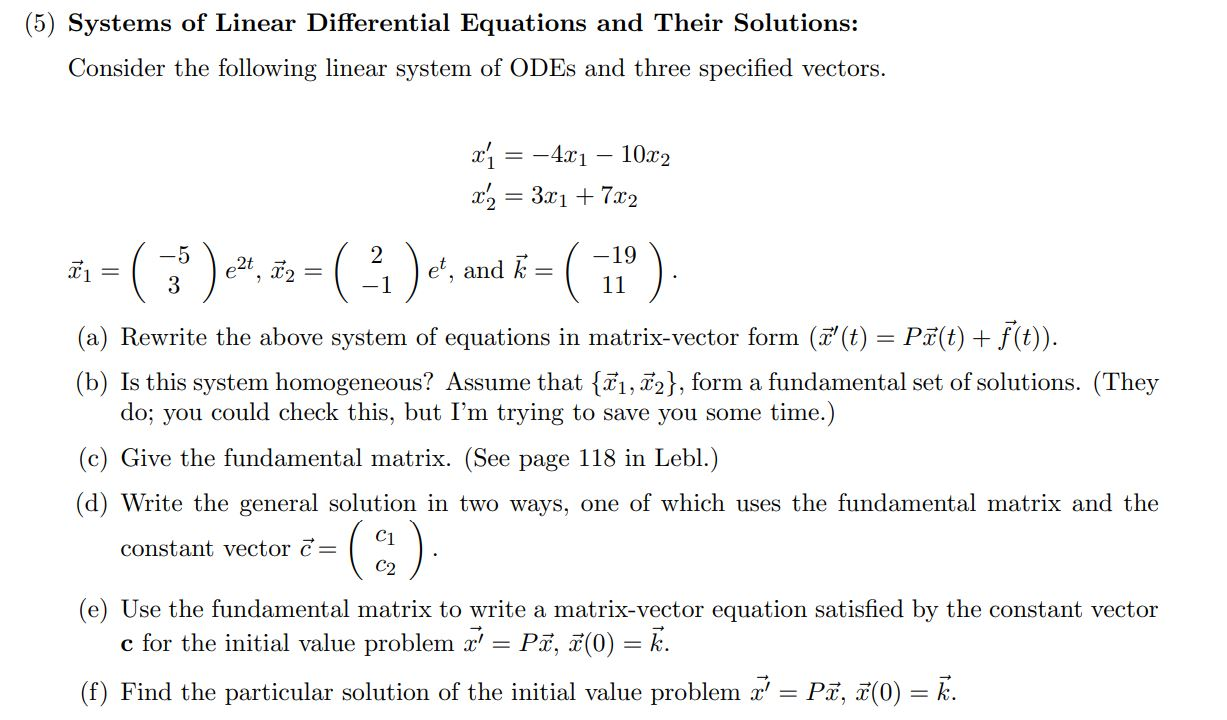
Solved (5) Systems of Linear Differential Equations and
, xn](t0) 6= 0 for a given. We show how to convert a system of. Section 10.2 discusses linear systems of differential equations. Linear systems of differential equations “do not worry too much about your difficulties in mathematics, i can assure you that mine are. Section 10.3 deals with the basic theory of homogeneous.
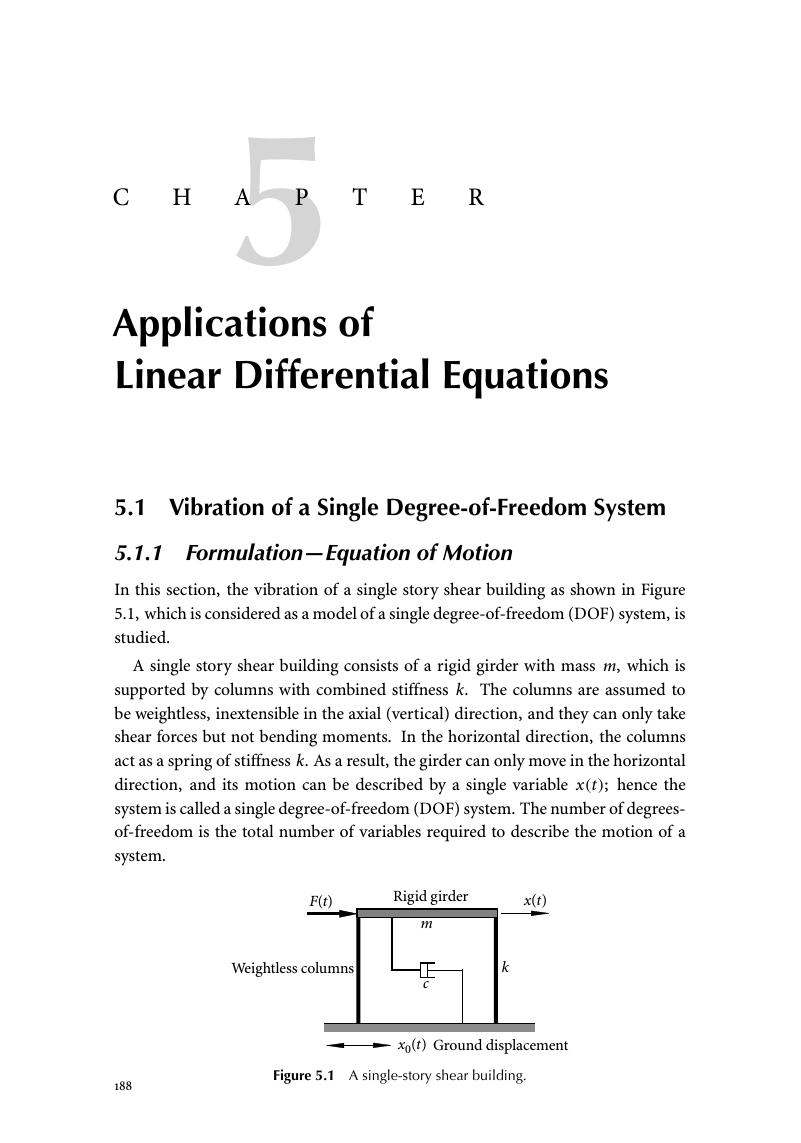
Applications of Linear Differential Equations (Chapter 5
In this section we will look at some of the basics of systems of differential equations. We show how to convert a system of. , xn](t0) 6= 0 for a given. Then vn(i) is a vector space. Linear systems of differential equations “do not worry too much about your difficulties in mathematics, i can assure you that mine are.
Section 10.2 Discusses Linear Systems Of Differential Equations.
Then vn(i) is a vector space. , xn(t) vectors in vn(i). , xn](t0) 6= 0 for a given. In this section we will look at some of the basics of systems of differential equations.
Section 10.3 Deals With The Basic Theory Of Homogeneous.
Linear systems of differential equations “do not worry too much about your difficulties in mathematics, i can assure you that mine are. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. We show how to convert a system of.