What Makes A Graph Not Differentiable - How to check for when a function is not differentiable step 1: 1) the tangent line is vertical (and that. We'll look at all 3 cases. At points on the graph where you can draw many tangents, the derivative is not defined, and you. Check to see if the. But the function f in figure 1.7.6 is not differentiable at a = 1 because f′(1) fails to. This can happen in essentially two ways:
How to check for when a function is not differentiable step 1: At points on the graph where you can draw many tangents, the derivative is not defined, and you. We'll look at all 3 cases. But the function f in figure 1.7.6 is not differentiable at a = 1 because f′(1) fails to. 1) the tangent line is vertical (and that. Check to see if the. This can happen in essentially two ways:
Check to see if the. But the function f in figure 1.7.6 is not differentiable at a = 1 because f′(1) fails to. How to check for when a function is not differentiable step 1: At points on the graph where you can draw many tangents, the derivative is not defined, and you. We'll look at all 3 cases. 1) the tangent line is vertical (and that. This can happen in essentially two ways:
calculus Continuous,Discontinuous ,Differential and non
This can happen in essentially two ways: 1) the tangent line is vertical (and that. Check to see if the. But the function f in figure 1.7.6 is not differentiable at a = 1 because f′(1) fails to. How to check for when a function is not differentiable step 1:
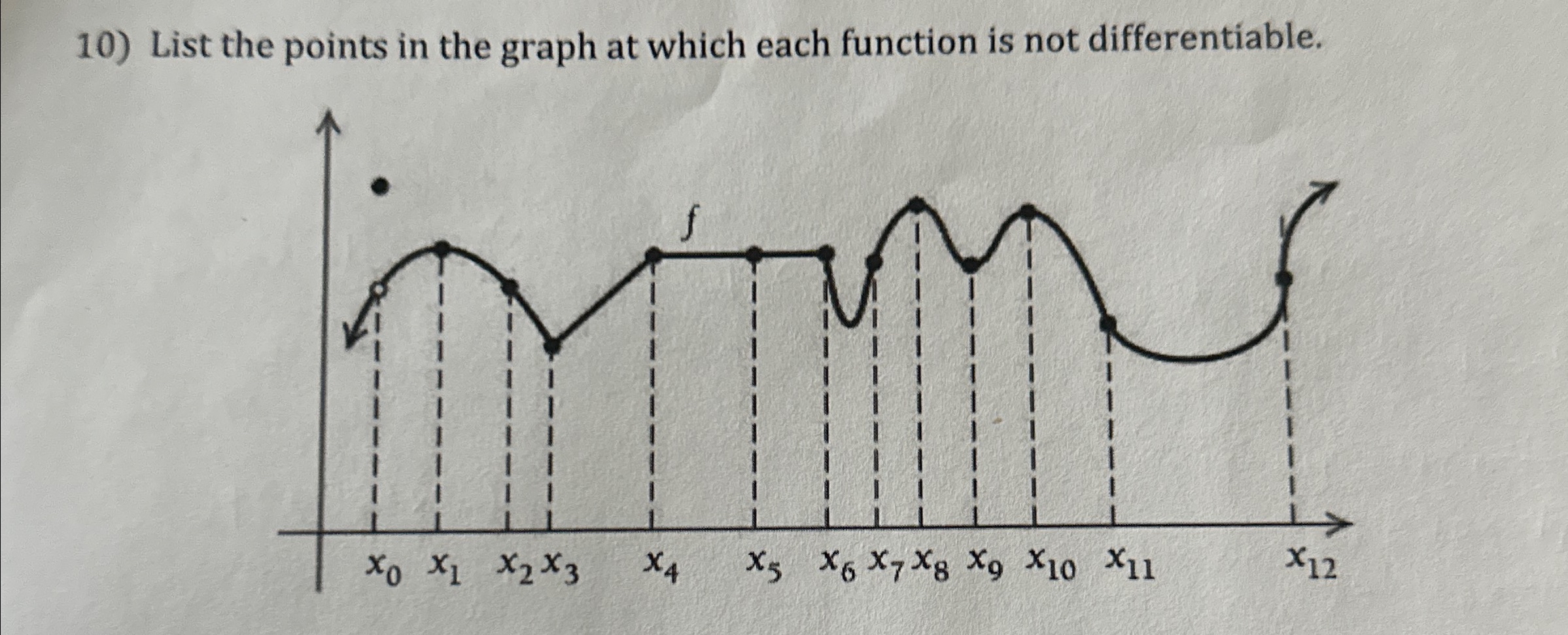
Solved List the points in the graph at which each function
How to check for when a function is not differentiable step 1: We'll look at all 3 cases. This can happen in essentially two ways: Check to see if the. At points on the graph where you can draw many tangents, the derivative is not defined, and you.
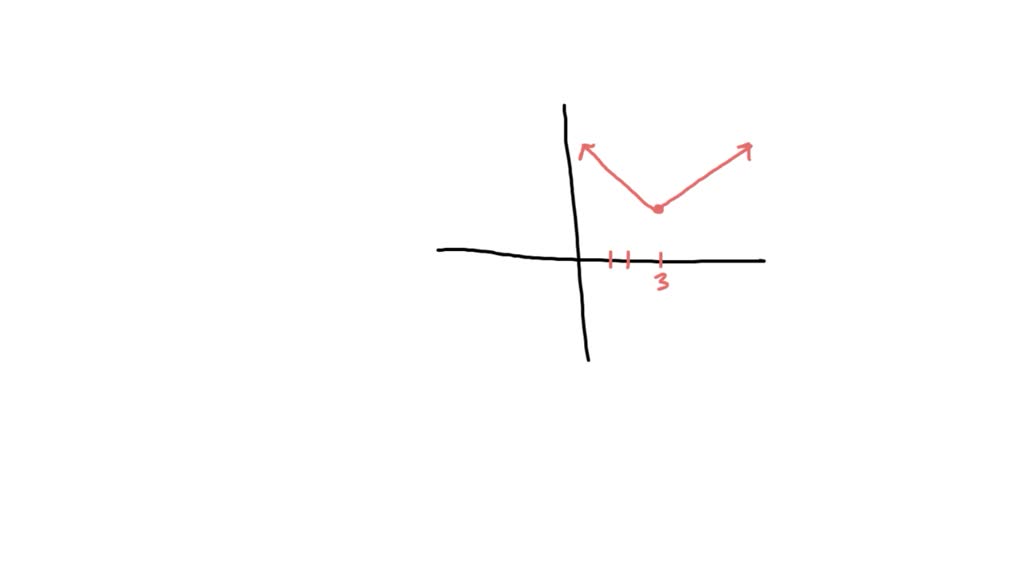
Draw a graph that is continuous, but not differentiable, at x=3. Numerade
How to check for when a function is not differentiable step 1: But the function f in figure 1.7.6 is not differentiable at a = 1 because f′(1) fails to. We'll look at all 3 cases. 1) the tangent line is vertical (and that. At points on the graph where you can draw many tangents, the derivative is not defined,.
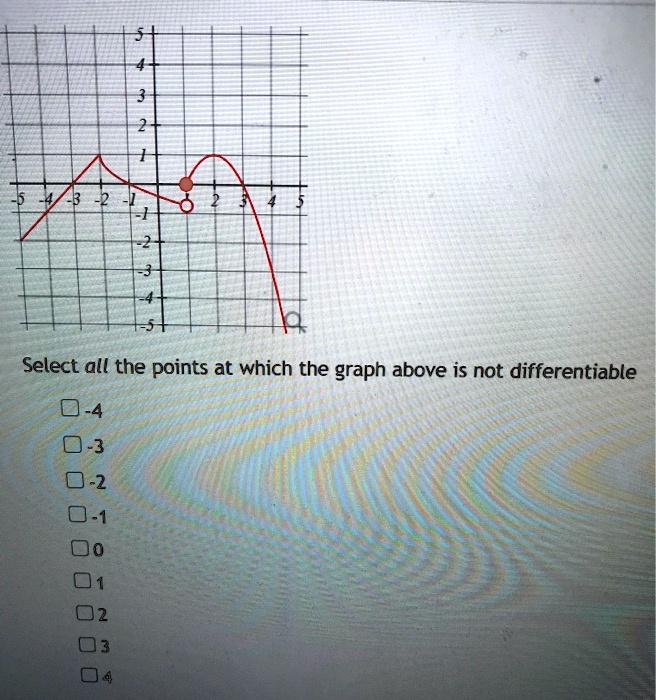
Solved Select all the points at which the graph above is not
But the function f in figure 1.7.6 is not differentiable at a = 1 because f′(1) fails to. We'll look at all 3 cases. This can happen in essentially two ways: How to check for when a function is not differentiable step 1: 1) the tangent line is vertical (and that.
SOLVED Draw a graph that is continuous, but not differentiable, at x=3.
This can happen in essentially two ways: But the function f in figure 1.7.6 is not differentiable at a = 1 because f′(1) fails to. At points on the graph where you can draw many tangents, the derivative is not defined, and you. 1) the tangent line is vertical (and that. We'll look at all 3 cases.
[Solved] Please help. Where is the graph below not differentiable and
1) the tangent line is vertical (and that. We'll look at all 3 cases. This can happen in essentially two ways: Check to see if the. How to check for when a function is not differentiable step 1:
Draw a graph that is continuous, but not differentiable, at Quizlet
How to check for when a function is not differentiable step 1: 1) the tangent line is vertical (and that. Check to see if the. At points on the graph where you can draw many tangents, the derivative is not defined, and you. We'll look at all 3 cases.
SOLVED The figure shows the graph of a function At the given value of
How to check for when a function is not differentiable step 1: This can happen in essentially two ways: At points on the graph where you can draw many tangents, the derivative is not defined, and you. We'll look at all 3 cases. 1) the tangent line is vertical (and that.
select all the points at which the graph above is not differentiable 0
We'll look at all 3 cases. At points on the graph where you can draw many tangents, the derivative is not defined, and you. This can happen in essentially two ways: How to check for when a function is not differentiable step 1: But the function f in figure 1.7.6 is not differentiable at a = 1 because f′(1) fails.
[Solved] Please help. Where is the graph below not differentiable and
Check to see if the. But the function f in figure 1.7.6 is not differentiable at a = 1 because f′(1) fails to. We'll look at all 3 cases. How to check for when a function is not differentiable step 1: This can happen in essentially two ways:
Check To See If The.
How to check for when a function is not differentiable step 1: We'll look at all 3 cases. But the function f in figure 1.7.6 is not differentiable at a = 1 because f′(1) fails to. This can happen in essentially two ways:
At Points On The Graph Where You Can Draw Many Tangents, The Derivative Is Not Defined, And You.
1) the tangent line is vertical (and that.