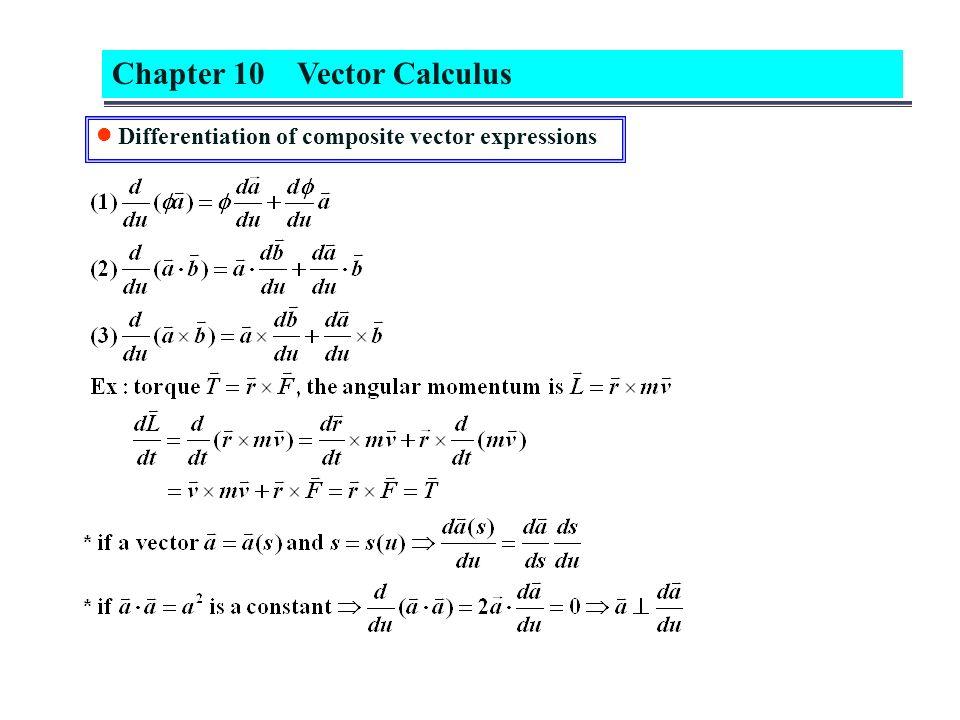
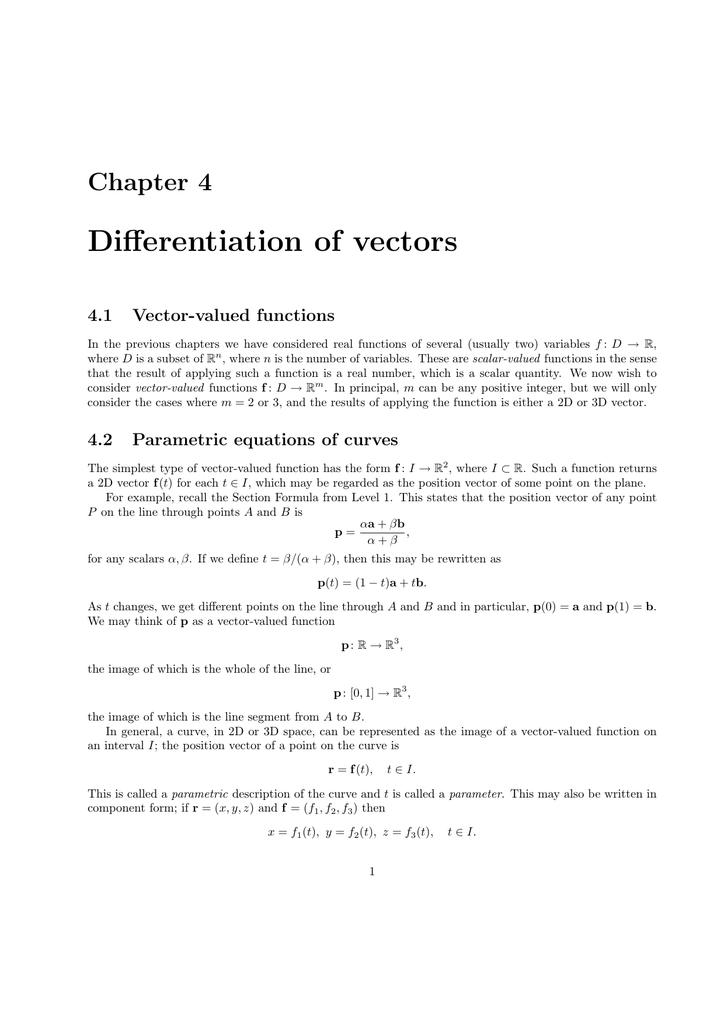
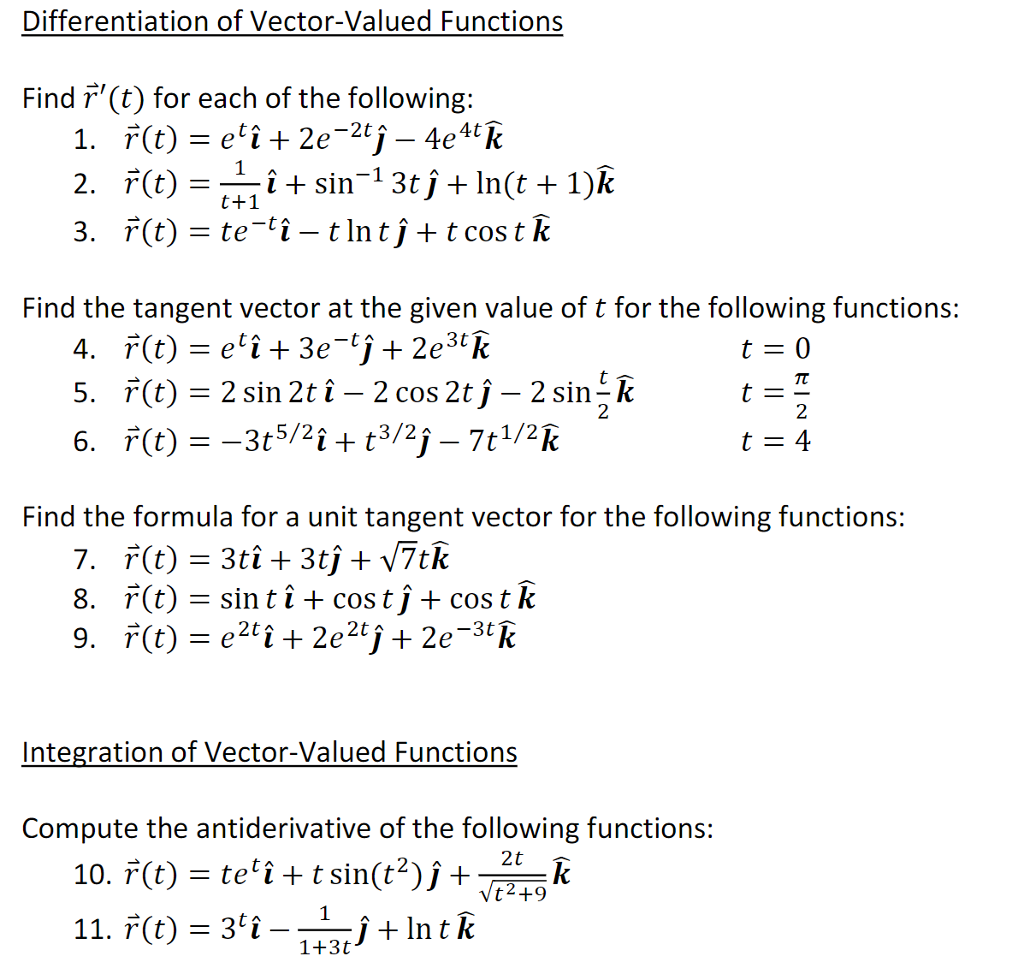
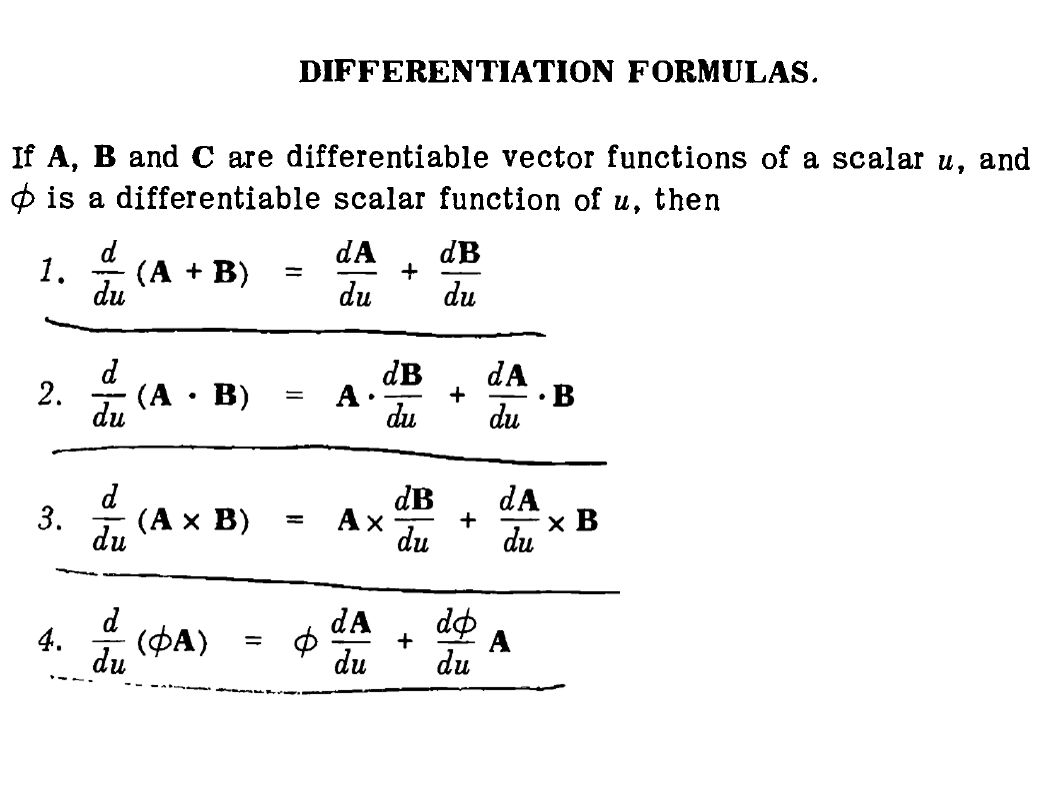
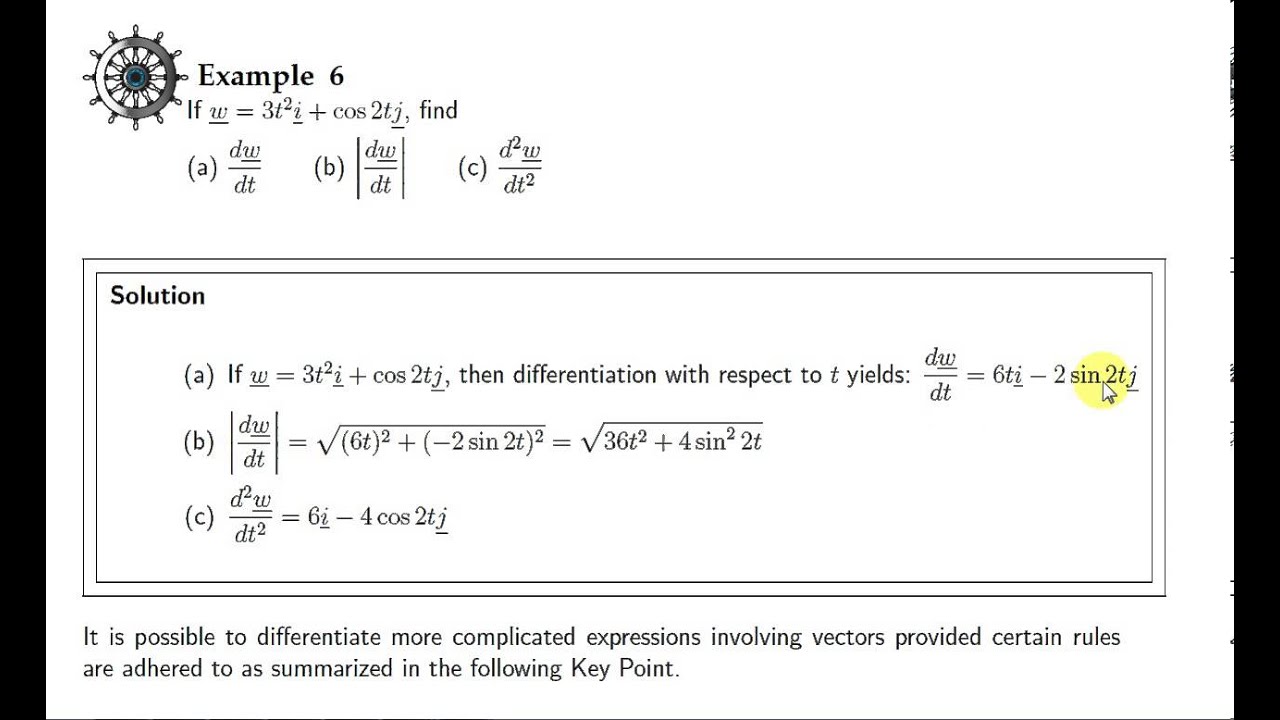
Golden Rule Of Vector Differentiation - For example, in f(t) = t2 + 2t, the input is t, whereas the o. Recall that a function f takes an input, and yields an output. We will consider two types of line integrals: As we will see, once we have. Integrals of scalar functions and integrals of vector functions.
We will consider two types of line integrals: As we will see, once we have. Integrals of scalar functions and integrals of vector functions. Recall that a function f takes an input, and yields an output. For example, in f(t) = t2 + 2t, the input is t, whereas the o.
Recall that a function f takes an input, and yields an output. For example, in f(t) = t2 + 2t, the input is t, whereas the o. We will consider two types of line integrals: As we will see, once we have. Integrals of scalar functions and integrals of vector functions.
Vector Differentiation at Collection of Vector
We will consider two types of line integrals: Integrals of scalar functions and integrals of vector functions. As we will see, once we have. For example, in f(t) = t2 + 2t, the input is t, whereas the o. Recall that a function f takes an input, and yields an output.
Vector Differentiation at Collection of Vector
For example, in f(t) = t2 + 2t, the input is t, whereas the o. As we will see, once we have. Integrals of scalar functions and integrals of vector functions. We will consider two types of line integrals: Recall that a function f takes an input, and yields an output.
Vector Differentiation at Collection of Vector
For example, in f(t) = t2 + 2t, the input is t, whereas the o. As we will see, once we have. We will consider two types of line integrals: Recall that a function f takes an input, and yields an output. Integrals of scalar functions and integrals of vector functions.
Vector Differentiation at Collection of Vector
As we will see, once we have. Integrals of scalar functions and integrals of vector functions. For example, in f(t) = t2 + 2t, the input is t, whereas the o. We will consider two types of line integrals: Recall that a function f takes an input, and yields an output.
Vector Differentiation at Collection of Vector
As we will see, once we have. We will consider two types of line integrals: Recall that a function f takes an input, and yields an output. Integrals of scalar functions and integrals of vector functions. For example, in f(t) = t2 + 2t, the input is t, whereas the o.
Vector Differentiation at Collection of Vector
For example, in f(t) = t2 + 2t, the input is t, whereas the o. Integrals of scalar functions and integrals of vector functions. Recall that a function f takes an input, and yields an output. We will consider two types of line integrals: As we will see, once we have.
Vector Differentiation at Collection of Vector
We will consider two types of line integrals: For example, in f(t) = t2 + 2t, the input is t, whereas the o. Recall that a function f takes an input, and yields an output. Integrals of scalar functions and integrals of vector functions. As we will see, once we have.
Golden rule PDF
As we will see, once we have. We will consider two types of line integrals: Recall that a function f takes an input, and yields an output. For example, in f(t) = t2 + 2t, the input is t, whereas the o. Integrals of scalar functions and integrals of vector functions.
Vector Differentiation at Collection of Vector
We will consider two types of line integrals: For example, in f(t) = t2 + 2t, the input is t, whereas the o. As we will see, once we have. Recall that a function f takes an input, and yields an output. Integrals of scalar functions and integrals of vector functions.
Vector Differentiation at Collection of Vector
As we will see, once we have. Recall that a function f takes an input, and yields an output. Integrals of scalar functions and integrals of vector functions. For example, in f(t) = t2 + 2t, the input is t, whereas the o. We will consider two types of line integrals:
For Example, In F(T) = T2 + 2T, The Input Is T, Whereas The O.
Recall that a function f takes an input, and yields an output. As we will see, once we have. We will consider two types of line integrals: Integrals of scalar functions and integrals of vector functions.