Solving Nonlinear Differential Equations - We explain the distinction between linear and. The logistic equation introduces the first example of a nonlinear differential equation. This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. Root nding in one dimension: The revised methods for solving nonlinear second order differential equations are obtained by combining the basic ideas of nonlinear second. F(x) = 0 with x 2[a;b] or more generally, solving. Basics of nonlinear solvers fundamentals simplest problem:
The revised methods for solving nonlinear second order differential equations are obtained by combining the basic ideas of nonlinear second. This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. Root nding in one dimension: The logistic equation introduces the first example of a nonlinear differential equation. We explain the distinction between linear and. F(x) = 0 with x 2[a;b] or more generally, solving. Basics of nonlinear solvers fundamentals simplest problem:
This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. F(x) = 0 with x 2[a;b] or more generally, solving. Root nding in one dimension: Basics of nonlinear solvers fundamentals simplest problem: The logistic equation introduces the first example of a nonlinear differential equation. The revised methods for solving nonlinear second order differential equations are obtained by combining the basic ideas of nonlinear second. We explain the distinction between linear and.
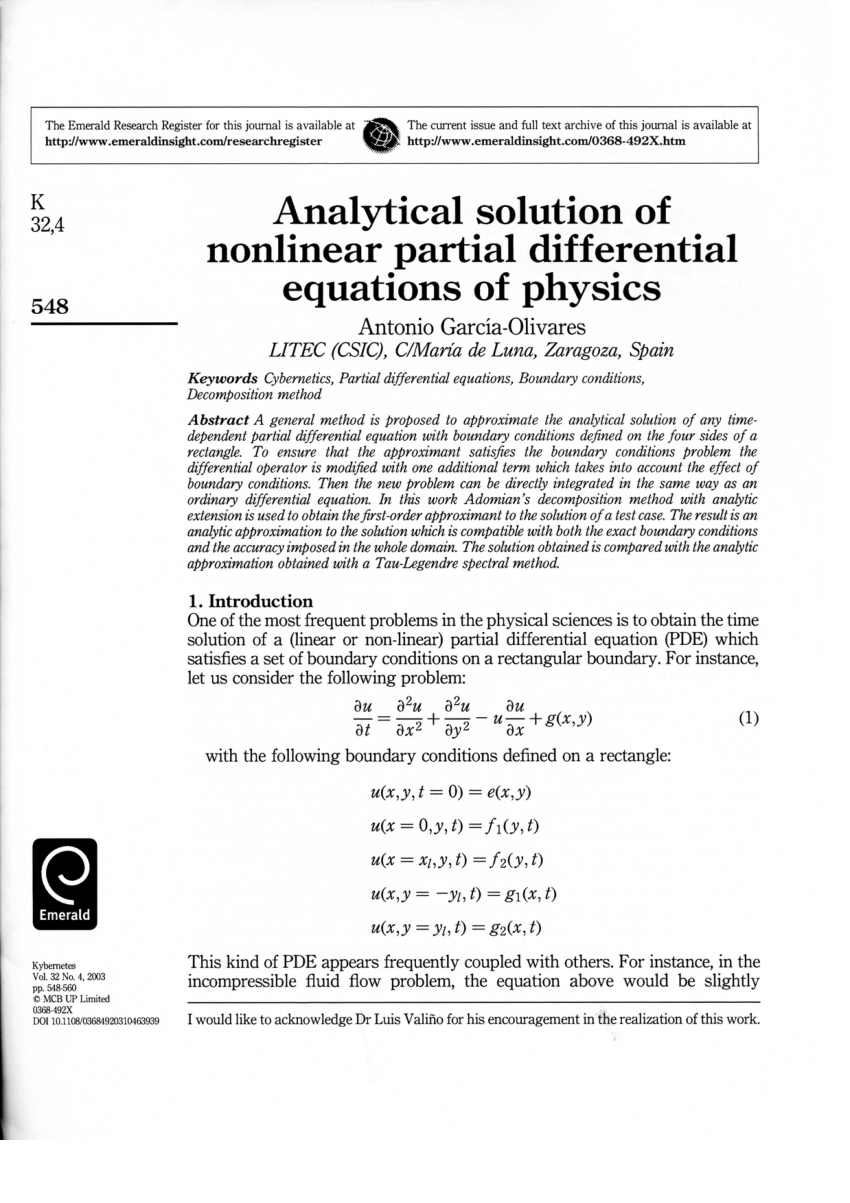
(PDF) Analytical solution of partial differential equations
This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. The revised methods for solving nonlinear second order differential equations are obtained by combining the basic ideas of nonlinear second. Basics of nonlinear solvers fundamentals simplest problem: F(x) = 0 with x 2[a;b] or more generally, solving. We explain.
Differential Equations and Dynamical Systems MDPI Books
We explain the distinction between linear and. This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. F(x) = 0 with x 2[a;b] or more generally, solving. The logistic equation introduces the first example of a nonlinear differential equation. Root nding in one dimension:
math Solving differential first order equations using
Basics of nonlinear solvers fundamentals simplest problem: Root nding in one dimension: The logistic equation introduces the first example of a nonlinear differential equation. F(x) = 0 with x 2[a;b] or more generally, solving. We explain the distinction between linear and.
SOLUTION linear and non linear differential equation examples
The logistic equation introduces the first example of a nonlinear differential equation. The revised methods for solving nonlinear second order differential equations are obtained by combining the basic ideas of nonlinear second. This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. We explain the distinction between linear and..
"Analytical techniques for solving partial differential
We explain the distinction between linear and. The revised methods for solving nonlinear second order differential equations are obtained by combining the basic ideas of nonlinear second. This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. Root nding in one dimension: Basics of nonlinear solvers fundamentals simplest problem:
(PDF) Solving differential equations with differentiable
This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. The logistic equation introduces the first example of a nonlinear differential equation. The revised methods for solving nonlinear second order differential equations are obtained by combining the basic ideas of nonlinear second. Root nding in one dimension: We explain.
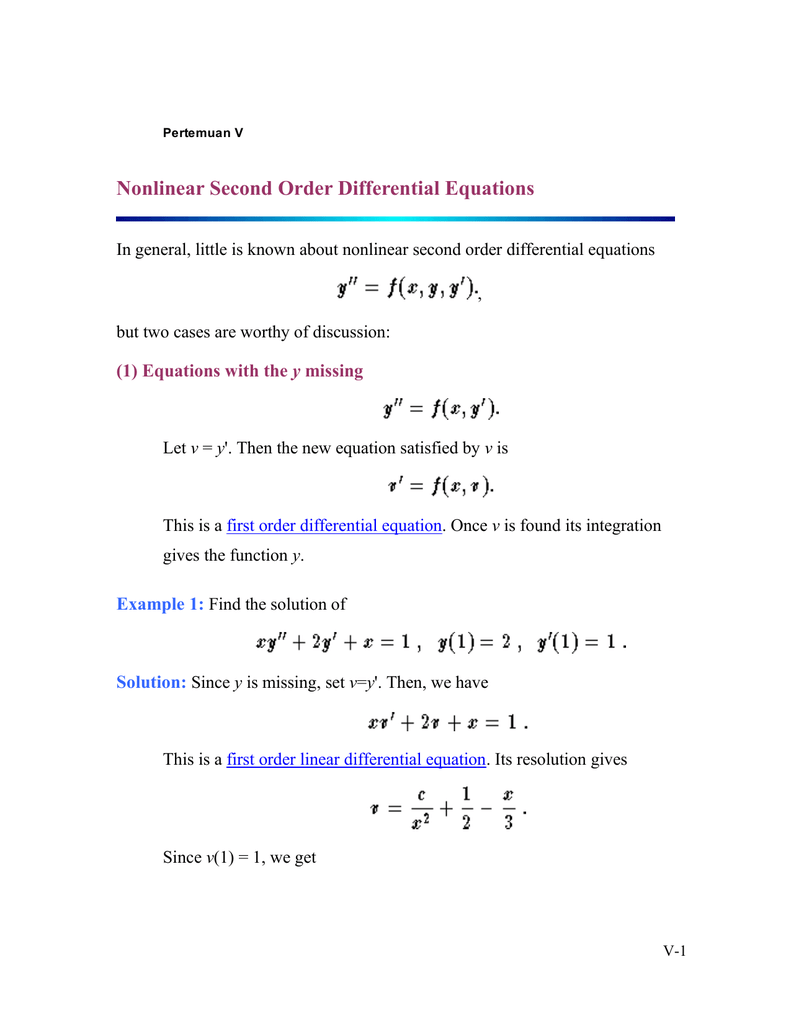
Second Order Differential Equations
Root nding in one dimension: Basics of nonlinear solvers fundamentals simplest problem: We explain the distinction between linear and. The revised methods for solving nonlinear second order differential equations are obtained by combining the basic ideas of nonlinear second. F(x) = 0 with x 2[a;b] or more generally, solving.
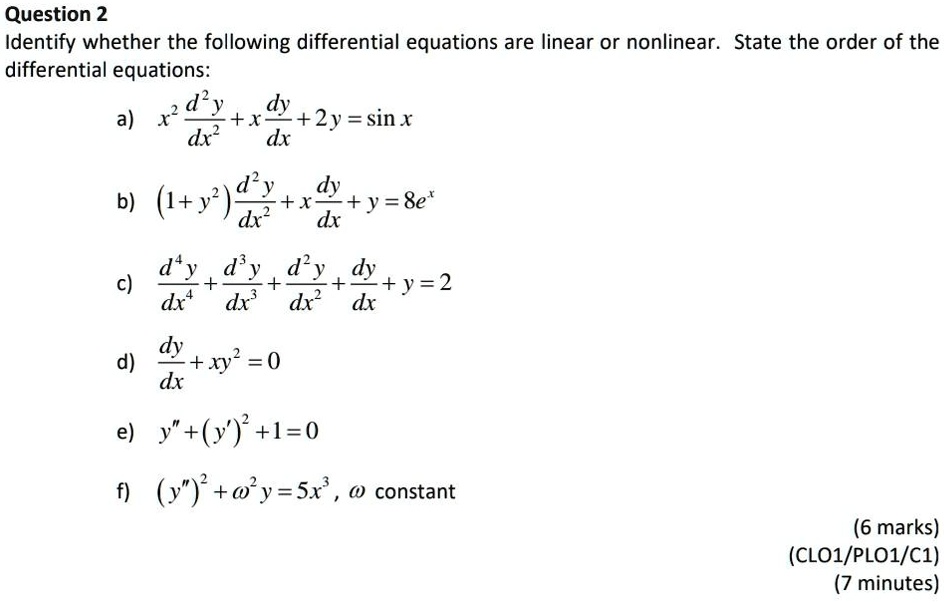
SOLVEDQuestion 2 Identify whether the following differential equations
This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. Root nding in one dimension: We explain the distinction between linear and. The logistic equation introduces the first example of a nonlinear differential equation. F(x) = 0 with x 2[a;b] or more generally, solving.
A neural network approach for solving differential equations
This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. The revised methods for solving nonlinear second order differential equations are obtained by combining the basic ideas of nonlinear second. The logistic equation introduces the first example of a nonlinear differential equation. We explain the distinction between linear and..
problem solving Solve first order differential equations
Basics of nonlinear solvers fundamentals simplest problem: This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. The revised methods for solving nonlinear second order differential equations are obtained by combining the basic ideas of nonlinear second. F(x) = 0 with x 2[a;b] or more generally, solving. Root nding.
We Explain The Distinction Between Linear And.
The logistic equation introduces the first example of a nonlinear differential equation. This paper discusses the basic techniques of solving linear ordinary di erential equations, as well as some tricks for solving nonlinear. Basics of nonlinear solvers fundamentals simplest problem: Root nding in one dimension:
The Revised Methods For Solving Nonlinear Second Order Differential Equations Are Obtained By Combining The Basic Ideas Of Nonlinear Second.
F(x) = 0 with x 2[a;b] or more generally, solving.