Homogeneous Vs Inhomogeneous Differential Equation - Homogeneity of a linear de. Thus, these differential equations are. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator.
The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Thus, these differential equations are. Homogeneity of a linear de. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g.
If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. Thus, these differential equations are. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Homogeneity of a linear de.
02 Tugas Kelompok Homogeneous Vs Inhomogeneous PDF
(1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. The simplest way to test whether an equation (here the equation for the.
Must Do Problems Of Homogeneous Differential Equation Livedu
If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Thus, these differential equations are. Homogeneity of a linear de. The simplest way to test whether an equation (here the equation.
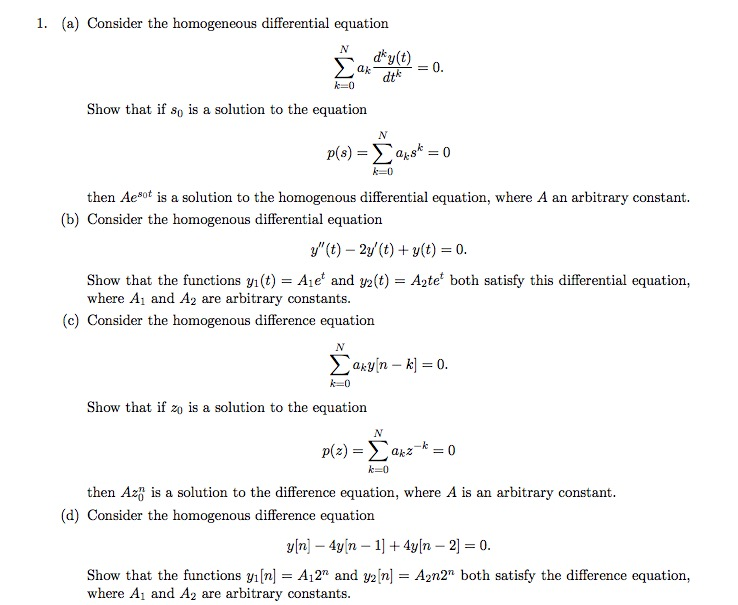
Solved Consider the homogeneous differential equation Show
If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator..
Ex 9.5, 17 Which is a homogeneous differential equation
Homogeneity of a linear de. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. Thus, these differential equations are. (1) and (2) are of the form $$ \mathcal{d} u.
Solved 4. (5 pts) Classify these equations as being
Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. The simplest way to test whether an equation (here the equation for the.
Homogeneous Differential Equation Know types, Steps to solve
Homogeneity of a linear de. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. Thus, these differential equations are. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. If all the terms of the equation contain the.
Inhomogeneous Linear Differential Equations KZHU.ai 🚀
(1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Homogeneity of a linear de. Thus, these differential equations are. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. Where f i(x) f i (x) and g(x) g.
SOLVED Question 1 Consider the inhomogeneous differential equation 5
Homogeneity of a linear de. The simplest way to test whether an equation (here the equation for the boundary conditions) is homogeneous is to substitute the. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal.
Homogeneous Differential Equations Docsity
(1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. Thus, these differential equations are. Homogeneity of a linear de. The simplest way.
SOLUTION Chapter 4 homogeneous differential equation Studypool
If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. (1) and (2) are of the form $$ \mathcal{d} u = 0 $$ where $\mathcal d$ is a differential operator. Thus, these differential equations are. Homogeneity of a linear de. The simplest way to test whether an equation (here the equation.
The Simplest Way To Test Whether An Equation (Here The Equation For The Boundary Conditions) Is Homogeneous Is To Substitute The.
Where f i(x) f i (x) and g(x) g (x) are functions of x, x, the differential equation is said to be homogeneous if g(x)= 0 g. Thus, these differential equations are. If all the terms of the equation contain the unknown function or its derivative then the equation is homogeneous;. Homogeneity of a linear de.